Advertisements
Advertisements
प्रश्न
A body is performing S.H.M. Then its ______.
- average total energy per cycle is equal to its maximum kinetic energy.
- average kinetic energy per cycle is equal to half of its maximum kinetic energy.
- mean velocity over a complete cycle is equal to `2/π` times of its π maximum velocity.
- root mean square velocity is times of its maximum velocity `1/sqrt(2)`.
उत्तर
a, b and d
Explanation:
In the case of S.H.M, the average total energy per cycle
= Maximum kinetic energy (K0)
= Maximum potential energy (U0)
Average KE per cycle = `(0 + K_0)/2 = K_0/2`
Let us write the equation for the SHM x = a sin ωt.
Clearly, it is a periodic motion as it involves a sine function.
Let us find velocity of the particle, `v = (dx)/(dt) = d/(dt) (a sin ωt) = aω cos ωt`
Mean velocity over a complete cycle,
`v_"mean" = (int_0^(2pi) ωa cos θd θ)/(2pi)`
= `(ωa[sin θ]_0^(2pi))/(2pi)`
= 0
So, `v_"mean" ≠ 2/pi v_"max"`
Root mean square speed,
`v_(rms) = sqrt((v_"min"^2 + v_"max"^2)/2`
= `sqrt((0 + v_"max"^2)/2`
`v_(rms) = 1/sqrt(2) v_"max"`
APPEARS IN
संबंधित प्रश्न
A particle executes simple harmonic motion with an amplitude of 10 cm. At what distance from the mean position are the kinetic and potential energies equal?
The equation of motion of a particle started at t = 0 is given by x = 5 sin (20t + π/3), where x is in centimetre and t in second. When does the particle
(a) first come to rest
(b) first have zero acceleration
(c) first have maximum speed?
Consider a particle moving in simple harmonic motion according to the equation x = 2.0 cos (50 πt + tan−1 0.75) where x is in centimetre and t in second. The motion is started at t = 0. (a) When does the particle come to rest for the first time? (b) When does he acceleration have its maximum magnitude for the first time? (c) When does the particle come to rest for the second time ?
A block suspended from a vertical spring is in equilibrium. Show that the extension of the spring equals the length of an equivalent simple pendulum, i.e., a pendulum having frequency same as that of the block.
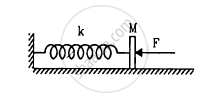
In following figure k = 100 N/m M = 1 kg and F = 10 N.
- Find the compression of the spring in the equilibrium position.
- A sharp blow by some external agent imparts a speed of 2 m/s to the block towards left. Find the sum of the potential energy of the spring and the kinetic energy of the block at this instant.
- Find the time period of the resulting simple harmonic motion.
- Find the amplitude.
- Write the potential energy of the spring when the block is at the left extreme.
- Write the potential energy of the spring when the block is at the right extreme.
The answer of b, e and f are different. Explain why this does not violate the principle of conservation of energy.
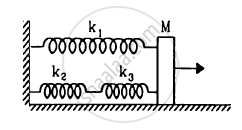
Repeat the previous exercise if the angle between each pair of springs is 120° initially.
The springs shown in the figure are all unstretched in the beginning when a man starts pulling the block. The man exerts a constant force F on the block. Find the amplitude and the frequency of the motion of the block.
When a particle executing S.H.M oscillates with a frequency v, then the kinetic energy of the particle?
When the displacement of a particle executing simple harmonic motion is half its amplitude, the ratio of its kinetic energy to potential energy is ______.
A body is executing simple harmonic motion with frequency ‘n’, the frequency of its potential energy is ______.
