Advertisements
Advertisements
प्रश्न
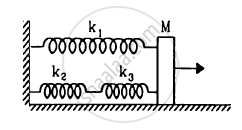
The springs shown in the figure are all unstretched in the beginning when a man starts pulling the block. The man exerts a constant force F on the block. Find the amplitude and the frequency of the motion of the block.
उत्तर
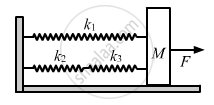
As the block of mass M is pulled, a net resultant force is exerted by the three springs opposing the motion of the block.
Now, springs k2 and k3 are in connected as a series combination.
Let k4 be the equivalent spring constant.
\[\therefore \frac{1}{k_4} = \frac{1}{k_2} + \frac{1}{k_3} = \frac{k_2 + k_3}{k_2 k_3}\]
\[ k_4 = \frac{k_2 k_3}{k_2 + k_3}\]
k4 and k1 form a parallel combination of springs. Hence, equivalent spring constant k = k1 + k4.
\[= \frac{k_2 k_3}{k_2 + k_3} + k_1 \]
\[ = \frac{k_2 k_3 + k_1 k_2 + k_1 k_3}{k_2 + k_3}\]
\[ \therefore \text { Time peiod }, T = 2\pi\sqrt{\frac{M}{k}}\]
\[ = 2\pi\sqrt{\frac{M \left( k_2 + k_3 \right)}{k_2 k_3 + k_1 k_2 + k_1 k_3}}\]
(b) Frequency \[\left( v \right)\] is given by,
\[v = \frac{1}{T}\]
\[= \frac{1}{2\pi}\sqrt{\frac{k_2 k_3 + k_1 k_2 + k_1 k_3}{M\left( k_2 + k_3 \right)}}\]
(c) Amplitude ( x ) is given by,
\[x = \frac{F}{k} = \frac{F\left( k_2 + k_3 \right)}{k_1 k_2 + k_2 k_3 + k_1 k_3}\]
APPEARS IN
संबंधित प्रश्न
A particle executes simple harmonic motion with an amplitude of 10 cm. At what distance from the mean position are the kinetic and potential energies equal?
A particle having mass 10 g oscillates according to the equation x = (2.0 cm) sin [(100 s−1)t + π/6]. Find (a) the amplitude, the time period and the spring constant. (c) the position, the velocity and the acceleration at t = 0.
The equation of motion of a particle started at t = 0 is given by x = 5 sin (20t + π/3), where x is in centimetre and t in second. When does the particle
(a) first come to rest
(b) first have zero acceleration
(c) first have maximum speed?
Consider a particle moving in simple harmonic motion according to the equation x = 2.0 cos (50 πt + tan−1 0.75) where x is in centimetre and t in second. The motion is started at t = 0. (a) When does the particle come to rest for the first time? (b) When does he acceleration have its maximum magnitude for the first time? (c) When does the particle come to rest for the second time ?
A block suspended from a vertical spring is in equilibrium. Show that the extension of the spring equals the length of an equivalent simple pendulum, i.e., a pendulum having frequency same as that of the block.
A block of mass 0.5 kg hanging from a vertical spring executes simple harmonic motion of amplitude 0.1 m and time period 0.314 s. Find the maximum force exerted by the spring on the block.
The spring shown in figure is unstretched when a man starts pulling on the cord. The mass of the block is M. If the man exerts a constant force F, find (a) the amplitude and the time period of the motion of the block, (b) the energy stored in the spring when the block passes through the equilibrium position and (c) the kinetic energy of the block at this position.
Solve the previous problem if the pulley has a moment of inertia I about its axis and the string does not slip over it.
Discuss in detail the energy in simple harmonic motion.
A body is executing simple harmonic motion with frequency ‘n’, the frequency of its potential energy is ______.
Motion of an oscillating liquid column in a U-tube is ______.
Draw a graph to show the variation of P.E., K.E. and total energy of a simple harmonic oscillator with displacement.
A mass of 2 kg is attached to the spring of spring constant 50 Nm–1. The block is pulled to a distance of 5 cm from its equilibrium position at x = 0 on a horizontal frictionless surface from rest at t = 0. Write the expression for its displacement at anytime t.
A body of mass m is attached to one end of a massless spring which is suspended vertically from a fixed point. The mass is held in hand so that the spring is neither stretched nor compressed. Suddenly the support of the hand is removed. The lowest position attained by the mass during oscillation is 4 cm below the point, where it was held in hand.
What is the amplitude of oscillation?
An object of mass 0.5 kg is executing a simple Harmonic motion. Its amplitude is 5 cm and the time period (T) is 0.2 s. What will be the potential energy of the object at an instant t = `T/4` s starting from the mean position? Assume that the initial phase of the oscillation is zero.
