Advertisements
Advertisements
प्रश्न
A body of mass m is attached to one end of a massless spring which is suspended vertically from a fixed point. The mass is held in hand so that the spring is neither stretched nor compressed. Suddenly the support of the hand is removed. The lowest position attained by the mass during oscillation is 4 cm below the point, where it was held in hand.
What is the amplitude of oscillation?
उत्तर
When the support of the hand is removed, the body oscillates about a mean position.

Suppose x is the maximum extension in the spring when it reaches the lowest point in oscillation.
Loss in PE of the block = `mgx` ......(i)
Where m = mass of the block
The gain in elastic potential energy of the spring = `1/2 kx^2` ......(ii)
As the two are equal, conserving mechanical energy,
We get, `mgx = 1/2kx^2` or `x = (2mg)/k` ......(iii)
Now, the mean position of oscillation will be, when the block is balanced by the spring.
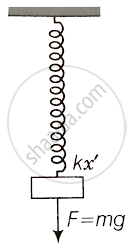
If x' is the extension in that case, then F = + kx'
But `F = mg`
⇒ `mg = + kx^'`
or `x^' = (mg)/k` ......(iv)
Dividing equation (iii) by equation (iv),
`x/x^' = ((2mg)/k)/((mg)/k)` = 2
⇒ `x = 2x^'`
But given x = 4 cm ......(maximum extension from the unstretched position)
∴ `2x^'` = 4
∴ `x^' = 4/2` = 2 cm
But the displacement of mass from the mean position to the position when the spring attains its natural length is equal to the amplitude of the oscillation.
∴ A = x' = 2 cm
Where A = amplitude of the motion.
APPEARS IN
संबंधित प्रश्न
A particle executes simple harmonic motion with an amplitude of 10 cm. At what distance from the mean position are the kinetic and potential energies equal?
The maximum speed and acceleration of a particle executing simple harmonic motion are 10 cm/s and 50 cm/s2. Find the position(s) of the particle when the speed is 8 cm/s.
A particle having mass 10 g oscillates according to the equation x = (2.0 cm) sin [(100 s−1)t + π/6]. Find (a) the amplitude, the time period and the spring constant. (c) the position, the velocity and the acceleration at t = 0.
Consider a particle moving in simple harmonic motion according to the equation x = 2.0 cos (50 πt + tan−1 0.75) where x is in centimetre and t in second. The motion is started at t = 0. (a) When does the particle come to rest for the first time? (b) When does he acceleration have its maximum magnitude for the first time? (c) When does the particle come to rest for the second time ?
A body of mass 2 kg suspended through a vertical spring executes simple harmonic motion of period 4 s. If the oscillations are stopped and the body hangs in equilibrium find the potential energy stored in the spring.
A rectangle plate of sides a and b is suspended from a ceiling by two parallel string of length L each in Figure . The separation between the string is d. The plate is displaced slightly in its plane keeping the strings tight. Show that it will execute simple harmonic motion. Find the time period.

Discuss in detail the energy in simple harmonic motion.
If a body is executing simple harmonic motion and its current displacements is `sqrt3/2` times the amplitude from its mean position, then the ratio between potential energy and kinetic energy is:
Find the displacement of a simple harmonic oscillator at which its P.E. is half of the maximum energy of the oscillator.
A mass of 2 kg is attached to the spring of spring constant 50 Nm–1. The block is pulled to a distance of 5 cm from its equilibrium position at x = 0 on a horizontal frictionless surface from rest at t = 0. Write the expression for its displacement at anytime t.
