Advertisements
Chapters
2: Units and Measurements
3: Motion In a Straight Line
4: Motion In a Plane
5: Laws of Motion
6: Work, Energy and Power
7: System of Particles and Rotational Motion
8: Gravitation
9: Mechanical Properties of Solids
10: Mechanical Properties of Fluids
11: Thermal Properties of Matter
12: Thermodynamics
13: Kinetic Theory
▶ 14: Oscillations
15: Waves
![NCERT Exemplar solutions for Physics [English] Class 11 chapter 14 - Oscillations NCERT Exemplar solutions for Physics [English] Class 11 chapter 14 - Oscillations - Shaalaa.com](/images/physics-english-class-11_6:5f2b1b2038084cf381bfa42c826a928c.jpg)
Advertisements
Solutions for Chapter 14: Oscillations
Below listed, you can find solutions for Chapter 14 of CBSE NCERT Exemplar for Physics [English] Class 11.
NCERT Exemplar solutions for Physics [English] Class 11 14 Oscillations Exercises [Pages 97 - 104]
MCQ I
The displacement of a particle is represented by the equation `y = 3 cos (pi/4 - 2ωt)`. The motion of the particle is ______.
simple harmonic with period 2p/w.
simple harmonic with period π/ω.
periodic but not simple harmonic.
non-periodic.
The displacement of a particle is represented by the equation y = sin3ωt. The motion is ______.
non-periodic.
periodic but not simple harmonic.
simple harmonic with period 2π/ω.
simple harmonic with period π/ω.
The relation between acceleration and displacement of four particles are given below: Which one of the particles is executing simple harmonic motion?
ax = + 2x.
ax = + 2x2.
ax = – 2x2.
ax = – 2x.
Motion of an oscillating liquid column in a U-tube is ______.
periodic but not simple harmonic.
non-periodic.
simple harmonic and time period is independent of the density of the liquid.
simple harmonic and time-period is directly proportional to the density of the liquid.
A particle is acted simultaneously by mutually perpendicular simple harmonic motions x = a cos ωt and y = a sin ωt. The trajectory of motion of the particle will be ______.
an ellipse.
a parabola.
a circle.
a straight line.
The displacement of a particle varies with time according to the relation y = a sin ωt + b cos ωt.
The motion is oscillatory but not S.H.M.
The motion is S.H.M. with amplitude a + b.
The motion is S.H.M. with amplitude a2 + b2.
The motion is S.H.M. with amplitude `sqrt(a^2 + b^2)`.
Four pendulums A, B, C and D are suspended from the same elastic support as shown in figure. A and C are of the same length, while B is smaller than A and D is larger than A. If A is given a transverse displacement ______.
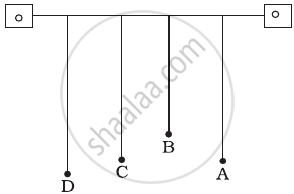
D will vibrate with maximum amplitude.
C will vibrate with maximum amplitude.
B will vibrate with maximum amplitude.
All the four will oscillate with equal amplitude.
Figure shows the circular motion of a particle. The radius of the circle, the period, sense of revolution and the initial position are indicated on the figure. The simple harmonic motion of the x-projection of the radius vector of the rotating particle P is ______.
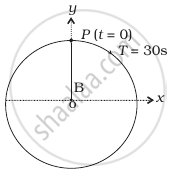
`x(t) = B sin ((2pit)/30)`.
`x(t) = B cos ((pit)/15)`.
`x(t) = B sin ((pit)/15 + pi/2)`.
`x(t) = B cos ((pit)/15 + pi/2)`.
The equation of motion of a particle is x = a cos (αt)2. The motion is ______.
periodic but not oscillatory.
periodic and oscillatory.
oscillatory but not periodic.
neither periodic nor oscillatory.
A particle executing S.H.M. has a maximum speed of 30 cm/s and a maximum acceleration of 60 cm/s2. The period of oscillation is ______.
πs.
`π/2` s.
2π s.
`π/t` s.
When a mass m is connected individually to two springs S1 and S2, the oscillation frequencies are ν1 and ν2. If the same mass is attached to the two springs as shown in figure, the oscillation frequency would be ______.
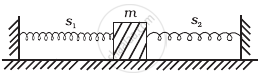
`v_1 + v_2`.
`sqrt(v_1^2 + v_2^2)`.
`(1/v_1 + 1/v_2)^-1`.
`sqrt(v_1^2 - v_2^2)`.
MCQ II
The rotation of earth about its axis is ______.
- periodic motion.
- simple harmonic motion.
- periodic but not simple harmonic motion.
- non-periodic motion.
Motion of a ball bearing inside a smooth curved bowl, when released from a point slightly above the lower point is ______.
- simple harmonic motion.
- non-periodic motion.
- periodic motion.
- periodic but not S.H.M.
Displacement vs. time curve for a particle executing S.H.M. is shown in figure. Choose the correct statements.
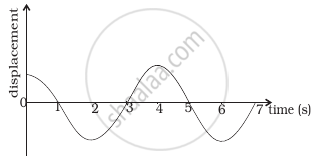
- Phase of the oscillator is same at t = 0 s and t = 2s.
- Phase of the oscillator is same at t = 2 s and t = 6s.
- Phase of the oscillator is same at t = 1 s and t = 7s.
- Phase of the oscillator is same at t = 1 s and t = 5s.
Which of the following statements is/are true for a simple harmonic oscillator?
- Force acting is directly proportional to displacement from the mean position and opposite to it.
- Motion is periodic.
- Acceleration of the oscillator is constant.
- The velocity is periodic.
The displacement time graph of a particle executing S.H.M. is shown in figure. Which of the following statement is/are true?
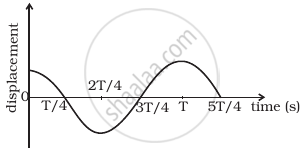
- The force is zero at `t = (T)/4`.
- The acceleration is maximum at `t = (4T)/4`.
- The velocity is maximum at `t = T/4`.
- The P.E. is equal to K.E. of oscillation at `t = T/2`.
A body is performing S.H.M. Then its ______.
- average total energy per cycle is equal to its maximum kinetic energy.
- average kinetic energy per cycle is equal to half of its maximum kinetic energy.
- mean velocity over a complete cycle is equal to `2/π` times of its π maximum velocity.
- root mean square velocity is times of its maximum velocity `1/sqrt(2)`.
A particle is in linear simple harmonic motion between two points A and B, 10 cm apart (Figure). Take the direction from A to B as the + ve direction and choose the correct statements.
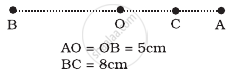
- The sign of velocity, acceleration and force on the particle when it is 3 cm away from A going towards B are positive.
- The sign of velocity of the particle at C going towards O is negative.
- The sign of velocity, acceleration and force on the particle when it is 4 cm away from B going towards A are negative.
- The sign of acceleration and force on the particle when it is at point B is negative.
VSA
Displacement versus time curve for a particle executing S.H.M. is shown in figure. Identify the points marked at which (i) velocity of the oscillator is zero, (ii) speed of the oscillator is maximum.

Two identical springs of spring constant K are attached to a block of mass m and to fixed supports as shown in figure. When the mass is displaced from equilibrium position by a distance x towards right, find the restoring force
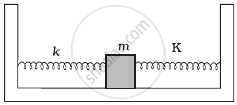
What are the two basic characteristics of a simple harmonic motion?
When will the motion of a simple pendulum be simple harmonic?
What is the ratio of maxmimum acceleration to the maximum velocity of a simple harmonic oscillator?
What is the ratio between the distance travelled by the oscillator in one time period and amplitude?
In figure, what will be the sign of the velocity of the point P′, which is the projection of the velocity of the reference particle P . P is moving in a circle of radius R in anticlockwise direction.
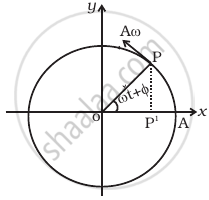
Show that for a particle executing S.H.M, velocity and displacement have a phase difference of π/2.
Draw a graph to show the variation of P.E., K.E. and total energy of a simple harmonic oscillator with displacement.
The length of a second’s pendulum on the surface of earth is 1 m. What will be the length of a second’s pendulum on the moon?
SA
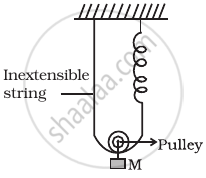
Find the time period of mass M when displaced from its equilibrium position and then released for the system shown in figure.
Show that the motion of a particle represented by y = sin ωt – cos ωt is simple harmonic with a period of 2π/ω.
Find the displacement of a simple harmonic oscillator at which its P.E. is half of the maximum energy of the oscillator.
A body of mass m is situated in a potential field U(x) = U0 (1 – cos αx) when U0 and α are constants. Find the time period of small oscillations.
A mass of 2 kg is attached to the spring of spring constant 50 Nm–1. The block is pulled to a distance of 5 cm from its equilibrium position at x = 0 on a horizontal frictionless surface from rest at t = 0. Write the expression for its displacement at anytime t.
Consider a pair of identical pendulums, which oscillate with equal amplitude independently such that when one pendulum is at its extreme position making an angle of 2° to the right with the vertical, the other pendulum makes an angle of 1° to the left of the vertical. What is the phase difference between the pendulums?
LA
A person normally weighing 50 kg stands on a massless platform which oscillates up and down harmonically at a frequency of 2.0 s–1 and an amplitude 5.0 cm. A weighing machine on the platform gives the persons weight against time.
- Will there be any change in weight of the body, during the oscillation?
- If answer to part (a) is yes, what will be the maximum and minimum reading in the machine and at which position?
A body of mass m is attached to one end of a massless spring which is suspended vertically from a fixed point. The mass is held in hand so that the spring is neither stretched nor compressed. Suddenly the support of the hand is removed. The lowest position attained by the mass during oscillation is 4 cm below the point, where it was held in hand.
What is the amplitude of oscillation?
A body of mass m is attached to one end of a massless spring which is suspended vertically from a fixed point. The mass is held in hand so that the spring is neither stretched nor compressed. Suddenly the support of the hand is removed. The lowest position attained by the mass during oscillation is 4 cm below the point, where it was held in hand.
Find the frequency of oscillation?
A cylindrical log of wood of height h and area of cross-section A floats in water. It is pressed and then released. Show that the log would execute S.H.M. with a time period. `T = 2πsqrt(m/(Apg))` where m is mass of the body and ρ is density of the liquid.
One end of a V-tube containing mercury is connected to a suction pump and the other end to atmosphere. The two arms of the tube are inclined to horizontal at an angle of 45° each. A small pressure difference is created between two columns when the suction pump is removed. Will the column of mercury in V-tube execute simple harmonic motion? Neglect capillary and viscous forces. Find the time period of oscillation.
A tunnel is dug through the centre of the Earth. Show that a body of mass ‘m’ when dropped from rest from one end of the tunnel will execute simple harmonic motion.
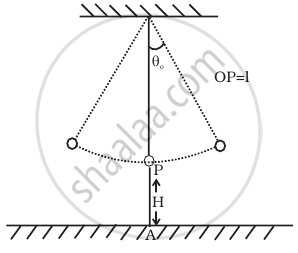
A simple pendulum of time period 1s and length l is hung from a fixed support at O, such that the bob is at a distance H vertically above A on the ground (Figure). The amplitude is θ0. The string snaps at θ = θ0/2. Find the time taken by the bob to hit the ground. Also find distance from A where bob hits the ground. Assume θo to be small so that sin θo = θo and cos θo = 1.
Solutions for 14: Oscillations
![NCERT Exemplar solutions for Physics [English] Class 11 chapter 14 - Oscillations NCERT Exemplar solutions for Physics [English] Class 11 chapter 14 - Oscillations - Shaalaa.com](/images/physics-english-class-11_6:5f2b1b2038084cf381bfa42c826a928c.jpg)
NCERT Exemplar solutions for Physics [English] Class 11 chapter 14 - Oscillations
Shaalaa.com has the CBSE Mathematics Physics [English] Class 11 CBSE solutions in a manner that help students grasp basic concepts better and faster. The detailed, step-by-step solutions will help you understand the concepts better and clarify any confusion. NCERT Exemplar solutions for Mathematics Physics [English] Class 11 CBSE 14 (Oscillations) include all questions with answers and detailed explanations. This will clear students' doubts about questions and improve their application skills while preparing for board exams.
Further, we at Shaalaa.com provide such solutions so students can prepare for written exams. NCERT Exemplar textbook solutions can be a core help for self-study and provide excellent self-help guidance for students.
Concepts covered in Physics [English] Class 11 chapter 14 Oscillations are Force Law for Simple Harmonic Motion, Energy in Simple Harmonic Motion, Some Systems Executing Simple Harmonic Motion, Damped Simple Harmonic Motion, Forced Oscillations and Resonance, Displacement as a Function of Time, Periodic Functions, Oscillations - Frequency, Periodic and Oscillatory Motion, Simple Harmonic Motion (S.H.M.), Simple Harmonic Motion and Uniform Circular Motion, Velocity and Acceleration in Simple Harmonic Motion, Simple Pendulum.
Using NCERT Exemplar Physics [English] Class 11 solutions Oscillations exercise by students is an easy way to prepare for the exams, as they involve solutions arranged chapter-wise and also page-wise. The questions involved in NCERT Exemplar Solutions are essential questions that can be asked in the final exam. Maximum CBSE Physics [English] Class 11 students prefer NCERT Exemplar Textbook Solutions to score more in exams.
Get the free view of Chapter 14, Oscillations Physics [English] Class 11 additional questions for Mathematics Physics [English] Class 11 CBSE, and you can use Shaalaa.com to keep it handy for your exam preparation.
