Advertisements
Advertisements
प्रश्न
Show that the motion of a particle represented by y = sin ωt – cos ωt is simple harmonic with a period of 2π/ω.
उत्तर
The given equation is in the form of a combination of two harmonic functions. We can write this equation in the form of a single harmonic (sine or cosine) function.
We have displacement function: `y = sin ωt - cos ωt`
`y = sqrt(2) (1/sqrt(2) * sin ωt - 1/sqrt(2) * cos ωt)`
= `sqrt(2)[cos (pi/4) * sin ωt - sin(pi/4) * cos ωt]`
= `sqrt(2) [sin (ωt - pi/4) = sqrt(2) [sin (ωt - pi/4)]]`
⇒ `y = sqrt(2) sin (ωt - pi/4)`
Comparing with the standard equation of S.H.M
`y = a sin(ωt + phi)` we get angular frequency of S.H.M, ω = `(2pi)/T` ⇒ `T = (2pi)/ω`
Hence the function represents S.H.M with a period T = 2π/ω.
APPEARS IN
संबंधित प्रश्न
The piston in the cylinder head of a locomotive has a stroke (twice the amplitude) of 1.0 m. If the piston moves with simple harmonic motion with an angular frequency of 200 rad/min, what is its maximum speed?
A particle executes simple harmonic motion under the restoring force provided by a spring. The time period is T. If the spring is divided in two equal parts and one part is used to continue the simple harmonic motion, the time period will
The string the spring and the pulley shown in figure are light. Find the time period of the mass m.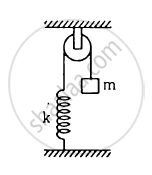
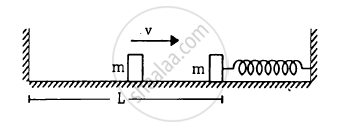
The left block in figure moves at a speed v towards the right block placed in equilibrium. All collisions to take place are elastic and the surfaces are frictionless. Show that the motions of the two blocks are periodic. Find the time period of these periodic motions. Neglect the widths of the blocks.
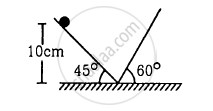
Find the time period of the motion of the particle shown in figure . Neglect the small effect of the bend near the bottom.
A body of mass 1 kg is mafe to oscillate on a spring of force constant 16 N/m. Calculate (a) Angular frequency, (b) Frequency of vibrations.
A simple pendulum is inside a spacecraft. What will be its periodic time?
Which of the following example represent periodic motion?
A freely suspended bar magnet displaced from its N-S direction and released.
Which of the following example represent (nearly) simple harmonic motion and which represent periodic but not simple harmonic motion?
A motion of an oscillating mercury column in a U-tube.
The time period of a simple pendulum is T inside a lift when the lift is stationary. If the lift moves upwards with an acceleration `g/2`, the time period of the pendulum will be ______.
