Advertisements
Advertisements
प्रश्न
Find the elastic potential energy stored in each spring shown in figure, when the block is in equilibrium. Also find the time period of vertical oscillation of the block.
उत्तर
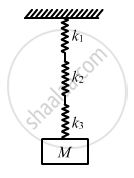
All three spring attached to the mass M are in series.
k1, k2, k3 are the spring constants.
Let k be the resultant spring constant.
\[\frac{1}{k} = \frac{1}{k_1} + \frac{1}{k_2} + \frac{1}{k_3}\]
\[ \Rightarrow k = \frac{k_1 k_2 k_3}{k_1 k_2 + k_2 k_3 + k_3 k_1}\]
\[\text { Time period }\left( T \right)\text{ is given by, }\]
\[T = 2\pi\sqrt{\frac{M}{k}}\]
\[ = 2\sqrt{\frac{M\left( k_1 k_2 + k_2 k_3 + k_3 k_1 \right)}{k_1 k_2 k_3}}\]
\[ = 2\sqrt{M\left( \frac{1}{k_1} + \frac{1}{k_2} + \frac{1}{k_3} \right)}\]
As force is equal to the weight of the body,
F = weight = Mg
Let x1, x2, and x3 be the displacements of the springs having spring constants k1, k2 andk3 respectively.
For spring k1,
\[x_1 = \frac{Mg}{k_1}\]
\[\text { Similarly }, x_2 = \frac{Mg}{k_2}\]
\[\text { and } x_3 = \frac{Mg}{k_3}\]
\[ \therefore {PE}_1 = \frac{1}{2} k_1 x_1^2 \]
\[ = \frac{1}{2} k_1 \left( \frac{Mg}{k_1} \right)^2 \]
\[ = \frac{1}{2} k_1 \frac{M^2 g^2}{k_1^2}\]
\[ = \frac{1}{2}\frac{M^2 g^2}{k_1} = \frac{M^2 g^2}{2 k_1}\]
\[\text { Similarly }, {PE}_2 = \frac{M^2 g^2}{2 k_2}\]
\[\text { and } {PE}_3 = \frac{M^2 g^2}{2 k_3}\]
Notes
The Figure is missing in Question .
APPEARS IN
संबंधित प्रश्न
A particle is in linear simple harmonic motion between two points, A and B, 10 cm apart. Take the direction from A to B as the positive direction and give the signs of velocity, acceleration and force on the particle when it is
(a) at the end A,
(b) at the end B,
(c) at the mid-point of AB going towards A,
(d) at 2 cm away from B going towards A,
(e) at 3 cm away from A going towards B, and
(f) at 4 cm away from B going towards A.
The maximum speed and acceleration of a particle executing simple harmonic motion are 10 cm/s and 50 cm/s2. Find the position(s) of the particle when the speed is 8 cm/s.
The equation of motion of a particle started at t = 0 is given by x = 5 sin (20t + π/3), where x is in centimetre and t in second. When does the particle
(a) first come to rest
(b) first have zero acceleration
(c) first have maximum speed?
Consider a particle moving in simple harmonic motion according to the equation x = 2.0 cos (50 πt + tan−1 0.75) where x is in centimetre and t in second. The motion is started at t = 0. (a) When does the particle come to rest for the first time? (b) When does he acceleration have its maximum magnitude for the first time? (c) When does the particle come to rest for the second time ?
A body of mass 2 kg suspended through a vertical spring executes simple harmonic motion of period 4 s. If the oscillations are stopped and the body hangs in equilibrium find the potential energy stored in the spring.
The block of mass m1 shown in figure is fastened to the spring and the block of mass m2 is placed against it. (a) Find the compression of the spring in the equilibrium position. (b) The blocks are pushed a further distance (2/k) (m1 + m2)g sin θ against the spring and released. Find the position where the two blocks separate. (c) What is the common speed of blocks at the time of separation?
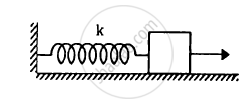
The spring shown in figure is unstretched when a man starts pulling on the cord. The mass of the block is M. If the man exerts a constant force F, find (a) the amplitude and the time period of the motion of the block, (b) the energy stored in the spring when the block passes through the equilibrium position and (c) the kinetic energy of the block at this position.
Repeat the previous exercise if the angle between each pair of springs is 120° initially.
Consider the situation shown in figure . Show that if the blocks are displaced slightly in opposite direction and released, they will execute simple harmonic motion. Calculate the time period.
A rectangle plate of sides a and b is suspended from a ceiling by two parallel string of length L each in Figure . The separation between the string is d. The plate is displaced slightly in its plane keeping the strings tight. Show that it will execute simple harmonic motion. Find the time period.

When the displacement of a particle executing simple harmonic motion is half its amplitude, the ratio of its kinetic energy to potential energy is ______.
If a body is executing simple harmonic motion and its current displacements is `sqrt3/2` times the amplitude from its mean position, then the ratio between potential energy and kinetic energy is:
A body is executing simple harmonic motion with frequency ‘n’, the frequency of its potential energy is ______.
A body is executing simple harmonic motion with frequency ‘n’, the frequency of its potential energy is ______.
Motion of an oscillating liquid column in a U-tube is ______.
Displacement versus time curve for a particle executing S.H.M. is shown in figure. Identify the points marked at which (i) velocity of the oscillator is zero, (ii) speed of the oscillator is maximum.

Draw a graph to show the variation of P.E., K.E. and total energy of a simple harmonic oscillator with displacement.
Find the displacement of a simple harmonic oscillator at which its P.E. is half of the maximum energy of the oscillator.
A body of mass m is attached to one end of a massless spring which is suspended vertically from a fixed point. The mass is held in hand so that the spring is neither stretched nor compressed. Suddenly the support of the hand is removed. The lowest position attained by the mass during oscillation is 4 cm below the point, where it was held in hand.
What is the amplitude of oscillation?
