Advertisements
Advertisements
प्रश्न
A bucket full of water is placed in a room at 15°C with initial relative humidity 40%. The volume of the room is 50 m3. (a) How much water will evaporate? (b) If the room temperature is increased by 5°C, how much more water will evaporate? The saturation vapour pressure of water at 15°C and 20°C are 1.6 kPa and 2.4 kPa respectively.
Use R = 8.3 J K-1 mol-1
उत्तर
(a) Relative humidity is given by
`(VP)/("SVP at" 15^circC)`
⇒ 0.4 = `(VP)/(1.6 xx 10^3)`
⇒VP = `0.4 xx 1.6 xx 10^3`
Evaporation occurs as long as the atmosphere is not saturated.
Net pressure change = `1.6 xx 10^3 - 0.4 xx 1.6 xx 10^3`
=`(1.6 - 0.4 xx 1.6)10^3`
=`0.96 xx 10^3`
Let the mass of water evaporated be m. Then,
⇒ `0.96 xx 10^3 xx 50 = (m xx 8.3 xx 288)/18`
⇒ `m = (0.96 xx 50 xx 18 xx 10^3)/(8.3 xx 288)`
=361.45 ≈ 361 g
(b) At `20^circC` , SVP = 2.4 KPa
At `15^circC` , SVP = 1.6 KPa
Net pressure change = `(2.4 - 1.6) xx 10^3 Pa`
= `0.8 xx 10^3 Pa`
Mass of water evaporated is given by
`m = (m^' xx 8.3 xx 293)/18`
⇒ `m^' = (0.8 xx 50 xx 18 xx 10^3)/(8.3 xx 293)`
= 296.06 ≈ 296 g
APPEARS IN
संबंधित प्रश्न
From a certain apparatus, the diffusion rate of hydrogen has an average value of 28.7 cm3 s–1. The diffusion of another gas under the same conditions is measured to have an average rate of 7.2 cm3 s–1. Identify the gas
[Hint: Use Graham’s law of diffusion: R1/R2 = (M2/M1)1/2, where R1, R2 are diffusion rates of gases 1 and 2, and M1 and M2 their respective molecular masses. The law is a simple consequence of kinetic theory.]
While gas from a cooking gas cylinder is used, the pressure does not fall appreciably till the last few minutes. Why?
Figure shows graphs of pressure vs density for an ideal gas at two temperatures T1 and T2.
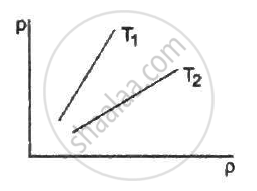
The pressure of a gas kept in an isothermal container is 200 kPa. If half the gas is removed from it, the pressure will be
Figure shows a cylindrical tube with adiabatic walls and fitted with a diathermic separator. The separator can be slid in the tube by an external mechanism. An ideal gas is injected into the two sides at equal pressures and equal temperatures. The separator remains in equilibrium at the middle. It is now slid to a position where it divides the tube in the ratio of 1:3. Find the ratio of the pressures in the two parts of the vessel.
Use R=8.314J K-1 mol-1

A vessel contains 1.60 g of oxygen and 2.80 g of nitrogen. The temperature is maintained at 300 K and the volume of the vessel is 0.166 m3. Find the pressure of the mixture.
Use R = 8.3 J K-1 mol-1
A container of volume 50 cc contains air (mean molecular weight = 28.8 g) and is open to atmosphere where the pressure is 100 kPa. The container is kept in a bath containing melting ice (0°C). (a) Find the mass of the air in the container when thermal equilibrium is reached. (b) The container is now placed in another bath containing boiling water (100°C). Find the mass of air in the container. (c) The container is now closed and placed in the melting-ice bath. Find the pressure of the air when thermal equilibrium is reached.
Use R = 8.3 J K-1 mol-1
Is a slow process always isothermal? Is a quick process always adiabatic?
In an adiabatic process on a gas with γ = 1.4, the pressure is increased by 0.5%. The volume decreases by about
A gas is enclosed in a cylindrical can fitted with a piston. The walls of the can and the piston are adiabatic. The initial pressure, volume and temperature of the gas are 100 kPa, 400 cm3 and 300 K, respectively. The ratio of the specific heat capacities of the gas, Cp / Cv = 1.5. Find the pressure and the temperature of the gas if it is (a) suddenly compressed (b) slowly compressed to 100 cm3.
The initial pressure and volume of a given mass of a gas (Cp/Cv = γ) are p0 and V0. The gas can exchange heat with the surrounding. (a) It is slowly compressed to a volume V0/2 and then suddenly compressed to V0/4. Find the final pressure. (b) If the gas is suddenly compressed from the volume V0 to V0/2 and then slowly compressed to V0/4, what will be the final pressure?
Three samples A, B and C of the same gas (γ = 1.5) have equal volumes and temperatures. The volume of each sample is doubled, the process being isothermal for A, adiabatic for B and isobaric for C. If the final pressures are equal for the three samples, find the ratio of the initial pressures.
A barometer tube is 80 cm long (above the mercury reservoir). It reads 76 cm on a particular day. A small amount of water is introduced in the tube and the reading drops to 75.4 cm. Find the relative humidity in the space above the mercury column if the saturation vapour pressure at the room temperature is 1.0 cm.
The human body has an average temperature of 98°F. Assume that the vapour pressure of the blood in the veins behaves like that of pure water. Find the minimum atmospheric pressure which is necessary to prevent the blood from boiling. Use figure for the vapour pressures.

A barometer correctly reads the atmospheric pressure as 76 cm of mercury. Water droplets are slowly introduced into the barometer tube by a dropper. The height of the mercury column first decreases and then becomes constant. If the saturation vapour pressure at the atmospheric temperature is 0.80 cm of mercury, find the height of the mercury column when it reaches its minimum value.
The temperature and humidity of air are 27°C and 50% on a particular day. Calculate the amount of vapour that should be added to 1 cubic metre of air to saturate it. The saturation vapour pressure at 27°C = 3600 Pa.
Use R = 8.3 J K-1 mol-1
The temperature and relative humidity in a room are 300 K and 20% respectively. The volume of the room is 50 m3. The saturation vapour pressure at 300 K 3.3 kPa. Calculate the mass of the water vapour present in the room.
Use R = 8.3 J K-1 mol-1
A cuboidal container having dimensions 2 m × 1.5 m × 0.5 m holds a mixture of 12 g of He, 36 g of Ar, and 20 g of Ne, If the container is maintained at 300 K, Find the pressure exerted by the mixture (given MHe = 4, MAr = 40, MNe = 20).
