Advertisements
Online Mock Tests
Chapters
▶ 2: Kinetic Theory of Gases
3: Calorimetry
4: Laws of Thermodynamics
5: Specific Heat Capacities of Gases
6: Heat Transfer
7: Electric Field and Potential
8: Gauss’s Law
9: Capacitors
10: Electric Current in Conductors
11: Thermal and Chemical Effects of Current
12: Magnetic Field
13: Magnetic Field due to a Current
14: Permanent Magnets
15: Magnetic Properties of Matter
16: Electromagnetic Induction
17: Alternating Current
18: Electromagnetic Waves
19: Electric Current through Gases
20: Photoelectric Effect and Wave-Particle Duality
21: Bohr’s Model and Physics of Atom
22: X-rays
23: Semiconductors and Semiconductor Devices
24: The Nucleus
25: The Special Theory of Relativity
![HC Verma solutions for Concepts of Physics Vol. 2 [English] Class 11 and 12 chapter 2 - Kinetic Theory of Gases HC Verma solutions for Concepts of Physics Vol. 2 [English] Class 11 and 12 chapter 2 - Kinetic Theory of Gases - Shaalaa.com](/images/9788177092325-concepts-of-physics-vol-2-english-class-11-and-12_6:cd4e4bfcb8474a60871d8e5659ec4eb9.jpg)
Advertisements
Solutions for Chapter 2: Kinetic Theory of Gases
Below listed, you can find solutions for Chapter 2 of CBSE, Karnataka Board PUC HC Verma for Concepts of Physics Vol. 2 [English] Class 11 and 12.
HC Verma solutions for Concepts of Physics Vol. 2 [English] Class 11 and 12 2 Kinetic Theory of Gases Short Answers [Page 32]
When we place a gas cylinder on a van and the van moves, does the kinetic energy of the molecules increase? Does the temperature increase?
While gas from a cooking gas cylinder is used, the pressure does not fall appreciably till the last few minutes. Why?
Do you expect the gas in a cooking gas cylinder to obey the ideal gas equation?
Can we define the temperature of (a) vacuum, (b) a single molecule?
Comment on the following statement: the temperature of all the molecules in a sample of a gas is the same.
Consider a gas of neutrons. Do you expect it to behave much better as an ideal gas as compared to hydrogen gas at the same pressure and temperature?
A gas is kept in a rigid cubical container. If a load of 10 kg is put on the top of the container, does the pressure increase?
If it were possible for a gas in a container to reach the temperature 0 K, its pressure would be zero. Would the molecules not collide with the walls? Would they not transfer momentum to the walls?
It is said that the assumptions of kinetic theory are good for gases having low densities. Suppose a container is so evacuated that only one molecule is left in it. Which of the assumptions of kinetic theory will not be valid for such a situation? Can we assign a temperature to this gas?
A gas is kept in an enclosure. The pressure of the gas is reduced by pumping out some gas. Will the temperature of the gas decrease by Charles's low?
Explain why cooking is faster in a pressure cooker.
If the molecules were not allowed to collide among themselves, would you expect more evaporation or less evaporation?
Is it possible to boil water at room temperature, say 30°C? If we touch a flask containing water boiling at this temperature, will it be hot?
When you come out of a river after a dip, you feel cold. Explain.
HC Verma solutions for Concepts of Physics Vol. 2 [English] Class 11 and 12 2 Kinetic Theory of Gases MCQ [Page 33]
Which of the following parameters is the same for molecules of all gases at a given temperature?
Mass
Speed
Momentum
Kinetic energy.
A gas behaves more closely as an ideal gas at
low pressure and low temperature
low pressure and high temperature
high pressure and low temperature
high pressure and high temperature.
The pressure of an ideal gas is written as \[P = \frac{2E}{3V}\] . Here E refers to
translational kinetic energy
rotational kinetic energy
vibrational kinetic energy
total kinetic energy.
The energy of a given sample of an ideal gas depends only on its
volume
pressure
density
temperature
Which of the following gases has maximum rms speed at a given temperature?
hydrogen
nitrogen
oxygen
carbon dioxide.
Figure shows graphs of pressure vs density for an ideal gas at two temperatures T1 and T2.
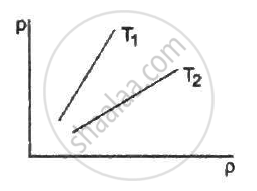
T1 > T2
T1 = T2
T1 < T2
Any of the three is possible.
The mean square speed of the molecules of a gas at absolute temperature T is proportional to
\[\frac{1}{T}\]
\[\sqrt{T}\]
T
T2
Suppose a container is evacuated to leave just one molecule of a gas in it. Let va and vrms represent the average speed and the rms speed of the gas.
va > vrms
va < vrms
va = vrms
vrms is undefined.
The rms speed of oxygen at room temperature is about 500 m/s. The rms speed of hydrogen at the same temperature is about
125 m s−1
2000 m s−1
8000 m s−1
31 m s−1.
The pressure of a gas kept in an isothermal container is 200 kPa. If half the gas is removed from it, the pressure will be
100 kPa
200 kPa
400 kPa
800 kPa
The rms speed of oxygen molecules in a gas is v. If the temperature is doubled and the oxygen molecules dissociate into oxygen atoms, the rms speed will become
v
\[v\sqrt{2}\]
2v
4v
The quantity \[\frac{pV}{kT}\] represents
mass of the gas
kinetic energy of the gas
number of moles of the gas
number of molecules in the gas.
The process on an ideal gas, shown in figure, is
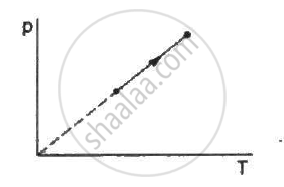
isothermal
isobaric
isochoric
none of these
There is some liquid in a closed bottle. The amount of liquid is continuously decreasing. The vapour in the remaining part
must be saturated
must be unsaturated
may be saturated
there will be no vapour.
There is some liquid in a closed bottle. The amount of liquid remains constant as time passes. The vapour in the remaining part
must be saturated
must be unsaturated
may be unsaturated
there will be no vapour.
Vapour is injected at a uniform rate in a closed vessel which was initially evacuated. The pressure in the vessel
increases continuously
decreases continuously
first increases and then decreases
first increases and then becomes constant.
A vessel A has volume V and a vessel B has volume 2V. Both contain some water which has a constant volume. The pressure in the space above water is pa for vessel A and pbfor vessel B.
pa = pb
pa = 2pb
pb = 2pa
pb = 4pa
HC Verma solutions for Concepts of Physics Vol. 2 [English] Class 11 and 12 2 Kinetic Theory of Gases MCQ [Pages 33 - 34]
Consider a collision between an oxygen molecule and a hydrogen molecule in a mixture of oxygen and hydrogen kept at room temperature. Which of the following are possible?
(a) The kinetic energies of both the molecules increase.
(b) The kinetic energies of both the molecules decrease.
(c) kinetic energy of the oxygen molecule increases and that of the hydrogen molecule decreases.
(d) The kinetic energy of the hydrogen molecule increases and that of the oxygen molecule decreases.
Consider a mixture of oxygen and hydrogen kept at room temperature. As compared to a hydrogen molecule an oxygen molecule hits the wall
with greater average speed
with smaller average speed
with greater average kinetic energy
with smaller average kinetic energy
Which of the following quantities is zero on an average for the molecules of an ideal gas in equilibrium?
Kinetic energy
Momentum
Density
Speed
Keeping the number of moles, volume and temperature the same, which of the following are the same for all ideal gases?
Rms speed of a molecule
Density
Pressure
Average magnitude of momentum.
The average momentum of a molecule in a sample of an ideal gas depends on
temperature
number of moles
volume
none of these.
Which of the following quantities is the same for all ideal gases at the same temperature?
(a) The kinetic energy of 1 mole
(b) The kinetic energy of 1 g
(c) The number of molecules in 1 mole
(d) The number of molecules in 1 g
Consider the quantity \[\frac{MkT}{pV}\] of an ideal gas where M is the mass of the gas. It depends on the
temperature of the gas
volume of the gas
pressure of the gas
nature of the gas.
HC Verma solutions for Concepts of Physics Vol. 2 [English] Class 11 and 12 2 Kinetic Theory of Gases Exercises [Pages 34 - 37]
Calculate the volume of 1 mole of an ideal gas at STP.
Find the number of molecules of an ideal gas in a volume of 1.000 cm3 at STP.
Find the number of molecules in 1 cm3 of an ideal gas at 0°C and at a pressure of 10−5mm of mercury.
Use R = 8.31 J K-1 mol-1
Calculate the mass of 1 cm3 of oxygen kept at STP.
Equal masses of air are sealed in two vessels, one of volume V0 and the other of volume 2V0. If the first vessel is maintained at a temperature 300 K and the other at 600 K, find the ratio of the pressures in the two vessels.
Use R = 8.31 JK-1 mol-1
An electric bulb of volume 250 cc was sealed during manufacturing at a pressure of 10−3 mm of mercury at 27°C. Compute the number of air molecules contained in the bulb. Avogadro constant = 6 × 1023 mol−1, density of mercury = 13600 kg m−3 and g = 10 m s−2.
Use R=8.314J K-1 mol-1
A gas cylinder has walls that can bear a maximum pressure of 1.0 × 106 Pa. It contains a gas at 8.0 × 105 Pa and 300 K. The cylinder is steadily heated. Neglecting any change in the volume, calculate the temperature at which the cylinder will break.
2 g of hydrogen is sealed in a vessel of volume 0.02 m3 and is maintained at 300 K. Calculate the pressure in the vessel.
Use R=8.3J K-1 mol-1
The density of an ideal gas is 1.25 × 10−3 g cm−3 at STP. Calculate the molecular weight of the gas.
Use R=8.31J K-1 mol-1
The temperature and pressure at Simla are 15.0°C and 72.0 cm of mercury and at Kalka these are 35.0°C and 76.0 cm of mercury. Find the ratio of air density at Kalka to the air density at Simla.
Use R=8.314J K-1 mol-1
Figure shows a cylindrical tube with adiabatic walls and fitted with a diathermic separator. The separator can be slid in the tube by an external mechanism. An ideal gas is injected into the two sides at equal pressures and equal temperatures. The separator remains in equilibrium at the middle. It is now slid to a position where it divides the tube in the ratio of 1:3. Find the ratio of the pressures in the two parts of the vessel.
Use R=8.314J K-1 mol-1

Find the rms speed of hydrogen molecules in a sample of hydrogen gas at 300 K. Find the temperature at which the rms speed is double the speed calculated in the previous part.
Use R=8.314 JK-1 mol-1
A sample of 0.177 g of an ideal gas occupies 1000 cm3 at STP. Calculate the rms speed of the gas molecules.
The average translational kinetic energy of air molecules is 0.040 eV (1 eV = 1.6 × 10−19J). Calculate the temperature of the air. Boltzmann constant k = 1.38 × 10−23 J K−1.
Consider a sample of oxygen at 300 K. Find the average time taken by a molecule to travel a distance equal to the diameter of the earth.
Use R=8.314 JK-1 mol-1
Find the average magnitude of linear momentum of a helium molecule in a sample of helium gas at 0°C. Mass of a helium molecule = 6.64 × 10−27 kg and Boltzmann constant = 1.38 × 10−23 J K−1.
The mean speed of the molecules of a hydrogen sample equals the mean speed of the molecules of a helium sample. Calculate the ratio of the temperature of the hydrogen sample to the temperature of the helium sample.
Use R = 8.314 JK-1 mol-1
At what temperature the mean speed of the molecules of hydrogen gas equals the escape speed from the earth?
Use R = 8.314 JK-1 mol-1
Find the ratio of the mean speed of hydrogen molecules to the mean speed of nitrogen molecules in a sample containing a mixture of the two gases.
Use R = 8.314 JK-1 mol-1
Figure shows a vessel partitioned by a fixed diathermic separator. Different ideal gases are filled in the two parts. The rms speed of the molecules in the left part equals the mean speed of the molecules in the right part. Calculate the ratio of the mass of a molecule in the left part to the mass of a molecule in the right part.

Estimate the number of collisions per second suffered by a molecule in a sample of hydrogen at STP. The mean free path (average distance covered by a molecule between successive collisions) = 1.38 × 10−5 cm.
Use R = 8.31 JK−1 mol−1
Hydrogen gas is contained in a closed vessel at 1 atm (100 kPa) and 300 K. (a) Calculate the mean speed of the molecules. (b) Suppose the molecules strike the wall with this speed making an average angle of 45° with it. How many molecules strike each square metre of the wall per second?
Use R = 8.31 JK-1 mol-1
Air is pumped into an automobile tyre's tube up to a pressure of 200 kPa in the morning when the air temperature is 20°C. During the day the temperature rises to 40°C and the tube expands by 2%. Calculate the pressure of the air in the tube at this temperature.
Oxygen is filled in a closed metal jar of volume 1.0 × 10−3 m3 at a pressure of 1.5 × 105Pa and temperature 400 K. The jar has a small leak in it. The atmospheric pressure is 1.0 × 105 Pa and the atmospheric temperature is 300 K. Find the mass of the gas that leaks out by the time the pressure and the temperature inside the jar equalise with the surrounding.
An air bubble of radius 2.0 mm is formed at the bottom of a 3.3 m deep river. Calculate the radius of the bubble as it comes to the surface. Atmospheric pressure = 1.0 × 105 Pa and density of water = 1000 kg m−3.
Air is pumped into the tubes of a cycle rickshaw at a pressure of 2 atm. The volume of each tube at this pressure is 0.002 m3. One of the tubes gets punctured and the volume of the tube reduces to 0.0005 m3. How many moles of air have leaked out? Assume that the temperature remains constant at 300 K and that the air behaves as an ideal gas.
Use R = 8.3 J K-1 mol-1
0.040 g of He is kept in a closed container initially at 100.0°C. The container is now heated. Neglecting the expansion of the container, calculate the temperature at which the internal energy is increased by 12 J.
Use R = 8.3 J K-1 mol-1
During an experiment, an ideal gas is found to obey an additional law pV2 = constant. The gas is initially at a temperature T and volume V. Find the temperature when it expands to a volume 2V.
Use R = 8.3 J K-1 mol-1
A vessel contains 1.60 g of oxygen and 2.80 g of nitrogen. The temperature is maintained at 300 K and the volume of the vessel is 0.166 m3. Find the pressure of the mixture.
Use R = 8.3 J K-1 mol-1
A vertical cylinder of height 100 cm contains air at a constant temperature. The top is closed by a frictionless light piston. The atmospheric pressure is equal to 75 cm of mercury. Mercury is slowly poured over the piston. Find the maximum height of the mercury column that can be put on the piston.
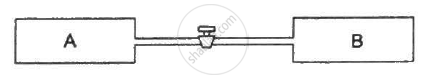
Figure shows two vessels A and B with rigid walls containing ideal gases. The pressure, temperature and the volume are pA, TA, V in the vessel A and pB, TB, V in the vessel B. The vessels are now connected through a small tube. Show that the pressure p and the temperature T satisfy `Ρ/T = 1/2 ({P_A}/{T_A}+{P_B}/{T_B))` when equilibrium is achieved.
A container of volume 50 cc contains air (mean molecular weight = 28.8 g) and is open to atmosphere where the pressure is 100 kPa. The container is kept in a bath containing melting ice (0°C). (a) Find the mass of the air in the container when thermal equilibrium is reached. (b) The container is now placed in another bath containing boiling water (100°C). Find the mass of air in the container. (c) The container is now closed and placed in the melting-ice bath. Find the pressure of the air when thermal equilibrium is reached.
Use R = 8.3 J K-1 mol-1
A uniform tube closed at one end, contains a pellet of mercury 10 cm long. When the tube is kept vertically with the closed-end upward, the length of the air column trapped is 20 cm. Find the length of the air column trapped when the tube is inverted so that the closed-end goes down. Atmospheric pressure = 75 cm of mercury.
A glass tube, sealed at both ends, is 100 cm long. It lies horizontally with the middle 10 cm containing mercury. The two ends of the tube contain air at 27°C and at a pressure 76 cm of mercury. The air column on one side is maintained at 0°C and the other side is maintained at 127°C. Calculate the length of the air column on the cooler side. Neglect the changes in the volume of mercury and of the glass.
An ideal gas is trapped between a mercury column and the closed-end of a narrow vertical tube of uniform base containing the column. The upper end of the tube is open to the atmosphere. The atmospheric pressure equals 76 cm of mercury. The lengths of the mercury column and the trapped air column are 20 cm and 43 cm respectively. What will be the length of the air column when the tube is tilted slowly in a vertical plane through an angle of 60°? Assume the temperature to remain constant.
Figure shows a cylindrical tube of length 30 cm which is partitioned by a tight-fitting separator. The separator is very weakly conducting and can freely slide along the tube. Ideal gases are filled in the two parts of the vessel. In the beginning, the temperatures in the parts A and B are 400 K and 100 K respectively. The separator slides to a momentary equilibrium position shown in the figure. Find the final equilibrium position of the separator, reached after a long time.

A vessel of volume V0 contains an ideal gas at pressure p0 and temperature T. Gas is continuously pumped out of this vessel at a constant volume-rate dV/dt = r keeping the temperature constant. The pressure of the gas being taken out equals the pressure inside the vessel. Find (a) the pressure of the gas as a function of time, (b) the time taken before half the original gas is pumped out.
Use R = 8.3 J K−1 mol−1
One mole of an ideal gas undergoes a process `P = (P_0)/(1+(V/V_0)^2` where `p_0` and `V_0` are constants . Find the temperature of the gas when `V=V_0` .
Show that the internal energy of the air (treated as an ideal gas) contained in a room remains constant as the temperature changes between day and night. Assume that the atmospheric pressure around remains constant and the air in the room maintains this pressure by communicating with the surrounding through the windows, doors, etc.
Use R = 8.314 J K-1 mol-1
Figure shows a cylindrical tube of radius 5 cm and length 20 cm. It is closed by a tight-fitting cork. The friction coefficient between the cork and the tube is 0.20. The tube contains an ideal gas at a pressure of 1 atm and a temperature of 300 K. The tube is slowly heated and it is found that the cork pops out when the temperature reaches 600 K. Let dN denote the magnitude of the normal contact force exerted by a small length dlof the cork along the periphery (see the figure). Assuming that the temperature of the gas is uniform at any instant, calculate `(dN)/(dt)`.

Figure shows a cylindrical tube of cross-sectional area A fitted with two frictionless pistons. The pistons are connected to each other by a metallic wire. Initially, the temperature of the gas is T0 and its pressure is p0 which equals the atmospheric pressure. (a) What is the tension in the wire? (b) What will be the tension if the temperature is increased to 2T0 ?

Figure shows a large closed cylindrical tank containing water. Initially the air trapped above the water surface has a height h0 and pressure 2p0 where p0 is the atmospheric pressure. There is a hole in the wall of the tank at a depth h1 below the top from which water comes out. A long vertical tube is connected as shown. (a) Find the height h2 of the water in the long tube above the top initially. (b) Find the speed with which water comes out of the hole. (c) Find the height of the water in the long tube above the top when the water stops coming out of the hole.
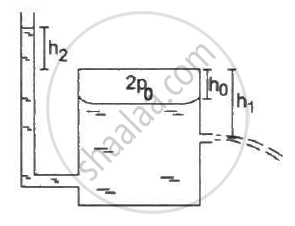
An ideal gas is kept in a long cylindrical vessel fitted with a frictionless piston of cross-sectional area 10 cm2 and weight 1 kg in figure. The vessel itself is kept in a big chamber containing air at atmospheric pressure 100 kPa. The length of the gas column is 20 cm. If the chamber is now completely evacuated by an exhaust pump, what will be the length of the gas column? Assume the temperature to remain constant throughout the process.
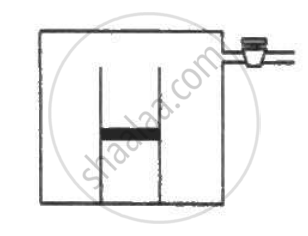
An ideal gas is kept in a long cylindrical vessel fitted with a frictionless piston of cross-sectional area 10 cm2 and weight 1 kg. The length of the gas column in the vessel is 20 cm. The atmospheric pressure is 100 kPa. The vessel is now taken into a spaceship revolving round the earth as a satellite. The air pressure in the spaceship is maintained at 100 kPa. Find the length of the gas column in the cylinder.
Use R = 8.3 J K-1 mol-1
Two glass bulbs of equal volume are connected by a narrow tube and are filled with a gas at 0°C at a pressure of 76 cm of mercury. One of the bulbs is then placed in melting ice and the other is placed in a water bath maintained at 62°C. What is the new value of the pressure inside the bulbs? The volume of the connecting tube is negligible.
The weather report reads, "Temperature 20°C : Relative humidity 100%". What is the dew point?
The condition of air in a closed room is described as follows. Temperature = 25°C, relative humidity = 60%, pressure = 104 kPa. If all the water vapour is removed from the room without changing the temperature, what will be the new pressure? The saturation vapour pressure at 25°C − 3.2 kPa.
The temperature and the dew point in an open room are 20°C and 10°C. If the room temperature drops to 15°C, what will be the new dew point?
Pure water vapour is trapped in a vessel of volume 10 cm3. The relative humidity is 40%. The vapour is compressed slowly and isothermally. Find the volume of the vapour at which it will start condensing.
A barometer tube is 80 cm long (above the mercury reservoir). It reads 76 cm on a particular day. A small amount of water is introduced in the tube and the reading drops to 75.4 cm. Find the relative humidity in the space above the mercury column if the saturation vapour pressure at the room temperature is 1.0 cm.
Using figure, find the boiling point of methyl alcohol at 1 atm (760 mm of mercury) and at 0.5 atm.

The human body has an average temperature of 98°F. Assume that the vapour pressure of the blood in the veins behaves like that of pure water. Find the minimum atmospheric pressure which is necessary to prevent the blood from boiling. Use figure for the vapour pressures.

A glass contains some water at room temperature 20°C. Refrigerated water is added to it slowly. when the temperature of the glass reaches 10°C, small droplets condense on the outer surface. Calculate the relative humidity in the room. The boiling point of water at a pressure of 17.5 mm of mercury is 20°C and at 8.9 mm of mercury it is 10°C.
50 m3 of saturated vapour is cooled down from 30°C to 20°C. Find the mass of the water condensed. The absolute humidity of saturated water vapour is 30 g m−3 at 30°C and 16 g m−3 at 20°C.
A barometer correctly reads the atmospheric pressure as 76 cm of mercury. Water droplets are slowly introduced into the barometer tube by a dropper. The height of the mercury column first decreases and then becomes constant. If the saturation vapour pressure at the atmospheric temperature is 0.80 cm of mercury, find the height of the mercury column when it reaches its minimum value.
50 cc of oxygen is collected in an inverted gas jar over water. The atmospheric pressure is 99.4 kPa and the room temperature is 27°C. The water level in the jar is same as the level outside. The saturation vapour pressure at 27°C is 3.4 kPa. Calculate the number of moles of oxygen collected in the jar.
A faulty barometer contains certain amount of air and saturated water vapour. It reads 74.0 cm when the atmospheric pressure is 76.0 cm of mercury and reads 72.10 cm when the atmospheric pressure is 74.0 cm of mercury. Saturation vapour pressure at the air temperature = 1.0 cm of mercury. Find the length of the barometer tube above the mercury level in the reservoir.
On a winter day, the outside temperature is 0°C and relative humidity 40%. The air from outside comes into a room and is heated to 20°C. What is the relative humidity in the room? The saturation vapour pressure at 0°C is 4.6 mm of mercury and at 20°C it is 18 mm of mercury.
The temperature and humidity of air are 27°C and 50% on a particular day. Calculate the amount of vapour that should be added to 1 cubic metre of air to saturate it. The saturation vapour pressure at 27°C = 3600 Pa.
Use R = 8.3 J K-1 mol-1
The temperature and relative humidity in a room are 300 K and 20% respectively. The volume of the room is 50 m3. The saturation vapour pressure at 300 K 3.3 kPa. Calculate the mass of the water vapour present in the room.
Use R = 8.3 J K-1 mol-1
The temperature and the relative humidity are 300 K and 20% in a room of volume 50 m3. The floor is washed with water, 500 g of water sticking on the floor. Assuming no communication with the surrounding, find the relative humidity when the floor dries. The changes in temperature and pressure may be neglected. Saturation vapour pressure at 300 K = 3.3 kPa.
Use R = 8.31 J K-1 mol-1
A bucket full of water is placed in a room at 15°C with initial relative humidity 40%. The volume of the room is 50 m3. (a) How much water will evaporate? (b) If the room temperature is increased by 5°C, how much more water will evaporate? The saturation vapour pressure of water at 15°C and 20°C are 1.6 kPa and 2.4 kPa respectively.
Use R = 8.3 J K-1 mol-1
Solutions for 2: Kinetic Theory of Gases
![HC Verma solutions for Concepts of Physics Vol. 2 [English] Class 11 and 12 chapter 2 - Kinetic Theory of Gases HC Verma solutions for Concepts of Physics Vol. 2 [English] Class 11 and 12 chapter 2 - Kinetic Theory of Gases - Shaalaa.com](/images/9788177092325-concepts-of-physics-vol-2-english-class-11-and-12_6:cd4e4bfcb8474a60871d8e5659ec4eb9.jpg)
HC Verma solutions for Concepts of Physics Vol. 2 [English] Class 11 and 12 chapter 2 - Kinetic Theory of Gases
Shaalaa.com has the CBSE, Karnataka Board PUC Mathematics Concepts of Physics Vol. 2 [English] Class 11 and 12 CBSE, Karnataka Board PUC solutions in a manner that help students grasp basic concepts better and faster. The detailed, step-by-step solutions will help you understand the concepts better and clarify any confusion. HC Verma solutions for Mathematics Concepts of Physics Vol. 2 [English] Class 11 and 12 CBSE, Karnataka Board PUC 2 (Kinetic Theory of Gases) include all questions with answers and detailed explanations. This will clear students' doubts about questions and improve their application skills while preparing for board exams.
Further, we at Shaalaa.com provide such solutions so students can prepare for written exams. HC Verma textbook solutions can be a core help for self-study and provide excellent self-help guidance for students.
Concepts covered in Concepts of Physics Vol. 2 [English] Class 11 and 12 chapter 2 Kinetic Theory of Gases are Specific Heat Capacities - Gases, Mean Free Path, Kinetic Theory of Gases - Concept of Pressure, Equation of State of a Perfect Gas, Work Done in Compressing a Gas, Introduction of Kinetic Theory of an Ideal Gas, Interpretation of Temperature in Kinetic Theory, Gases and Its Characteristics, Assumptions of Kinetic Theory of Gases, RMS Speed of Gas Molecules, Degrees of Freedom, Avogadro's Number, Molecular Nature of Matter, Law of Equipartition of Energy.
Using HC Verma Concepts of Physics Vol. 2 [English] Class 11 and 12 solutions Kinetic Theory of Gases exercise by students is an easy way to prepare for the exams, as they involve solutions arranged chapter-wise and also page-wise. The questions involved in HC Verma Solutions are essential questions that can be asked in the final exam. Maximum CBSE, Karnataka Board PUC Concepts of Physics Vol. 2 [English] Class 11 and 12 students prefer HC Verma Textbook Solutions to score more in exams.
Get the free view of Chapter 2, Kinetic Theory of Gases Concepts of Physics Vol. 2 [English] Class 11 and 12 additional questions for Mathematics Concepts of Physics Vol. 2 [English] Class 11 and 12 CBSE, Karnataka Board PUC, and you can use Shaalaa.com to keep it handy for your exam preparation.
