HC Verma solutions for Concepts of Physics Vol. 2 [English] Class 11 and 12 chapter 21 - Bohr’s Model and Physics of Atom [Latest edition]

How many wavelengths are emitted by atomic hydrogen in visible range (380 nm − 780 nm)? In the range 50 nm to 100 nm?

The first excited energy of a He⁺ ion is the same as the ground state energy of hydrogen. Is it always true that one of the energies of any hydrogen-like ion will be the same as the ground state energy of a hydrogen atom?

Which wavelengths will be emitted by a sample of atomic hydrogen gas (in ground state) if electrons of energy 12.2 eV collide with the atoms of the gas?

When white radiation is passed through a sample of hydrogen gas at room temperature, absorption lines are observed in Lyman series only. Explain.

Balmer series was observed and analysed before the other series. Can you suggest a reason for such an order?

What will be the energy corresponding to the first excited state of a hydrogen atom if the potential energy of the atom is taken to be 10 eV when the electron is widely separated from the proton? Can we still write E_n = E₁/n², or r_n = a₀ n²?

The difference in the frequencies of series limit of Lyman series and Balmer series is equal to the frequency of the first line of the Lyman series. Explain.

The numerical value of ionization energy in eV equals the ionization potential in volts. Does the equality hold if these quantities are measured in some other units?

We have stimulated emission and spontaneous emission. Do we also have stimulated absorption and spontaneous absorption?

An atom is in its excited state. Does the probability of its coming to ground state depend on whether the radiation is already present or not? If yes, does it also depend on the wavelength of the radiation present?

The minimum orbital angular momentum of the electron in a hydrogen atom is

Three photons coming from excited atomic-hydrogen sample are picked up. Their energies are 12.1 eV, 10.2 eV and 1.9 eV. These photons must come from

Suppose, the electron in a hydrogen atom makes transition from n = 3 to n = 2 in 10⁻⁸ s. The order of the torque acting on the electron in this period, using the relation between torque and angular momentum as discussed in the chapter on rotational mechanics is

In which of the following transitions will the wavelength be minimum? 

In which of the following systems will the radius of the first orbit (n = 1) be minimum?

In which of the following systems will the wavelength corresponding to n = 2 to n = 1 be minimum?

Which of the following curves may represent the speed of the electron in a hydrogen atom as a function of trincipal quantum number n?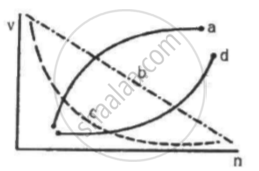

As one considers orbits with higher values of n in a hydrogen atom, the electric potential energy of the atom

The energy of an atom (or ion) in its ground state is −54.4 eV. It may be

The radius of the shortest orbit in a one-electron system is 18 pm. It may be

A hydrogen atom in ground state absorbs 10.2 eV of energy. The orbital angular momentum of the electron is increased by

Which of the following parameters are the same for all hydrogen-like atoms and ions in their ground states?

In a laser tube, all the photons

In a laboratory experiment on emission from atomic hydrogen in a discharge tube, only a small number of lines are observed whereas a large number of lines are present in the hydrogen spectrum of a star. This is because in a laboratory

An electron with kinetic energy 5 eV is incident on a hydrogen atom in its ground state. The collision

Which of the following products in a hydrogen atom are independent of the principal quantum number n? The symbols have their usual meanings.

(a) vn
(b) Er
(c) En
(d) vr

Let A_n be the area enclosed by the nth orbit in a hydrogen atom. The graph of ln (A_n/A₁) against ln(n)

(a) will pass through the origin
(b) will be a straight line with slope 4
(c) will be a monotonically increasing nonlinear curve
(d) will be a circle

Ionization energy of a hydrogen-like ion A is greater than that of another hydrogen-like ion B. Let r, u, E and L represent the radius of the orbit, speed of the electron, energy of the atom and orbital angular momentum of the electron respectively. In ground state

When a photon stimulates the emission of another photon, the two photons have
(a) same energy
(b) same direction
(c) same phase
(d) same wavelength

The Bohr radius is given by  `a_0 = (∈_0h^2)/{pime^2}`. Verify that the RHS has dimensions of length.

Find the wavelength of the radiation emitted by hydrogen in the transitions (a) n = 3 to n= 2, (b) n = 5 to n = 4 and (c) n = 10 to n = 9.

Calculate the smallest wavelength of radiation that may be emitted by (a) hydrogen, (b) He⁺ and (c) Li⁺⁺.

Evaluate Rydberg constant by putting the values of the fundamental constants in its expression.

Find the binding energy of a hydrogen atom in the state n = 2.

A hydrogen atom emits ultraviolet radiation of wavelength 102.5 nm. What are the quantum numbers of the states involved in the transition?

(a) Find the first excitation potential of He⁺ ion. (b) Find the ionization potential of Li⁺⁺ion.

A group of hydrogen atoms are prepared in n = 4 states. List the wavelength that are emitted as the atoms make transitions and return to n = 2 states.

A positive ion having just one electron ejects it if a photon of wavelength 228 Å or less is absorbed by it. Identify the ion.

Find the maximum Coulomb force that can act on the electron due to the nucleus in a hydrogen atom.

A hydrogen atom in a state having a binding energy of 0.85 eV makes transition to a state with excitation energy 10.2 e.V (a) Identify the quantum numbers n of the upper and the lower energy states involved in the transition. (b) Find the wavelength of the emitted radiation.

Whenever a photon is emitted by hydrogen in Balmer series, it is followed by another photon in Lyman series. What wavelength does this latter photon correspond to?

A hydrogen atom in state n = 6 makes two successive transitions and reaches the ground state. In the first transition a photon of 1.13 eV is emitted. (a) Find the energy of the photon emitted in the second transition (b) What is the value of n in the intermediate state?

What is the energy of a hydrogen atom in the first excited state if the potential energy is taken to be zero in the ground state?

A hot gas emits radiation of wavelengths 46.0 nm, 82.8 nm and 103.5 nm only. Assume that the atoms have only two excited states and the difference between consecutive energy levels decreases as energy is increased. Taking the energy of the highest energy state to be zero, find the energies of the ground state and the first excited state.

Find the maximum angular speed of the electron of a hydrogen atom in a stationary orbit.

A spectroscopic instrument can resolve two nearby wavelengths λ and λ + Δλ if λ/Δλ is smaller than 8000. This is used to study the spectral lines of the Balmer series of hydrogen. Approximately how many lines will be resolved by the instrument?

Suppose, in certain conditions only those transitions are allowed to hydrogen atoms in which the principal quantum number n changes by 2. (a) Find the smallest wavelength emitted by hydrogen. (b) List the wavelength emitted by hydrogen in the visible range (380 nm to 780 nm).

According to Maxwell's theory of electrodynamics, an electron going in a circle should emit radiation of frequency equal to its frequency of revolution. What should be the wavelength of the radiation emitted by a hydrogen atom in ground state if this rule is followed?

The average kinetic energy of molecules in a gas at temperature T is 1.5 kT. Find the temperature at which the average kinetic energy of the molecules of hydrogen equals the binding energy of its atoms. Will hydrogen remain in molecular from at this temperature? Take k = 8.62 × 10⁻⁵ eV K⁻¹.

Find the temperature at which the average thermal kinetic energy is equal to the energy needed to take a hydrogen atom from its ground state to n = 3 state. Hydrogen can now emit red light of wavelength 653.1 nm. Because of Maxwellian distribution of speeds, a hydrogen sample emits red light at temperatures much lower than that obtained from this problem. Assume that hydrogen molecules dissociate into atoms.

Average lifetime of a hydrogen atom excited to n = 2 state is 10⁻⁸ s. Find the number of revolutions made by the electron on the average before it jumps to the ground state.

Calculate the magnetic dipole moment corresponding to the motion of the electron in the ground state of a hydrogen atom.

Show that the ratio of the magnetic dipole moment to the angular momentum (l = mvr) is a universal constant for hydrogen-like atoms and ions. Find its value. 

A beam of  light having wavelengths distributed uniformly between 450 nm to 550 nm passes through a sample of hydrogen gas. Which wavelength will have the least intensity in the transmitted beam?

Radiation coming from transition n = 2 to n = 1 of hydrogen atoms falls on helium ions in n = 1 and n = 2 states. What are the possible transitions of helium ions as they absorbs energy from the radiation?

A hydrogen atom in ground state absorbs a photon of ultraviolet radiation of wavelength 50 nm. Assuming that the entire photon energy is taken up by the electron with what kinetic energy will the electron be ejected?

A parallel beam of light of wavelength 100 nm passes through a sample of atomic hydrogen gas in ground state. (a) Assume that when a photon supplies some of its energy to a hydrogen atom, the rest of the energy appears as another photon. Neglecting the light emitted by the excited hydrogen atoms in the direction of the incident beam, what wavelengths may be observed in the transmitted beam? (b) A radiation detector is placed near the gas to detect radiation coming perpendicular to the incident beam. Find the wavelengths of radiation that may be detected by the detector.

A beam of monochromatic light of wavelength λ ejects photoelectrons from a cesium surface (Φ = 1.9 eV). These photoelectrons are made to collide with hydrogen atoms in ground state. Find the maximum value of λ for which (a) hydrogen atoms may be ionized, (b) hydrogen atoms may get excited from the ground state to the first excited state and (c) the excited hydrogen atoms may emit visible light.

Electrons are emitted from an electron gun at almost zero velocity and are accelerated by an electric field E through a distance of 1.0 m. The electrons are now scattered by an atomic hydrogen sample in ground state. What should be the minimum value of E so that red light of wavelength 656.3 nm may be emitted by the hydrogen?

A neutron having kinetic energy 12.5 eV collides with a hydrogen atom at rest. Nelgect the difference in mass between the neutron and the hydrogen atom and assume that the neutron does not leave its line of motion. Find the possible kinetic energies of the neutron after the event.

A hydrogen atom moving at speed υ collides with another hydrogen atom kept at rest. Find the minimum value of υ for which one of the atoms may get ionized.
The mass of a hydrogen atom = 1.67 × 10⁻²⁷ kg.

A neutron moving with a speed υ strikes a hydrogen atom in ground state moving towards it with the same speed. Find the minimum speed of the neutron for which inelastic (completely or partially) collision may take place. The mass of neutron = mass of hydrogen = 1.67 × 10⁻²⁷ kg.v

When a photon is emitted by a hydrogen atom, the photon carries a momentum with it. (a) Calculate the momentum carries by the photon when a hydrogen atom emits light of wavelength 656.3 nm. (b) With what speed does the atom recoil during this transition? Take the mass of the hydrogen atom = 1.67 × 10⁻²⁷ kg. (c) Find the kinetic energy of recoil of the atom.

When a photon is emitted from an atom, the atom recoils. The kinetic energy of recoil and the energy of the photon come from the difference in energies between the states involved in the transition. Suppose, a hydrogen atom changes its state from n = 3 to n = 2. Calculate the fractional change in the wavelength of light emitted, due to the recoil.

The light emitted in the transition n = 3 to n = 2 in hydrogen is called H_α light. Find the maximum work function a metal can have so that H_α light can emit photoelectrons from it.

Light from Balmer series of hydrogen is able to eject photoelectrons from a metal. What can be the maximum work function of the metal?

Radiation from hydrogen discharge tube falls on a cesium plate. Find the maximum possible kinetic energy of the photoelectrons. Work function of cesium is 1.9 eV.

A filter transmits only the radiation of wavelength greater than 440 nm. Radiation from a hydrogen-discharge tube goes through such a filter and is incident on a metal of work function 2.0 eV. Find the stopping potential which can stop the photoelectrons.

The earth revolves round the sun due to gravitational attraction. Suppose that the sun and the earth are point particles with their existing masses and that Bohr's quantization rule for angular momentum is valid in the case of gravitation. (a) Calculate the minimum radius the earth can have for its orbit. (b) What is the value of the principal quantum number n for the present radius? Mass of the earth = 6.0 × 10⁻²⁴ kg. Mass of the sun = 2.0 × 10³⁰ kg, earth-sun distance = 1.5 × 10¹¹ m.

Consider a neutron and an electron bound to each other due to gravitational force. Assuming Bohr's quantization rule for angular momentum to be valid in this case, derive an expression for the energy of the neutron-electron system.

A uniform magnetic field B exist in a region. An electron projected perpendicular to the field goes in a circle. Assuming Bohr's quantization rule for angular momentum, calculate (a) the smallest possible radius of the electron (b) the radius of the nth orbit and (c) the minimum possible speed of the electron.

Suppose in an imaginary world the angular momentum is quantized to be even integral multiples of h/2π. What is the longest possible wavelength emitted by hydrogen atoms in visible range in such a world according to Bohr's model?

Consider an excited hydrogen atom in state n moving with a velocity υ(ν<<c). It emits a photon in the direction of its motion and changes its state to a lower state m. Apply momentum and energy conservation principles to calculate the frequency ν of the emitted radiation. Compare this with the frequency ν₀ emitted if the atom were at rest.