Advertisements
Advertisements
प्रश्न
A certain number of metallic cones, each of radius 2 cm and height 3 cm are melted and recast into a solid sphere of radius 6 cm. Find the number of cones.
उत्तर
Let the number of cones be n.
Let the radius of the sphere be rs =6 cm
Radius of a cone be rc =2 cm
And the height of the cone be h =3 cm
A volume of sphere = n(Volume of a metallic cone)
`=> 4/3 pir_s^3 = n(1/3 pir_c^2 h)`
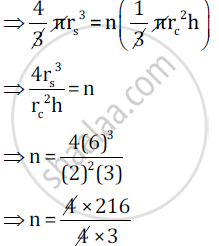
n = 72
Hence, the number of cones is 72.
APPEARS IN
संबंधित प्रश्न
Find the surface area of a sphere of diameter 14 cm.
`["Assume "pi=22/7]`
A model of a ship is made to a scale 1: 300
1) The length of the model of the ship is 2 m. Calculate the lengths of the ship.
2) The area of the deck ship is 180,000 m2. Calculate the area of the deck of the model.
3) The volume of the model in 6.5 m3. Calculate the volume of the ship.
The surface area of a solid metallic sphere is 2464 cm2. It is melted and recast into solid right circular cones of radius 3.5 cm and height 7 cm. Calculate:
- the radius of the sphere.
- the number of cones recast. (Take π = `22/7`)
A solid cone of radius 5 cm and height 8 cm is melted and made into small spheres of radius 0.5 cm. Find the number of spheres formed.
Find the surface area of a sphere of radius 14 cm.
Total volume of three identical cones is the same as that of a bigger cone whose height is 9 cm and diameter 40 cm. Find the radius of the base of each smaller cone, if height of each is 108 cm.
Find the maximum volume of a cone that can be carved out of a solid hemisphere of radius r cm.
A largest sphere is to be carved out of a right circular cylinder of radius 7 cm and height 14 cm. Find the volume of the sphere.
Find the volume of a sphere whose surface area is 154 cm2.
If a hollow sphere of internal and external diameters 4 cm and 8 cm respectively melted into a cone of base diameter 8 cm, then find the height of the cone.
If a solid sphere of radius 10 cm is moulded into 8 spherical solid balls of equal radius, then the surface area of each ball (in sq.cm) is
If a sphere is inscribed in a cube, then the ratio of the volume of the sphere to the volume of the cube is
If the radius of a solid hemisphere is 5 cm, then find its curved surface area and total surface area. ( π = 3.14 )
The radius of a sphere is 9 cm. It is melted and drawn into a wire of diameter 2 mm. Find the length of the wire in metre.
Find the length of the wire of diameter 4 m that can be drawn from a solid sphere of radius 9 m.
Find the volume of the hollow sphere whose inner diameter is 8 cm and the thickness of the material of which it is made is 1 cm .
A conical tent is to accommodate 77 persons. Each person must have 16 m3 of air to breathe. Given the radius of the tent as 7 m, find the height of the tent and also its curved surface area.
The radius of two spheres are in the ratio of 1 : 3. Find the ratio between their volume.
The cylinder of radius 12 cm have filled the 20 cm with water. One piece of iron drop in the stands of water goes up 6.75 cm. Find the radius of sphere piece.
The total surface area of a hemisphere is how many times the square of its radius
