Advertisements
Advertisements
प्रश्न
A copper wire, 3 mm in diameter, is wound about a cylinder whose length is 12 cm, and diameter 10 cm, so as to cover the curved surface of the cylinder. Find the length and mass of the wire, assuming the density of copper to be 8.88 g per cm3.
उत्तर
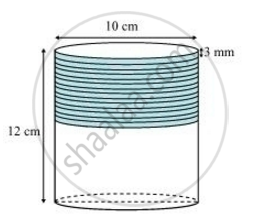
It can be observed that 1 round of wire will cover 3 mm height of cylinder.
Number of rounds = Height of cylinder/Diameter of wire
= 12/0.3 = 40 rounds
Length of wire required in 1 round = Circumference of base of cylinder
= 2πr = 2π × 5 = 10π
Length of wire in 40 rounds = 40 × 10π
`= (400xx22)/7 = 8800/7`
= 1257.14 cm = 12.57 m
Radius of wire = 0.3/2 = 0.15 cm
Volume of wire = Area of cross-section of wire × Length of wire
= π(0.15)2 × 1257.14
= 88.898 cm3
Mass = Volume × Density
= 88.898 × 8.88
= 789.41 gm
APPEARS IN
संबंधित प्रश्न
Due to heavy floods in a state, thousands were rendered homeless. 50 schools collectively offered to the state government to provide place and the canvas for 1500 tents to be fixed by the governments and decided to share the whole expenditure equally. The lower part of each tent is cylindrical of base radius 2.8 cm and height 3.5 m, with conical upper part of same base radius but of height 2.1 m. If the canvas used to make the tents costs Rs. 120 per sq. m, find the amount shared by each school to set up the tents. What value is generated by the above problem? (use `pi =22/7`)
Two spheres of same metal weigh 1 kg and 7 kg. The radius of the smaller sphere is 3 cm. The two spheres are melted to form a single big sphere. Find the diameter of the new sphere ?
The capacity of a certain cuboidal tank is 50000 litres of water. Find the breadth of the tank, if its height and length are 10 m and 2.5 m respectively.
Lead spheres of diameter 6 cm are dropped into a cylindrical beaker containing some water and are fully submerged. If the diameter of the beaker is 18 cm and water rises by 40 cm. find the number of lead spheres dropped in the water.
The height of a cone is 30 cm. A small cone is cut off at the top by a plane parallel to the base. If its volume be \[\frac{1}{27}\] of the volume of the given cone, then the height above the base at which the section has been made, is
In a village, a well with 10 m inside diameter, is dug 14 m deep. Earth taken out of it is spread all around to a width 5 m to form an embankment. Find the height of the embankment. What value of the villagers is reflected here?
Arrange the given objects according to their volume
Two identical cubes each of volume 64 cm3 are joined together end to end. What is the surface area of the resulting cuboid?
______ surface area of room = area of 4 walls.
The radius of a metal sphere is 3 cm. The sphere is melted and made into a long wire of uniform circular cross-section, whose length is 36 cm. To calculate the radius of wire, complete the following activity.
Radius of the sphere = `square`
Length of the wire = `square`
Let the radius of the wire by r cm.
Now, Volume of the wire = Volume of the `square`
`square` = `square`
r2 × `square` = `square` × `square`
r2 × `square` = `square`
r = `square`
Hence, the radius of the wire is `square` cm.
