Advertisements
Advertisements
प्रश्न
A cylindrical log of wood of height h and area of cross-section A floats in water. It is pressed and then released. Show that the log would execute S.H.M. with a time period. `T = 2πsqrt(m/(Apg))` where m is mass of the body and ρ is density of the liquid.
उत्तर
Consider the diagram,
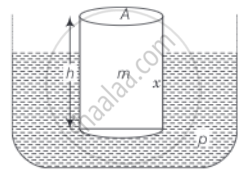
Let the log be pressed and let the vertical displacement at the equilibrium position be x0.
At equilibrium, mg = buoyant force = (ρAx0)g ......[∵ m = Vρ = (Ax0)ρ]
When it is displaced by a further displacement x, the buoyant force is A(x0 + x)ρg
∴ Net restoring force = Buoyant force – Weight
= A(x0 + x)ρg – mg
= (Aρg)x .....[∵ mg = ρAx0g]
As displacement x is downward and restoring force is upward, We can write
`F_"restoring" = - (Aρg)x`
= `- kx`
Where k = constant = Aρg
So, the motion is S.H.M .....(∵ F ∝ – x)
Now, Acceleration a = `F_"restoring"/m = - k/m x`
Comparing with a = `- ω^2x`
⇒ `ω^2 = k/m`
⇒ `ω = sqrt(k/m)`
⇒ `(2pi)/T = sqrt(k/m)`
⇒ `T = 2pi sqrt(m/k)`
⇒ `T = 2pi sqrt(m/(Aρg))`
APPEARS IN
संबंधित प्रश्न
The phase difference between displacement and acceleration of a particle performing S.H.M. is _______.
(A) `pi/2rad`
(B) π rad
(C) 2π rad
(D)`(3pi)/2rad`
let us take the position of mass when the spring is unstretched as x = 0, and the direction from left to right as the positive direction of the x-axis. Give x as a function of time t for the oscillating mass if at the moment we start the stopwatch (t = 0), the mass is
(a) at the mean position,
(b) at the maximum stretched position, and
(c) at the maximum compressed position.
In what way do these functions for SHM differ from each other, in frequency, in amplitude or the initial phase?
The cylindrical piece of the cork of density of base area A and height h floats in a liquid of density `rho_1`. The cork is depressed slightly and then released. Show that the cork oscillates up and down simple harmonically with a period
`T = 2pi sqrt((hrho)/(rho_1g)`
where ρ is the density of cork. (Ignore damping due to viscosity of the liquid).
If the maximum velocity and acceleration of a particle executing SHM are equal in magnitude, the time period will be ______.
The period of oscillation of a simple pendulum of constant length at the surface of the earth is T. Its time period inside mine will be ______.
The relation between acceleration and displacement of four particles are given below: Which one of the particles is executing simple harmonic motion?
A particle executing S.H.M. has a maximum speed of 30 cm/s and a maximum acceleration of 60 cm/s2. The period of oscillation is ______.
Which of the following statements is/are true for a simple harmonic oscillator?
- Force acting is directly proportional to displacement from the mean position and opposite to it.
- Motion is periodic.
- Acceleration of the oscillator is constant.
- The velocity is periodic.
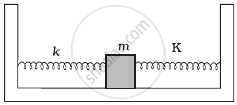
Two identical springs of spring constant K are attached to a block of mass m and to fixed supports as shown in figure. When the mass is displaced from equilibrium position by a distance x towards right, find the restoring force
A tunnel is dug through the centre of the Earth. Show that a body of mass ‘m’ when dropped from rest from one end of the tunnel will execute simple harmonic motion.
