Advertisements
Advertisements
प्रश्न
A charged particle goes undeflected in a region containing an electric and a magnetic field. It is possible that
(a) `vecE" || "vecB , vecv" || " vec E `
(b) `vecE "is not parallel" vecB`
(c) `vecv " || " vecB but vecv "is not parallel"`
(d) `vecE" || " vecB but vecv "is not parallel"`
उत्तर
(a) `vecE" || "vecB , vecv" || " vec E `
(b) `vecE "is not parallel to B"`
In option (a) velocity, electric field and magnetic field are parallel to each other. So, the particle may accelerate but always continue to travel in the same straight path or go undeflected.
Another possibility of the particle to go undeviated is that magnetic force acting on it is counterbalanced by electric force. This is possible if all the three, i.e. velocity, magnetic field and electric field are perpendicular to each other, so that magnetic force is balanced by electric force. So option (b) can also be one possibility. But (c) and (d) are wrong statements.
APPEARS IN
संबंधित प्रश्न
Two infinitely long straight parallel wires, '1' and '2', carrying steady currents I1 and I2 in the same direction are separated by a distance d. Obtain the expression for the magnetic field `vecB`due to the wire '1' acting on wire '2'. Hence find out, with the help of a suitable diagram, the magnitude and direction of this force per unit length on wire '2' due to wire '1'. How does the nature of this force changes if the currents are in opposite direction? Use this expression to define the S.I. unit of current.
and ```vecE` and `vecB`denote electric and magnetic fields in a frame S and `vecE`→ and `vecB` in another frame S' moving with respect to S at a velocity `vecV` Two of the following equations are wrong. Identify them.
(a) `B_y^, = B_y + (vE_z)/c^2`
(b) `E_y^' = E_y - (vB_z)/(c^2)`
`(c) Ey = By + vE_z`
`(d) E_y = E_y + vB_z`
Two parallel, long wires carry currents i1 and i2 with i1 > i2. When the currents are in the same direction, the magnetic field at a point midway between the wires is 10 µT. If the direction of i2 is reversed, the field becomes 30 µT. The ratio i1/i2 is
A copper wire of diameter 1.6 mm carries a current of 20 A. Find the maximum magnitude of the magnetic field `vecB` due to this current.
The magnetic field existing in a region is given by `vecB = B_0(1 + x/1)veck` . A square loop of edge l and carrying a current i, is placed with its edges parallel to the x−y axes. Find the magnitude of the net magnetic force experienced by the loop.
Two parallel wires carry equal currents of 10 A along the same direction and are separated by a distance of 2.0 cm. Find the magnetic field at a point which is 2.0 cm away from each of these wires.
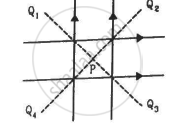
Four long, straight wires, each carrying a current of 5.0 A, are placed in a plane as shown in figure. The points of intersection form a square of side 5.0 cm.
(a) Find the magnetic field at the centre P of the square.
(b) Q1, Q2, Q3, and Q4, are points situated on the diagonals of the square and at a distance from P that is equal to the diagonal of the square. Find the magnetic fields at these points.
Consider a 10-cm long piece of a wire which carries a current of 10 A. Find the magnitude of the magnetic field due to the piece at a point which makes an equilateral triangle with the ends of the piece.
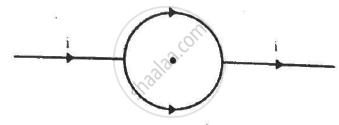
A conducting circular loop of radius a is connected to two long, straight wires. The straight wires carry a current i as shown in figure. Find the magnetic field B at the centre of the loop.
If a current I is flowing in a straight wire parallel to x-axis and magnetic field is there in the y-axis then, ______.
According to Ampere's circuital law, ______.
A milli voltmeter of 25 milli volt range is to be converted into an ammeter of 25 ampere range. The value (in ohm) of necessary shunt will be ______.
The nature of parallel and anti-parallel currents are ______.
Equal currents are passing through two very long and straight parallel wires in the same direction. They will ______
Do magnetic forces obey Newton’s third law. Verify for two current elements dl1 = dlî located at the origin and dl2 = dlĵ located at (0, R, 0). Both carry current I.
Five long wires A, B, C, D and E, each carrying current I are arranged to form edges of a pentagonal prism as shown in figure. Each carries current out of the plane of paper.
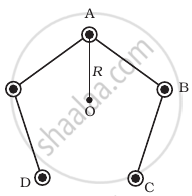
- What will be magnetic induction at a point on the axis O? AxisE is at a distance R from each wire.
- What will be the field if current in one of the wires (say A) is switched off?
- What if current in one of the wire (say) A is reversed?
