Advertisements
Advertisements
प्रश्न
A function f: R→ R defined by f(x) = `(3x) /5 + 2`, x ∈ R. Show that f is one-one and onto. Hence find f−1.
उत्तर
f: R → R defined by f(x) = `(3x)/5 + 2`
First we have to prove that f is one-one function for that we have to prove if f(x1) = f(x2) then x1 = x2
Here f(x) = `(3x)/5 + 2`
Let f(x1) = f(x2)
∴ `(3x_1)/5 + 2 = (3x_2)/5 + 2`
∴ `(3x_1)/5 = (3x_2)/5`
∴ x1 = x2
∴ f is a one-one function.
Now, we have to prove that f is an onto function. Let y ∈ R be such that
y = f(x)
∴ y = `(3x)/5 + 2`
∴ y – 2 =`(3x)/5`
∴ `x = (5(y-2))/3 ∈ R`
∴ for any y ∈ co-domain R, there exist an element x = `(5(y-2))/3` ∈ domain R such that f(x) = y
∴ f is an onto function.
∴ f is one-one onto function.
∴ f-1 exists
∴ f-1(y) = `(5(y-2))/3`
∴ f -1(x) = `(5(x-2))/3`
APPEARS IN
संबंधित प्रश्न
Let S = {a, b, c} and T = {1, 2, 3}. Find F−1 of the following functions F from S to T, if it exists.
F = {(a, 2), (b, 1), (c, 1)}
Classify the following function as injection, surjection or bijection : f : Z → Z given by f(x) = x2
Classify the following function as injection, surjection or bijection :
f : R → R, defined by f(x) = x3 − x
Classify the following function as injection, surjection or bijection :
f : Q → Q, defined by f(x) = x3 + 1
Find gof and fog when f : R → R and g : R → R is defined by f(x) = x2 + 8 and g(x) = 3x3 + 1 .
Find fog and gof if : f (x) = |x|, g (x) = sin x .
Find fog and gof if : f(x)= x + 1, g (x) = 2x + 3 .
Let f, g, h be real functions given by f(x) = sin x, g (x) = 2x and h (x) = cos x. Prove that fog = go (fh).
Let f : R → R+ be defined by f(x) = ax, a > 0 and a ≠ 1. Write f−1 (x).
Let A = {a, b, c, d} and f : A → A be given by f = {( a,b ),( b , d ),( c , a ) , ( d , c )} write `f^-1`. [NCERT EXEMPLAR]
If a function\[f : [2, \infty )\text{ to B defined by f}\left( x \right) = x^2 - 4x + 5\] is a bijection, then B =
If \[g \left( f \left( x \right) \right) = \left| \sin x \right| \text{and} f \left( g \left( x \right) \right) = \left( \sin \sqrt{x} \right)^2 , \text{then}\]
Let \[f\left(x\right) = x^3\] be a function with domain {0, 1, 2, 3}. Then domain of \[f^{-1}\] is ______.
For sets A, B and C, let f: A → B, g: B → C be functions such that g o f is surjective. Then g is surjective.
Let A = {1, 2, 3, ...n} and B = {a, b}. Then the number of surjections from A into B is ______.
Let f : R → R be defind by f(x) = `1/"x" AA "x" in "R".` Then f is ____________.
Number of integral values of x satisfying the inequality `(3/4)^(6x + 10 - x^2) < 27/64` is ______.
`x^(log_5x) > 5` implies ______.
If f: [0, 1]→[0, 1] is defined by f(x) = `(x + 1)/4` and `d/(dx) underbrace(((fofof......of)(x)))_("n" "times")""|_(x = 1/2) = 1/"m"^"n"`, m ∈ N, then the value of 'm' is ______.
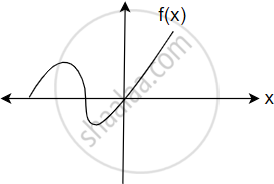
The given function f : R → R is not ‘onto’ function. Give reason.
