Advertisements
Advertisements
प्रश्न
Let \[f\left(x\right) = x^3\] be a function with domain {0, 1, 2, 3}. Then domain of \[f^{-1}\] is ______.
विकल्प
{3, 2, 1, 0}
{0, −1, −2, −3}
{0, 1, 8, 27}
{0, −1, −8, −27}
उत्तर
Let f(x) = x3 be a function with domain {0, 1, 2, 3}. Then domain of f-1 is {0, 1, 8, 27}.
Explanation:
f(x) = x3
Domain = {0, 1, 2, 3}
Range = {03, 13, 23, 33} = {0, 1, 8, 27}
So, f = {(0, 0), (1, 1), (2, 8), (3, 27)}
f-1 = {(0, 0), (1, 1), (8, 2), (27, 3)}
Domain of f-1 = {0, 1, 8, 27}
संबंधित प्रश्न
Check the injectivity and surjectivity of the following function:
f: N → N given by f(x) = x3
Following the case, state whether the function is one-one, onto, or bijective. Justify your answer.
f : R → R defined by f(x) = 3 − 4x
Let A and B be sets. Show that f: A × B → B × A such that (a, b) = (b, a) is bijective function.
Give an example of a function which is one-one but not onto ?
Classify the following function as injection, surjection or bijection :
f : R → R, defined by f(x) = x3 − x
Classify the following function as injection, surjection or bijection :
f : Q − {3} → Q, defined by `f (x) = (2x +3)/(x-3)`
Find the number of all onto functions from the set A = {1, 2, 3, ..., n} to itself.
Give examples of two surjective functions f1 and f2 from Z to Z such that f1 + f2 is not surjective.
Let f = {(1, −1), (4, −2), (9, −3), (16, 4)} and g = {(−1, −2), (−2, −4), (−3, −6), (4, 8)}. Show that gof is defined while fog is not defined. Also, find gof.
Let R+ be the set of all non-negative real numbers. If f : R+ → R+ and g : R+ → R+ are defined as `f(x)=x^2` and `g(x)=+sqrtx` , find fog and gof. Are they equal functions ?
Give examples of two functions f : N → N and g : N → N, such that gof is onto but f is not onto.
If f : A → B and g : B → C are onto functions, show that gof is a onto function.
Find fog and gof if : f(x) = sin−1 x, g(x) = x2
Find fog and gof if : f(x)= x + 1, g (x) = 2x + 3 .
If f(x) = sin x and g(x) = 2x be two real functions, then describe gof and fog. Are these equal functions?
if f (x) = `sqrt (x +3) and g (x) = x ^2 + 1` be two real functions, then find fog and gof.
Let f be a real function given by f (x)=`sqrt (x-2)`
Find each of the following:
(i) fof
(ii) fofof
(iii) (fofof) (38)
(iv) f2
Also, show that fof ≠ `f^2` .
State with reason whether the following functions have inverse:
h : {2, 3, 4, 5} → {7, 9, 11, 13} with h = {(2, 7), (3, 9), (4, 11), (5, 13)}
Let f : [−1, ∞) → [−1, ∞) be given by f(x) = (x + 1)2 − 1, x ≥ −1. Show that f is invertible. Also, find the set S = {x : f(x) = f−1 (x)}.
Let A = {x &epsis; R | −1 ≤ x ≤ 1} and let f : A → A, g : A → A be two functions defined by f(x) = x2 and g(x) = sin (π x/2). Show that g−1 exists but f−1 does not exist. Also, find g−1.
Write the total number of one-one functions from set A = {1, 2, 3, 4} to set B = {a, b, c}.
Let f be an invertible real function. Write ( f-1 of ) (1) + ( f-1 of ) (2) +..... +( f-1 of ) (100 )
If f(x) = x + 7 and g(x) = x − 7, x ∈ R, write fog (7).
Let f : R → R be the function defined by f(x) = 4x − 3 for all x ∈ R Then write f . [NCERT EXEMPLAR]
Which one the following relations on A = {1, 2, 3} is a function?
f = {(1, 3), (2, 3), (3, 2)}, g = {(1, 2), (1, 3), (3, 1)} [NCERT EXEMPLAR]
Let A = {a, b, c, d} and f : A → A be given by f = {( a,b ),( b , d ),( c , a ) , ( d , c )} write `f^-1`. [NCERT EXEMPLAR]
If a function g = {(1, 1), (2, 3), (3, 5), (4, 7)} is described by g(x) = \[\alpha x + \beta\] then find the values of \[\alpha\] and \[ \beta\] . [NCERT EXEMPLAR]
Let\[A = \left\{ x \in R : - 1 \leq x \leq 1 \right\} = \text{B and C} = \left\{ x \in R : x \geq 0 \right\} and\]\[S = \left\{ \left( x, y \right) \in A \times B : x^2 + y^2 = 1 \right\} \text{and } S_0 = \left\{ \left( x, y \right) \in A \times C : x^2 + y^2 = 1 \right\}\]
Then,
The function
\[f : R \to R\] defined by\[f\left( x \right) = \left( x - 1 \right) \left( x - 2 \right) \left( x - 3 \right)\]
(a) one-one but not onto
(b) onto but not one-one
(c) both one and onto
(d) neither one-one nor onto
Let
\[f : R \to R\] be a function defined by
\[f : R \to R\] is defined by
\[f\left( x \right) = \frac{e^{x^2} - e^{- x^2}}{e^{x^2 + e^{- x^2}}} is\]
The function
If \[g \left( f \left( x \right) \right) = \left| \sin x \right| \text{and} f \left( g \left( x \right) \right) = \left( \sin \sqrt{x} \right)^2 , \text{then}\]
If \[f : R \to \left( - 1, 1 \right)\] is defined by
\[f\left( x \right) = \frac{- x|x|}{1 + x^2}, \text{ then } f^{- 1} \left( x \right)\] equals
If \[g\left( x \right) = x^2 + x - 2\text{ and} \frac{1}{2} gof\left( x \right) = 2 x^2 - 5x + 2\] is equal to
Mark the correct alternative in the following question:
If the set A contains 5 elements and the set B contains 6 elements, then the number of one-one and onto mappings from A to B is
Let the function f: R → R be defined by f(x) = 4x – 1, ∀ x ∈ R. Then, show that f is one-one.
Let f: R → R be the function defined by f(x) = 4x – 3 ∀ x ∈ R. Then write f–1
The domain of the function f: R → R defined by f(x) = `sqrt(x^2 - 3x + 2)` is ______
Let D be the domain of the real valued function f defined by f(x) = `sqrt(25 - x^2)`. Then, write D
Are the following set of ordered pairs functions? If so, examine whether the mapping is injective or surjective.
{(x, y): x is a person, y is the mother of x}
Are the following set of ordered pairs functions? If so, examine whether the mapping is injective or surjective.
{(a, b): a is a person, b is an ancestor of a}
Let X = {1, 2, 3}and Y = {4, 5}. Find whether the following subset of X ×Y are function from X to Y or not
f = {(1, 4), (1, 5), (2, 4), (3, 5)}
Let X = {1, 2, 3}and Y = {4, 5}. Find whether the following subset of X ×Y are function from X to Y or not
g = {(1, 4), (2, 4), (3, 4)}
Let A = [–1, 1]. Then, discuss whether the following functions defined on A are one-one, onto or bijective:
k(x) = x2
Let f: R → R be defined by f(x) = `1/x` ∀ x ∈ R. Then f is ______.
Let f : R → R be defind by f(x) = `1/"x" AA "x" in "R".` Then f is ____________.
The domain of the function `"f"("x") = 1/(sqrt ({"sin x"} + {"sin" ( pi + "x")}))` where {.} denotes fractional part, is
Range of `"f"("x") = sqrt((1 - "cos x") sqrt ((1 - "cos x")sqrt ((1 - "cos x")....infty))`
If f: R → R given by f(x) =(3 − x3)1/3, find f0f(x)
Let the function f: R → R be defined by f(x) = 4x – 1, ∀ x ∈ R then 'f' is
Consider a function f: `[0, pi/2] ->` R, given by f(x) = sinx and `g[0, pi/2] ->` R given by g(x) = cosx then f and g are
If log102 = 0.3010.log103 = 0.4771 then the number of ciphers after decimal before a significant figure comes in `(5/3)^-100` is ______.
Let x is a real number such that are functions involved are well defined then the value of `lim_(t→0)[max{(sin^-1 x/3 + cos^-1 x/3)^2, min(x^2 + 4x + 7)}]((sin^-1t)/t)` where [.] is greatest integer function and all other brackets are usual brackets.
The graph of the function y = f(x) is symmetrical about the line x = 2, then ______.
If A = {x ∈ R: |x – 2| > 1}, B = `{x ∈ R : sqrt(x^2 - 3) > 1}`, C = {x ∈ R : |x – 4| ≥ 2} and Z is the set of all integers, then the number of subsets of the set (A ∩ B ∩ C) C ∩ Z is ______.
Let S = {1, 2, 3, 4, 5, 6, 7}. Then the number of possible functions f: S `rightarrow` S such that f(m.n) = f(m).f(n) for every m, n ∈ S and m.n ∈ S is equal to ______.
Let A = {1, 2, 3, ..., 10} and f : A `rightarrow` A be defined as
f(k) = `{{:(k + 1, if k "is odd"),( k, if k "is even"):}`.
Then the number of possible functions g : A `rightarrow` A such that gof = f is ______.
Let f(x) be a polynomial function of degree 6 such that `d/dx (f(x))` = (x – 1)3 (x – 3)2, then
Assertion (A): f(x) has a minimum at x = 1.
Reason (R): When `d/dx (f(x)) < 0, ∀ x ∈ (a - h, a)` and `d/dx (f(x)) > 0, ∀ x ∈ (a, a + h)`; where 'h' is an infinitesimally small positive quantity, then f(x) has a minimum at x = a, provided f(x) is continuous at x = a.
Which one of the following graphs is a function of x?
 |
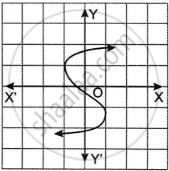 |
| Graph A | Graph B |
If f : R `rightarrow` R is defined by `f(x) = (2x - 7)/4`, show that f(x) is one-one and onto.
The trigonometric equation tan–1x = 3tan–1 a has solution for ______.
