Advertisements
Advertisements
प्रश्न
A horse is tethered to one corner of a field which is in the shape of an equilateral triangle of side 12 m. If the length of the rope is 7 m, find the area of the field which the horse cannot graze. Write the answer correct to 2 places of decimal.
उत्तर
Side of the equilateral triangle = 12 m
Area of the equilateral triangle `= sqrt(3)/4xx("Side")^2`
`=sqrt(3)/4xx12xx12`
Length of the rope = 7 m
Area of the field the horse can graze is the area of the sector of radius 7 m .Also, the angle subtended at the centre is 60°
`=theta/360xxpi"r"^2`
`=60/360xx22/7xx(7)^2`
= 25.67 m2
Area of the field the horse cannot graze = Area of the equilateral triangle -- Area of the field the horse can graze
= 62.28 - 25.67 = 36.61 m2
APPEARS IN
संबंधित प्रश्न
Find the area and perimeter of an isosceles right angled triangle, each of whose equal sides measure 10cm.
In a circle of radius 21 cm, an arc subtends an angle of 60° at the centre. Find the area of the sector formed by the arc. (Use π = `22/7`)
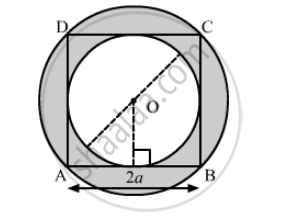
In the following figure, ABCD is a square of side 2a, Find the ratio between
(i) the circumferences
(ii) the areas of the in circle and the circum-circle of the square.
Area of the largest triangle that can be inscribed in a semi-circle of radius r units is ______.
What is the diameter of a circle whose area is equal to the sum of the areas of two circles of diameters 10 cm and 24 cm?
Find the lengths of the arcs cut off from a circle of radius 12 cm by a chord 12 cm long. Also, find the area of the minor segment.
In the given figure, an equilateral triangle has been inscribed in a circle of radius 4 cm. Find the area of the shaded region.

The minute hand of a clock is 8 cm long. Find the area swept by the minute hand between 8.30 a.m. and 9.05 a.m.
There is a path of uniform width 7 m round and outside a circular garden of diameter 210 m. Find the area of the path.
If the radius of a circle is tripled, the area becomes ______.
