Advertisements
Advertisements
प्रश्न
A person normally weighing 50 kg stands on a massless platform which oscillates up and down harmonically at a frequency of 2.0 s–1 and an amplitude 5.0 cm. A weighing machine on the platform gives the persons weight against time.
- Will there be any change in weight of the body, during the oscillation?
- If answer to part (a) is yes, what will be the maximum and minimum reading in the machine and at which position?
Advertisements
उत्तर
In this case, acceleration is variable. In accelerated motion, the weight of body depends on the magnitude and direction of acceleration for upward or downward motion.
a. Hence, the weight of the body changes during oscillations
b. Considering the situation in two extreme positions. as their acceleration is maximum in magnitude.
We have, mg – N = ma
∵ At the highest point, the platform is accelerating downward.
⇒ N = mg – ma
But a = ω2A .....[In magnitude]
∴ N = mg – mω2A
Where, A = amplitude of motion
Given, m = 50 kg, frequency v = 2 s–1
∴ ω = 2πv = 4π rad/s
A = 5 cm = 5 × 10–2 m
∴ N = 50 × 9.8 – 50 × (4π)2 × 5×10–2
= 50[9.8 – 16π2 × 5 × 10–2]
= 50[9.8 – 7.89]
= 50 × 1.91
= 95.5 N
When the platform is at the lowest position of its oscillation,
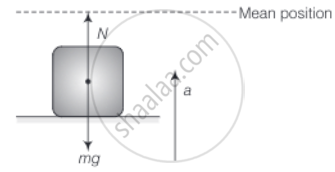
It is accelerating towards the mean position that is vertically upwards.
Writing the equation of motion
N – mg = ma = mω2A
or N = mg + mω2A
= m[g + ω2A]
Putting the data N = 50[9.8 + (4π)2 × 5 × 10–2]
= 50[9.8 + (12.56)2 × 5 × 10–2]
= 50[9.8 + 7.88]
= 50 × 17.68
= 884 N
Now, the machine reads the normal reaction. It is clear that
Maximum weight = 884 N ......(At lowest point)
Minimum weight = 955 N .....(At top point)
APPEARS IN
संबंधित प्रश्न
A copper metal cube has each side of length 1 m. The bottom edge of the cube is fixed and tangential force 4.2x108 N is applied to a top surface. Calculate the lateral displacement of the top surface if modulus of rigidity of copper is 14x1010 N/m2.
Which of the following example represent (nearly) simple harmonic motion and which represent periodic but not simple harmonic motion?
General vibrations of a polyatomic molecule about its equilibrium position.
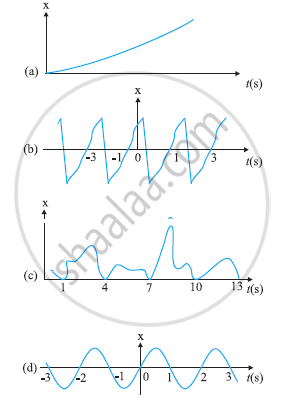
Figure depicts four x-t plots for linear motion of a particle. Which of the plots represent periodic motion? What is the period of motion (in case of periodic motion)?
The length of the second’s pendulum in a clock is increased to 4 times its initial length. Calculate the number of oscillations completed by the new pendulum in one minute.
Two bodies A and B of equal mass are suspended from two separate massless springs of spring constant k1 and k2 respectively. If the bodies oscillate vertically such that their maximum velocities are equal, the ratio of the amplitude of A to that of B is
The position, velocity and acceleration of a particle executing simple harmonic motion are found to have magnitude 2 cm, 1 m s−1 and 10 m s−2 at a certain instant. Find the amplitude and the time period of the motion.
Consider a simple harmonic motion of time period T. Calculate the time taken for the displacement to change value from half the amplitude to the amplitude.
A small block of mass m is kept on a bigger block of mass M which is attached to a vertical spring of spring constant k as shown in the figure. The system oscillates vertically. (a) Find the resultant force on the smaller block when it is displaced through a distance x above its equilibrium position. (b) Find the normal force on the smaller block at this position. When is this force smallest in magnitude? (c) What can be the maximum amplitude with which the two blocks may oscillate together?
A particle of mass m is attatched to three springs A, B and C of equal force constants kas shown in figure . If the particle is pushed slightly against the spring C and released, find the time period of oscillation.
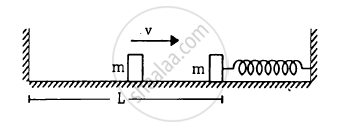
The left block in figure moves at a speed v towards the right block placed in equilibrium. All collisions to take place are elastic and the surfaces are frictionless. Show that the motions of the two blocks are periodic. Find the time period of these periodic motions. Neglect the widths of the blocks.
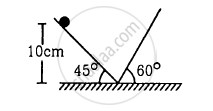
Find the time period of the motion of the particle shown in figure . Neglect the small effect of the bend near the bottom.
A body of mass 1 kg is mafe to oscillate on a spring of force constant 16 N/m. Calculate (a) Angular frequency, (b) Frequency of vibrations.
A 20 cm wide thin circular disc of mass 200 g is suspended to rigid support from a thin metallic string. By holding the rim of the disc, the string is twisted through 60° and released. It now performs angular oscillations of period 1 second. Calculate the maximum restoring torque generated in the string under undamped conditions. (π3 ≈ 31)
The maximum speed of a particle executing S.H.M. is 10 m/s and maximum acceleration is 31.4 m/s2. Its periodic time is ______
Which of the following example represent (nearly) simple harmonic motion and which represent periodic but not simple harmonic motion?
The rotation of the earth about its axis.
Which of the following example represent (nearly) simple harmonic motion and which represent periodic but not simple harmonic motion?
A motion of an oscillating mercury column in a U-tube.
The displacement time graph of a particle executing S.H.M. is shown in figure. Which of the following statement is/are true?
- The force is zero at `t = (T)/4`.
- The acceleration is maximum at `t = (4T)/4`.
- The velocity is maximum at `t = T/4`.
- The P.E. is equal to K.E. of oscillation at `t = T/2`.
When a particle executes Simple Harmonic Motion, the nature of the graph of velocity as a function of displacement will be ______.
A particle performs simple harmonic motion with a period of 2 seconds. The time taken by the particle to cover a displacement equal to half of its amplitude from the mean position is `1/a` s. The value of 'a' to the nearest integer is ______.
