Advertisements
Advertisements
प्रश्न
A person standing on the bank of a river observes that the angle of elevation of the top of a tree standing on the opposite bank is 60°. When he moves 40 m away from the bank, he finds the angle of elevation to be 30°. Find:
- the height of the tree, correct to 2 decimal places,
- the width of the river.
उत्तर
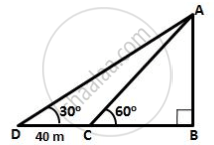
Let AB be the tree of height 'h' m and BC be the width of the river.
Let D be the point on the opposite bank of tree such that CD = 40 m.
Here ∠ADB = 30° and ∠ACB = 60°
Let speed of the boat be 'x' metre per minute.
In ΔABC,
`(AB)/(BC) = tan 60^circ = sqrt(3) `
`=> h/(BC) = sqrt(3)`
`=> h = BC sqrt(3)`
In ΔADB,
`(AB)/(BD) = tan 30^circ`
`=> h/(40 + BC) = 1/sqrt(3)`
`=> (BC sqrt(3))/(40 + BC) = 1/sqrt(3)`
`=> BC sqrt(3) * sqrt(3) = 40 + BC`
`=>` 3BC = 40 + BC
`=>` 3BC – BC = 40
`=>` 2BC = 40 m
`=> BC = 40/2 m`
`=>` BC = 20 m
∴ h = 20 × 1.732 = 34.64 m
Hence, height of the tree is 34.64 m and width of the river is 20 m.
APPEARS IN
संबंधित प्रश्न
An aeroplane at an altitude of 250 m observes the angle of depression of two boats on the opposite banks of a river to be 45° and 60° respectively. Find the width of the river. Write the answer correct to the nearest whole number.
The angle of elevation of the top of a tower is observed to be 60°. At a point, 30 m vertically above the first point of observation, the elevation is found to be 45°. Find:
- the height of the tower,
- its horizontal distance from the points of observation.
From the top of a cliff, 60 metres high, the angles of depression of the top and bottom of a tower are observed to be 30° and 60°. Find the height of the tower.
A man in a boat rowing away from a lighthouse 150 m high, takes 2 minutes to change the angle of elevation of the top of the lighthouse from 60° to 45°. Find the speed of the boat.
A vertical tower stand on horizontel plane and is surmounted by a vertical flagstaff of height h metre. At a point on the plane, the angle of elevation of the bottom of the flagstaff is α and that of the top of flagstaff is β. Prove that the height of the tower is
`(h tan alpha)/(tan beta - tan alpha)`
The length of the shadow of a statue increases by 8m, when the latitude of the sun changes from 45° to 30°. Calculate the height of the tower.
A vertical pole and a vertical tower are on the same level ground in such a way that from the top of the pole, the angle of elevation of the top of the tower is 60o and the angle of depression of the bottom of the tower is 30o. Find: the height of the pole, if the height of the tower is 75 m.
In figures, find the length CF.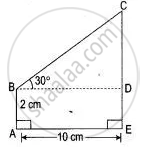
A man is standing on the deck of a ship, which is 10 m above water level. He observes the angle of elevation of the top of a hill as 60° and the angle of depression of the base of the hill as 30°. Calculate the distance of the hill from the ship and the height of the hill.
Vertical tower is 20m high. A man standing at some distance from the tower knows that the cosine of the angle of elevation of the top of the tower is 0.53. How far is he standing from the foot of the tower?
