Question

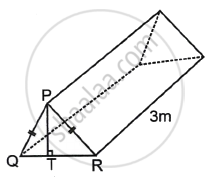
A tent is a shape of a triangular 'prism' resting on a rectangular side PQ = PR, PT = 1.5 m, QR = 1.8 m, length of the tent = 3 m. Find:

1. ∠PQR
2. The volume of the tent

Answer 1

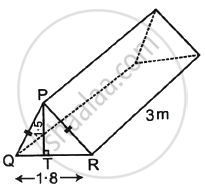
Given: QR = 1.8 m, PT = 1.5 m, PQ = PR.

Since, PQ = PR

So, ΔPQR is an isosceles triangle.

So, PT bisects QR.

∴ QT = ${\displaystyle \frac{1.8}{2}}$ = 0.9

i. tan Q = ${\displaystyle \frac{\text{Perpendicular}}{\text{Base}}}$

tan Q = ${\displaystyle \frac{1.5}{0.9}}$

tan Q = 1.6666666667

⇒ Q = 59.036°

Hence, ∠PQR = 59.036°.

ii. Volume of the tent,

V = ${\displaystyle \frac{1}{2}}$ LWH

Here, L = 3 m, W = 1.8 m and H = 1.5 m

∴ V = ${\displaystyle \frac{1}{2}\times 3\times 1.8\times 1.5}$ = 4.05 m³

As a result, the volume of the tent is 4.05 m³.