Question

A three-wheeler starts from rest, accelerates uniformly with 1 m s^–2 on a straight road for 10 s, and then moves with uniform velocity. Plot the distance covered by the vehicle during the n^th second (n = 1,2,3….) versus n. What do you expect this plot to be during accelerated motion: a straight line or a parabola?

Answer 1

Straight line

Distance covered by a body in n^th second is given by the relation

`D_n = u + a/2 (2n -1)`  ....(i)

Where,

u = Initial velocity

a = Acceleration

n = Time = 1, 2, 3, ..... ,n

In the given case,

u = 0 and a = 1 m/s²

^{`:.D_n = 1/2(2n-1)`  ....(ii)}

This relation shows that:

D_n ∝ n … (iii)

Now, substituting different values of n in equation (iii), we get the following table:

n	1	2	3	4	5	6	7	8	9	10
Dn	0.5	1.5	2.5	3.5	4.5	5.5	6.5	7.5	8.5	9.5

The plot between n and D_n will be a straight line as shown:

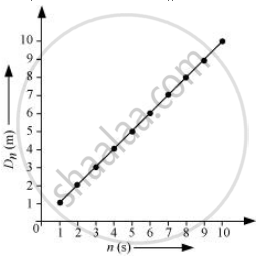
Since the given three-wheeler acquires uniform velocity after 10 s, the line will be parallel to the time-axis after n = 10 s

Answer 2

Since `S_(n^(th)) = u+ 1/2a(2n-1)`

when u = 0, a = 1 `ms^(-2)`

`:.S_(n^(th)) = 0 + 1/2(2n-1) = 1/2(2n-1)`

`:. For n = 1,2,3....`

`S_1 = 1/2(2xx1-1) = 0.5 m`

`S_2 = 1/2(2xx2-1) = 1.5 m`

`S_3 = 1/2 (2xx3-1) = 2.5 m`

`S_4 = 1/2(2xx4-1)= 3.5 m`

`S_5 = 1/2(2xx5-1) = 4.5 m`

`S_6 = 1/2(2xx6-1) = 5.5 m`

`S_7 = 1/2(2xx7-1) = 6.5 m`

`S_8 = 1/2(2xx8-1) = 7.5 m`

`S_9 = 1/2(2xx9-1) = 8.5m`

`S_10 =1/2 (2xx10-1)= 9.5 m`