Advertisements
Advertisements
प्रश्न
ABC is a right triangle with angle B = 90º. A circle with BC as diameter meets by hypotenuse AC at point D. Prove that: BD2 = AD × DC.
उत्तर
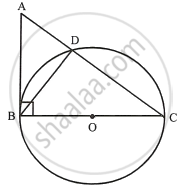
In ΔADB,
∠D = 90°
∴ ∠A + ∠ABD = 90° ...(i)
But in ΔABC, ∠B = 90°
∴ ∠A + ∠C = 90° ...(ii)
From (i) and (ii)
∠C = ∠ABD
Now in ΔABD and ΔCBD
∠BDA = ∠BDA = 90°
∠ABD = ∠BCD
∴ ΔABD ∼ ΔCBD ...(AA postulate)
∴ `(BD)/(DC) = (AD)/(BD)`
`=>` BD2 = AD × DC
APPEARS IN
संबंधित प्रश्न
A line intersecting a circle in two points is called a ______.
A circle can have ______ parallel tangents at the most.
The given figure shows a circle with centre O and BCD is tangent to it at C. Show that : ∠ACD + ∠BAC = 90°.

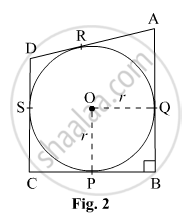
In Fig.2, a circle with centre O is inscribed in a quadrilateral ABCD such that, it touches the sides BC, AB, AD and CD at points P, Q, R and S respectively, If AB = 29 cm, AD = 23 cm, ∠B = 90° and DS = 5 cm, then the radius of the circle (in cm.) is:
Out of the two concentric circles, the radius of the outer circle is 5 cm and the chord AC of length 8 cm is a tangent to the inner circle. Find the radius of the inner circle.
A chord of a length 16.8 cm is at a distance of 11.2 cm from the centre of a circle . Find the length of the chord of the same circle which is at a distance of 8.4 cm from the centre.
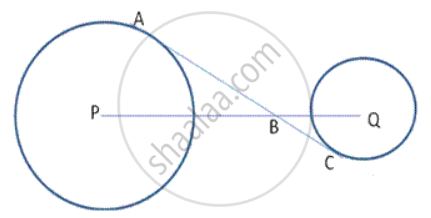
In the following fig. , AC is a transversal common tangent to tvvo circles with centres P and Q and of radii 6cm and 3cm respectively. Given that AB = 8cm, calculate PQ.
In figure, AT is a tangent to the circle with centre O such that OT = 4 cm and ∠OTA = 30°. Then AT is equal to ______.

In the adjoining figure, PT is a tangent at T to the circle with centre O. If ∠TPO = 30°, find the value of x.

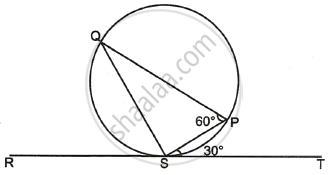
In the given diagram RT is a tangent touching the circle at S. If ∠PST = 30° and ∠SPQ = 60°, then ∠PSQ is equal to ______.