Advertisements
Advertisements
प्रश्न
ABCD is a trapezium with AD ∥ BC and AD = 4 cm. If the diagonals AC and BD intersect each other at O such that AO/OC = DO/OB = 1/2, then BC = ______.
विकल्प
6 cm
7 cm
8 cm
9 cm
उत्तर
ABCD is a trapezium with AD ∥ BC and AD = 4 cm. If the diagonals AC and BD intersect each other at O such that AO/OC = DO/OB = 1/2, then BC = 8 cm.
Explanation:
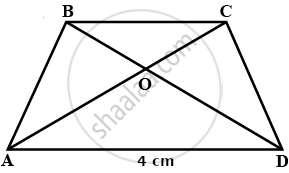
In ΔAOD and ΔBOC
∠AOD = ∠BOC .....(Vertically opposite angle)
`(AO)/(OC) = (DO)/(OB)` ......(Given)
∴ ΔAOD ∼ΔBOC ......(SAS similarity)
Since both triangles are similar.
Their sides will be in proportion
`(AO)/(OC) = (DO)/(OB) = (AD)/(BC)`
`1/2 = (AD)/(BC)`
Putting AD = 4 cm
`1/2 = 4/(BC)`
BC = 2 × 4
BC = 8 cm
APPEARS IN
संबंधित प्रश्न
State which pair of triangles in the following figure are similar. Write the similarity criterion used by you for answering the question, and also write the pairs of similar triangles in the symbolic form:
E is a point on the side AD produced of a parallelogram ABCD and BE intersects CD at F. Show that ΔABE ∼ ΔCFB.
ABCD is a parallelogram and APQ is a straight line meeting BC at P and DC produced at Q. Prove that the rectangle obtained by BP and DQ is equal to the AB and BC.
The sides of certain triangles are given below. Determine which of them right triangles are.
(a – 1) cm, `2sqrta` cm, (a + 1) cm
The corresponding sides of two similar triangles are in the ratio 2 : 3. If the area of the smaller triangle is 48 cm2, find the area of the larger triangle.
In figure, BD and CE intersect each other at the point P. Is ΔPBC ~ ΔPDE? Why?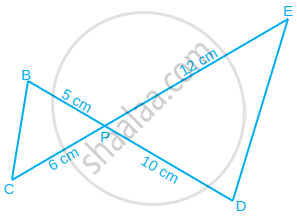
If in two triangles ABC and PQR, `(AB)/(QR) = (BC)/(PR) = (CA)/(PQ)`, then ______.
In figure, if ∠D = ∠C, then it is true that ΔADE ~ ΔACB? Why?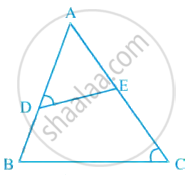
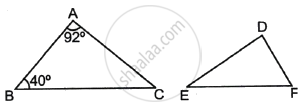
If ΔABC ∼ ΔDEF such that ∠A = 92° and ∠B = 40°, then ∠F = ?
If in two right triangles, one of the acute angles of one triangle is equal to an acute angle of the other triangle, can you say that the two triangles will be similar? Why?
