Advertisements
Advertisements
प्रश्न
Answer the following question.
Derive the relation between three coefficients of thermal expansion.
उत्तर
- Consider a square plate of side l0 at 0° C and lT at T °C.
∴ lT = l0 (1 + αT)
If area of plate at 0° C is A0, A0 = `l_0^2`
If area of plate at T °C is AT,
`"A"_"T" = l_1^2 = l_0^2 (1 +alpha"T")^2`
or `"A"_"T" = "A"_0(1 + alpha"T")^2` ....(1)
Also,
`"A"_"T" = "A"_0(1 + beta"T")` ....(2) `[because beta = ("A"_"r" - "A"_0)/("A"_0("T" - "T"_0))]` - Using Equations (1) and (2),
`"A"_0(1 + alpha"T")^2 = "A"_0(1 + beta"T")`
∴ `1 + 2alpha"T" + alpha^2"T"^2 = 1 + beta"T"` - Since the values of α are very small, the term α2T2 is very small and may be neglected.
∴ β = 2α - The result is general because any solid can be regarded as a collection of small squares.
- Consider a cube of side l0 at 0 °C and lT at T °C.
∴ lT = l0(1 + αT)
If volume of the cube at 0 °C is V0, V0 = `l_0^3`
If volume of the cube at T °C is
`"V"_"T", "V"_"T" = l_"T"^3 = l_0^3 (1 + alpha"T")^3`
`"V"_"T" = "V"_0(1 + alpha"T")^3` ....(1)
Also,
`"V"_"T" = "V"_0(1 + gamma"T")` ....(2) ...`[therefore gamma = ("V"_"T" - "V"_0)/("V"_0("T" - "T"_0))]` - Using Equations (1) and (2),
`"V"_0 (1 + alpha"T")^3 = "V"_0(1 + gamma"T")`
∴ `1 + 3alpha"T" + 3alpha^2"T"^2 + alpha^3"T"^3 = 1 + gamma"T"` - Since the values of α are very small, the terms with higher powers of α may be neglected.
∴ γ = 3α - The result is general because any solid can be regarded as a collection of small cubes.
- Relation between α, β and γ is given by,
`alpha = beta/2 = gamma/3`
where, α = coefficient of linear expansion,
β = coefficient of superficial expansion,
γ = coefficient of cubical expansion.
APPEARS IN
संबंधित प्रश्न
If two bodies are in thermal equilibrium in one frame, will they be in thermal equilibrium in all frames?
If mercury and glass had equal coefficients of volume expansion, could we make a mercury thermometer in a glass tube?
If an automobile engine is overheated, it is cooled by pouring water on it. It is advised that the water should be poured slowly with the engine running. Explain the reason.
Show that the moment of inertia of a solid body of any shape changes with temperature as I = I0 (1 + 2αθ), where I0 is the moment of inertia at 0°C and α is the coefficient of linear expansion of the solid.
Answer the following question.
What is thermal stress?
Solve the following problem.
In olden days, while laying the rails for trains, small gaps used to be left between the rail sections to allow for thermal expansion. Suppose the rails are laid at room temperature 27 °C. If maximum temperature in the region is 45 °C and the length of each rail section is 10 m, what should be the gap left given that α = 1.2 × 10–5K–1 for the material of the rail section?
Solve the following problem.
A blacksmith fixes iron ring on the rim of the wooden wheel of a bullock cart. The diameter of the wooden rim and the iron ring are 1.5 m and 1.47 m respectively at room temperature of 27 °C. To what temperature the iron ring should be heated so that it can fit the rim of the wheel? (αiron = 1.2 × 10–5K–1).
A clock pendulum having coefficient of linear expansion. α = 9 × 10-7/°C-1 has a period of 0.5 s at 20°C. If the clock is used in a climate, where the temperature is 30°C, how much time does the clock lose in each oscillation? (g = constant)
A metre scale made of a metal reads accurately at 25 °C. Suppose in an experiment an accuracy of 0.12 mm in 1 m is required, the range of temperature in which the experiment can be performed with this metre scale is ______.(coefficient of linear expansion of the metal is `20 xx 10^-6 / (°"C")`
A metal sphere 10.01 cm in diameter is placed on a brass ring of internal diameter 10 cm and at the same temperature of 12° C. The temperature up to which they should be heated together so that the metal sphere just passes through the ring is `[alpha_"metal"= 12 xx 10^-6//°"C" and alpha_"brass" =18 xx 10^-6//°"C"]` ____________.
A hot body at a temperature 'T' is kept in a surrounding of temperature 'T0'. It takes time 't1' to cool from 'T' to 'T2', time t2 to cool from 'T2' to 'T3' and time 't3' to cool from 'T3' to 'T4'. If (T - T2) = (T2 - T3) = (T3 - T4), then ______.
A metal rod of cross-sectional area 3 × 10-6 m2 is suspended vertically from one end has a length 0.4 m at 100°C. Now the rod is cooled upto 0°C, but prevented from contracting by attaching a mass 'm' at the lower end. The value of 'm' is ______.
(Y = 1011 N/m2, coefficient of linear expansion = 10-5/K, g = 10m/s2)
A metal rod of length Land cross-sectional area A is heated through T °C. What is the force required to prevent the expansion of the rod lengthwise?
(Y = Young's modulus of material of the rod, α = coefficient of linear expansion of the rod.)
The volume of a metal block changes by 0.86% when heated through 200 °C then its coefficient of cubical expansion is ______.
As the temperature is increased, the time period of a pendulum ______.
The radius of a metal sphere at room temperature T is R, and the coefficient of linear expansion of the metal is α. The sphere is heated a little by a temperature ∆T so that its new temperature is T + ∆T. The increase in the volume of the sphere is approximately ______.
Find out the increase in moment of inertia I of a uniform rod (coefficient of linear expansion α) about its perpendicular bisector when its temperature is slightly increased by ∆T.
Calculate the stress developed inside a tooth cavity filled with copper when hot tea at temperature of 57°C is drunk. You can take body (tooth) temperature to be 37°C and α = 1.7 × 10–5/°C, bulk modulus for copper = 140 × 109 N/m2.
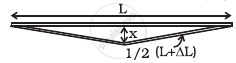
A rail track made of steel having length 10 m is clamped on a raillway line at its two ends (figure). On a summer day due to rise in temperature by 20° C, it is deformed as shown in figure. Find x (displacement of the centre) if αsteel = 1.2 × 10–5/°C.
At what temperature a gold ring of diameter 6.230 cm be heated so that it can be fitted on a wooden bangle of diameter 6.241 cm? Both diameters have been measured at room temperature (27°C). (Given: coefficient of linear thermal expansion of gold αL = 1.4 × 10-5 K-1).
The height of mercury column measured with brass scale at temperature T0 is H0. What height H' will the mercury column have at T = 0°C. Coefficient of volume expansion of mercury is γ. Coefficient of linear expansion of brass is α ______.
A solid metallic cube having a total surface area of 24 m2 is uniformly heated. If its temperature is increased by 10°C, calculate the increase in the volume of the cube.
(Given: α = 5.0 × 10-4°C-1)
A glass flask is filled up to a mark with 50 cc of mercury at 18°C. If the flask and contents are heated to 38°C, how much mercury will be above the mark? (α for glass is 9 × 10-6/°C and coefficient of real expansion of mercury is 180 × 10-6/°C)
A clock with an iron pendulum keeps the correct time at 15°C. If the room temperature is 20°C, the error in seconds per day will be near ______.
(coefficient of linear expansion of iron is 1.2 × 10-5/°C)
