Advertisements
Advertisements
प्रश्न
Describe the locus of a runner, running around a circular track and always keeping a distance of 1.5 m from the inner edge.
उत्तर

The locus of the runner, running around a circular track and always keeping a distance of 1.5 m from the inner edge will be the circumference of a circle whose radius is equal to the radius of the inner circular track plus 1.5 m.
APPEARS IN
संबंधित प्रश्न
Construct a triangle ABC, in which AB = 4.2 cm, BC = 6.3 cm and AC = 5 cm. Draw perpendicular bisector of BC which meets AC at point D. Prove that D is equidistant from B and C.
The bisectors of ∠B and ∠C of a quadrilateral ABCD intersect each other at point P. Show that P is equidistant from the opposite sides AB and CD.
Draw an angle ABC = 75°. Draw the locus of all the points equidistant from AB and BC.
In the figure given below, find a point P on CD equidistant from points A and B.

Describe the locus of the centre of a wheel of a bicycle going straight along a level road.
Sketch and describe the locus of the vertices of all triangles with a given base and a given altitude.
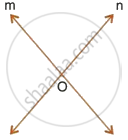
In the given figure, obtain all the points equidistant from lines m and n; and 2.5 cm from O.
In a quadrilateral ABCD, if the perpendicular bisectors of AB and AD meet at P, then prove that BP = DP.
ΔPBC and ΔQBC are two isosceles triangles on the same base BC but on the opposite sides of line BC. Show that PQ bisects BC at right angles.
The bisectors of ∠B and ∠C of a quadrilateral ABCD intersect in P. Show that P is equidistant from the opposite sides AB and CD.
