Advertisements
Advertisements
प्रश्न
The bisectors of ∠B and ∠C of a quadrilateral ABCD intersect in P. Show that P is equidistant from the opposite sides AB and CD.
उत्तर
Given: A quadrilateral ABCD in which bisectors of ∠B and ∠C meet in P. PM ⊥ ABand PN ⊥ CD.
To prove: PM = PN
Construction: Draw PL ⊥ BC
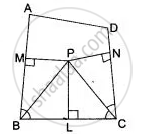
Proof: Since, P lies on the bisector of ∠B
∴ P is equidistant from BC and BA
⇒ PL = PM ...(i)
Also, P lies on the bisector of ∠C ...[Given]
∴ P is equidistant from CB and CD
⇒ PL = PN ...(ii)
From (i) and (ii), we have
PL = PM
and PL = PN
⇒ PM = PN.
Hence proved.
APPEARS IN
संबंधित प्रश्न
In each of the given figures; PA = PB and QA = QB.
| i. | 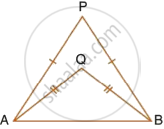 |
| ii. | 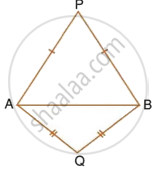 |
Prove, in each case, that PQ (produce, if required) is perpendicular bisector of AB. Hence, state the locus of the points equidistant from two given fixed points.
Construct a right angled triangle PQR, in which ∠Q = 90°, hypotenuse PR = 8 cm and QR = 4.5 cm. Draw bisector of angle PQR and let it meets PR at point T. Prove that T is equidistant from PQ and QR.
Describe the locus of the centre of a wheel of a bicycle going straight along a level road.
Describe the locus of the door handle, as the door opens.
Describe the locus of a point in rhombus ABCD, so that it is equidistant from
- AB and BC;
- B and D.
By actual drawing obtain the points equidistant from lines m and n; and 6 cm from a point P, where P is 2 cm above m, m is parallel to n and m is 6 cm above n.
Prove that the common chord of two intersecting circles is bisected at right angles by the line of centres.
Show that the locus of the centres of all circles passing through two given points A and B, is the perpendicular bisector of the line segment AB.
ΔPBC, ΔQBC and ΔRBC are three isosceles triangles on the same base BC. Show that P, Q and R are collinear.
Use ruler and compasses for the following question taking a scale of 10 m = 1 cm. A park in a city is bounded by straight fences AB, BC, CD and DA. Given that AB = 50 m, BC = 63 m, ∠ABC = 75°. D is a point equidistant from the fences AB and BC. If ∠BAD = 90°, construct the outline of the park ABCD. Also locate a point P on the line BD for the flag post which is equidistant from the corners of the park A and B.
