Advertisements
Advertisements
प्रश्न
ΔPBC, ΔQBC and ΔRBC are three isosceles triangles on the same base BC. Show that P, Q and R are collinear.
उत्तर
Given: Three isosceles triangles PBC, QBC and RBC on the same base BC such that PB = PC, QB = QC and RB = RC.
To prove: P, Q, R are collinear.
Proof: Let l be the perpendicular bisector of BC. Since, the locus of points equidistant from B and C is the perpendicular of the segment joining them. Therefore,
ΔPBC is an isosceles
⇒ PB = PC
⇒ P lies on l ...(i)
ΔQBC is isosceles
⇒ QB = QC
⇒ Q lies on l ...(ii)
ΔRBC is an isosceles
⇒ RB = RC
⇒ R lies on l ...(iii)
From (i), (ii) and (iii), it follows that P, Q and R lie on L.
Hence, P, Q and R are collinear.
Hence proved.
APPEARS IN
संबंधित प्रश्न
Construct a triangle ABC, in which AB = 4.2 cm, BC = 6.3 cm and AC = 5 cm. Draw perpendicular bisector of BC which meets AC at point D. Prove that D is equidistant from B and C.
In each of the given figures; PA = PB and QA = QB.
| i. | 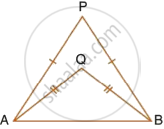 |
| ii. | 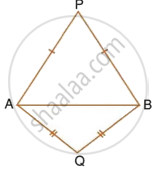 |
Prove, in each case, that PQ (produce, if required) is perpendicular bisector of AB. Hence, state the locus of the points equidistant from two given fixed points.
In parallelogram ABCD, side AB is greater than side BC and P is a point in AC such that PB bisects angle B. Prove that P is equidistant from AB and BC.
Draw an ∠ABC = 60°, having AB = 4.6 cm and BC = 5 cm. Find a point P equidistant from AB and BC; and also equidistant from A and B.
Describe the locus of points at a distance 2 cm from a fixed line.
Describe the locus of the moving end of the minute hand of a clock.
In a quadrilateral PQRS, if the bisectors of ∠ SPQ and ∠ PQR meet at O, prove that O is equidistant from PS and QR.
In a quadrilateral ABCD, if the perpendicular bisectors of AB and AD meet at P, then prove that BP = DP.
Prove that the common chord of two intersecting circles is bisected at right angles by the line of centres.
Given: ∠BAC, a line intersects the arms of ∠BAC in P and Q. How will you locate a point on line segment PQ, which is equidistant from AB and AC? Does such a point always exist?
