Advertisements
Advertisements
Question
ΔPBC, ΔQBC and ΔRBC are three isosceles triangles on the same base BC. Show that P, Q and R are collinear.
Solution
Given: Three isosceles triangles PBC, QBC and RBC on the same base BC such that PB = PC, QB = QC and RB = RC.
To prove: P, Q, R are collinear.
Proof: Let l be the perpendicular bisector of BC. Since, the locus of points equidistant from B and C is the perpendicular of the segment joining them. Therefore,
ΔPBC is an isosceles
⇒ PB = PC
⇒ P lies on l ...(i)
ΔQBC is isosceles
⇒ QB = QC
⇒ Q lies on l ...(ii)
ΔRBC is an isosceles
⇒ RB = RC
⇒ R lies on l ...(iii)
From (i), (ii) and (iii), it follows that P, Q and R lie on L.
Hence, P, Q and R are collinear.
Hence proved.
APPEARS IN
RELATED QUESTIONS
The given figure shows a triangle ABC in which AD bisects angle BAC. EG is perpendicular bisector of side AB which intersects AD at point F.
Prove that:
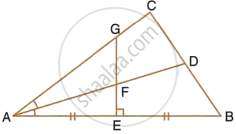
F is equidistant from AB and AC.
Draw an angle ABC = 75°. Draw the locus of all the points equidistant from AB and BC.
Describe the locus of the centre of a wheel of a bicycle going straight along a level road.
Describe the locus of a runner, running around a circular track and always keeping a distance of 1.5 m from the inner edge.
Describe the locus of the centres of all circles passing through two fixed points.
Describe the locus of points at distances greater than or equal to 35 mm from a given point.
By actual drawing obtain the points equidistant from lines m and n; and 6 cm from a point P, where P is 2 cm above m, m is parallel to n and m is 6 cm above n.
In a quadrilateral PQRS, if the bisectors of ∠ SPQ and ∠ PQR meet at O, prove that O is equidistant from PS and QR.
In Fig. ABCD is a quadrilateral in which AB = BC. E is the point of intersection of the right bisectors of AD and CD. Prove that BE bisects ∠ABC.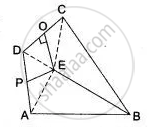
Find the locus of points which are equidistant from three non-collinear points.
