Advertisements
Advertisements
Question
Draw an angle ABC = 75°. Draw the locus of all the points equidistant from AB and BC.
Solution
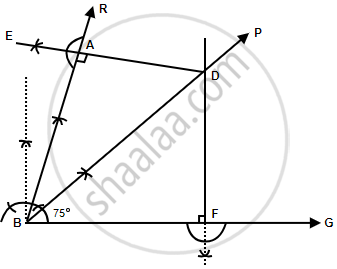
Steps of construction:
- Draw a ray BC.
- Construct a ray RA making an angle of 75° with BC. Therefore, ABC = ABC = 75°
- Draw the angle bisector BP of ∠ABC.
BP is the required locus. - Take any point D on BP.
- From D, draw DE ⊥ AB and DF ⊥ BC.
Since D lies on the angle bisector BP of ∠ABC.
D is equidistant from AB and BC.
Hence, DE = DF
Similarly, any point on BP is equidistant from AB and BC.
Therefore, BP is the locus of all points which are equidistant from AB and BC.
APPEARS IN
RELATED QUESTIONS
Construct a right angled triangle PQR, in which ∠Q = 90°, hypotenuse PR = 8 cm and QR = 4.5 cm. Draw bisector of angle PQR and let it meets PR at point T. Prove that T is equidistant from PQ and QR.
The given figure shows a triangle ABC in which AD bisects angle BAC. EG is perpendicular bisector of side AB which intersects AD at point F.
Prove that:
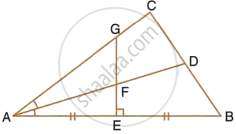
F is equidistant from A and B.
Draw a line AB = 6 cm. Draw the locus of all the points which are equidistant from A and B.
Describe the locus of a runner, running around a circular track and always keeping a distance of 1.5 m from the inner edge.
Describe the locus of the door handle, as the door opens.
Describe the locus of the centres of all circles passing through two fixed points.
Describe the locus of points at distances greater than or equal to 35 mm from a given point.
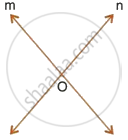
In the given figure, obtain all the points equidistant from lines m and n; and 2.5 cm from O.
Prove that the common chord of two intersecting circles is bisected at right angles by the line of centres.
ΔPBC and ΔQBC are two isosceles triangles on the same base BC but on the opposite sides of line BC. Show that PQ bisects BC at right angles.
