Advertisements
Advertisements
प्रश्न
Determine the number of sides of a polygon whose exterior and interior angles are in the ratio 1 : 5.
उत्तर
\[\text{ Let n be the number of sides of a polygon } . \]
\[\text { Let x and 5x be the exterior and interior angles } . \]
\[\text{ Since the sum of an interior and the corresponding exterior angle is } 180° , \text{ we have } : \]
\[x + 5x = 180° \]
\[ \Rightarrow 6x = 180° \]
\[ \Rightarrow x = 30° \]
\[\text{ The polygon has n sides } . \]
\[\text{ So, sum of all the exterior angles } = \left( 30n \right)° \]
\[\text{ We know that the sum of all the exterior angles of a polygon is } 360° . \]
\[i . e . , 30n = 360\]
\[ \therefore n = 12\]
APPEARS IN
संबंधित प्रश्न
In a quadrilateral, define of the following Opposite angles .
In a quadrilateral, define of the following Interior .
Two angles of a quadrilateral are of measure 65° and the other two angles are equal. What is the measure of each of these two angles?
In a quadrilateral ABCD, CO and DO are the bisectors of ∠C and ∠D respectively. Prove that \[∠COD = \frac{1}{2}(∠A + ∠B) .\]
Mark the correct alternative in each of the following:
The opposite sides of a quadrilateral have
ABCDE is a regular pentagon. The bisector of angle A of the pentagon meets the side CD in point M. Show that ∠AMC = 90°.
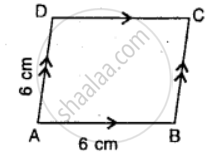
Write, giving reason, the name of the figure drawn alongside. Under what condition will this figure be a square.
In the quadrilateral ABCD, AB = BC and AD = DC Measure of ∠BCD is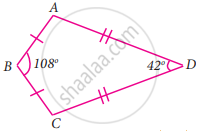
Using the information given, name the right angles in part of figure:
RT ⊥ ST
Draw a rough sketch of a quadrilateral KLMN. State two pairs of opposite angles.
