Advertisements
Advertisements
प्रश्न
Determine the centre of the circle on which the points (1, 7), (7 – 1), and (8, 6) lie. What is the radius of the circle?
उत्तर
Let P(x, y) be the centre of the circle and A(1, 7), B(7, -1) and C(8, 6) be the given points.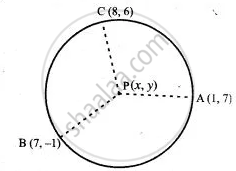
Then PA = PB = PC = radius
⇒ PA2 = PB = PC = r2
⇒ (x - 1)2 + y( - 7)2 = r2 ...(i)
(x - 7)2 + (y + 1)2 = r2 ...(ii)
Also (x - 8)2 + (y - 6)2 = r2 ...(iii)
Subtracting (ii) from (i)
(x2 + 1 - 2x + y2 + 49 - 14y)
-(x2 + 49 - 14x + y2 + 1 + 2y) = 0
⇒ 12x - 16y = 0
`y = (3)/(4)x` ...(iv)
Subtracting (iii) from (ii)
⇒ (x2 + 49 - 14x + y2 + 1 + 2y)
-(x2 + 64 - 16x + y2 + 36 - 12y) = 0
⇒ 2x + 14y - 50 = 0
⇒ `x + 7(3/4x)-25` = 0
⇒ `(25x)/(4)` = 25
⇒ x = 4
From (iv) y = `(3)/(4)x = (3)/(4) xx 4`
y = 3.
The centre is P(4, 3).
Also radius, r = PA = `sqrt((4 - 1)^2 + (3 - 7)^2)`
= `sqrt(9 + 16)`
= 5 units.
APPEARS IN
संबंधित प्रश्न
Calculate the co-ordinates of the point P which divides the line segment joining: A (1, 3) and B (5, 9) in the ratio 1 : 2
Calculate the co-ordinates of the point P which divides the line segment joining: A (–4, 6) and B (3, –5) in the ratio 3 : 2
Calculate the ratio in which the line joining A(-4,2) and B(3,6) is divided by point p(x,3). Also, find x
A(–4, 2), B(0, 2) and C(–2, –4) are vertices of a triangle ABC. P, Q and R are mid-points of sides BC, CA and AB respectively. Show that the centroid of ΔPQR is the same as the centroid of ΔABC.
Prove that the points A(-5, 4), B(-1, -2) and C(S, 2) are the vertices of an isosceles right-angled triangle. Find the coordinates of D so that ABCD is a square.
The line segment joining A (2, 3) and B (6, – 5) is intersected by the X axis at the point K. Write the ordinate of the point K. Hence find the ratio in which K divides AB.
Show that the points A(1, 3), B(2, 6), C(5, 7) and D(4, 4) are the vertices of a rhombus.
= `a(1/t^2 + 1) = (a(t^2 + 1))/t^2`
Now `(1)/"SP" + (1)/"SQ" = (1)/(a(t^2 + 1)) + (1 xx t^2)/(a(t^2 + 1)`
= `((1 + t^2))/(a(t^2 + 1)`
`(1)/"SP" + (1)/"SQ" = (1)/a`.
If the line joining the points A(4, - 5) and B(4, 5) is divided by the point P such that `"AP"/"AB" = (2)/(5)`, find the coordinates of P.
Determine the ratio in which the line 3x + y – 9 = 0 divides the line joining (1, 3) and (2, 7).
From the adjacent figure:
(i) Write the coordinates of the points A, B, and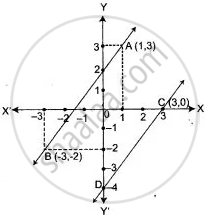
(ii) Write the slope of the line AB.
(iii) Line through C, drawn parallel to AB, intersects Y-axis at D. Calculate the co-ordinates of D.
