Advertisements
Advertisements
प्रश्न
Diagonals of a trapezium PQRS intersect each other at the point O, PQ || RS and PQ = 3 RS. Find the ratio of the areas of triangles POQ and ROS.
उत्तर
Given PQRS is a trapezium in which PQ || RS and PQ = 3 RS
⇒ `("PQ")/("RS") = 3/1` ...(i)
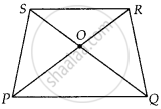
In ∆POQ and ∆ROS,
∠SOR = ∠QOP ...[Vertically opposite angles]
∠SRP = ∠RPQ ...[Alternate angles]
∴ ∆POQ ~ ∆ROS ...[By AAA similarity criterion]
By property of area of similar triangle,
`("ar(∆POQ)")/("ar(∆SOR)") = ("PQ")^2/("RS")^2`
= `("PQ"/"RS")^2`
= `(3/1)^2` ...[From equation (i)]
⇒ `("ar(∆POQ)")/("ar(∆SOR)") = 9/1`
Hence, the required ratio is 9 : 1.
APPEARS IN
संबंधित प्रश्न
State which pair of triangles in the following figure are similar. Write the similarity criterion used by you for answering the question, and also write the pairs of similar triangles in the symbolic form:
A vertical pole of a length 6 m casts a shadow 4m long on the ground and at the same time a tower casts a shadow 28 m long. Find the height of the tower.
D is the mid-point of side BC of a ΔABC. AD is bisected at the point E and BE produced cuts AC at the point X. Prove that BE : EX = 3 : 1
State the two properties which are necessary for given two triangles to be similar.
In a trapezium ABCD, it is given that AB║CD and AB = 2CD. Its diagonals AC and BD intersect at the point O such that ar(ΔAOB) = 84cm2. Find ar(ΔCOD).
In triangle PQR and MST, ∠P = 55°, ∠Q = 25°, ∠M = 100° and ∠S = 25°. Is ∆QPR ~ ∆TSM? Why?
D is a point on side QR of ΔPQR such that PD ⊥ QR. Will it be correct to say that ΔPQD ~ ΔRPD? Why?
A 15 metres high tower casts a shadow 24 metres long at a certain time and at the same time, a telephone pole casts a shadow 16 metres long. Find the height of the telephone pole.
In triangles ABC and DEF, ∠B = ∠E, ∠F = ∠C and AB = 3DE. Then, the two triangles are ______.
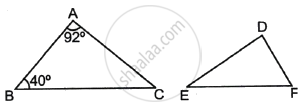
If ΔABC ∼ ΔDEF such that ∠A = 92° and ∠B = 40°, then ∠F = ?