Advertisements
Advertisements
प्रश्न
Father of a family wishes to divide his square field bounded by x = 0, x = 4, y = 4 and y = 0 along the curve y2 = 4x and x2 = 4y into three equal parts for his wife, daughter and son. Is it possible to divide? If so, find the area to be divided among them
उत्तर
Given curve y2 = 4x and x2 = 4y
Draw these two curves
Also draw the square bounded by the lines
x = 0, x = 4, y = 4 and y = 0
To prove Area A1 = Area A2 = Area A3
Now the point of intersection of the curves y2 = 4x and x2 = 4y is given by
`(y^2/4)^2` = 4y
y4 = 64y
⇒ y(y3 – 64) = 0
y = 0, y = 4
when y = 0
⇒ x = 0
y = 4
⇒ x = 4
Point of intersection are O(0, 0) and B(4, 4)
Now, the area of the region bounded by the curves y2 = 4x and x2 = 4y is
A2 = `int_0^4 (sqrt(4x) - x^2/4) "d"x`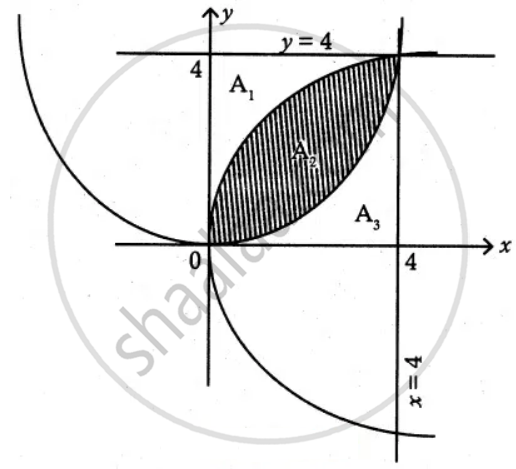
= `int_0^4 (2sqrt(x) - x^2/4) "d"x`
= `[2 x^(3/2)/(3/2) - x^3/12]_0^4`
= `[4/3 (4)^(3/2) - 4^3/12 - 0]`
= `32/3 - 16/3`
= `16/3` .......(1)
Now the area of the region bounded by the curves x2 = 4y, x = 4 and x axis is
A3 = `int_0^4 y "d"x`
= `int_0^4(x^2/4) "d"x`
= `[x^3/12]_0^4`
= `4^3/12 - 0`
= `16/3` sq.units ......(2)
Similarly the area of the region bounded by the curve y2 = 4x, y-axis and y = 4 is
A1 = `int_0^4 x "d"y`
= `int_0^4 (y^2/4) "d"y`
= `[y^3/12]_0^4`
= `1/12 xx 4^3`
= `16/3` ......(3)
Hence we see that
A1 = A2 = A3 = `16/3` sq.units
APPEARS IN
संबंधित प्रश्न
Find the area of the region bounded by 3x – 2y + 6 = 0, x = – 3, x = 1 and x-axis
Find the area of the region bounded by 2x – y + 1 = 0, y = – 1, y = 3 and y-axis
Find the area of the region bounded by the curve 2 + x – x2 + y = 0, x axis, x = – 3 and x = 3
Find the area of the region bounded by the line y = 2x + 5 and the parabola y = x2 – 2x
Find the area of the region bounded between the curves y = sin x and y = cos x and the lines x = 0 and x = π
Find the area of the region bounded by y = tan x, y = cot x and the lines x = 0, x = `pi/2`, y = 0
Find the area of the region bounded by the parabola y2 = x and the line y = x – 2
The curve y = (x – 2)2 + 1 has a minimum point at P. A point Q on the curve is such that the slope of PQ is 2. Find the area bounded by the curve and the chord PQ
Find the area of the region common to the circle x2 + y2 = 16 and the parabola y2 = 6x
Choose the correct alternative:
The area between y2 = 4x and its latus rectum is
Choose the correct alternative:
If `int_0^"a" 1/(4 + x^2) "dx=pi/8` then a is
