Advertisements
Advertisements
प्रश्न
Find the area bounded by curves (x – 1)2 + y2 = 1 and x2 + y 2 = 1
उत्तर
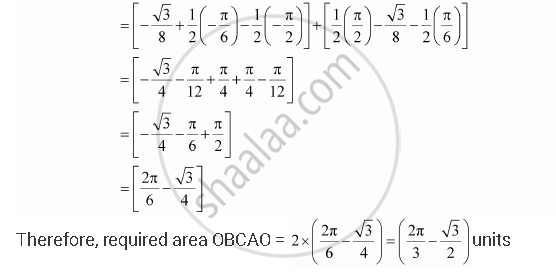
The area bounded by the curves, (x – 1)2 + y2 = 1 and x2 + y 2 = 1, is represented by the shaded area as
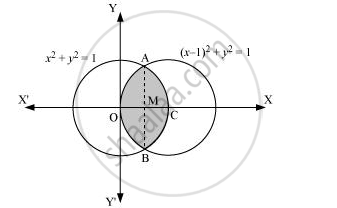
On solving the equations, (x – 1)2 + y2 = 1 and x2 + y 2 = 1, we obtain the point of intersection as A

APPEARS IN
संबंधित प्रश्न
Find the area of the circle 4x2 + 4y2 = 9 which is interior to the parabola x2 = 4y
Find the area of the region bounded by the curves y = x2 + 2, y = x, x = 0 and x = 3
Using integration finds the area of the region bounded by the triangle whose vertices are (–1, 0), (1, 3) and (3, 2).
Using integration find the area of the triangular region whose sides have the equations y = 2x +1, y = 3x + 1 and x = 4.
Smaller area enclosed by the circle x2 + y2 = 4 and the line x + y = 2 is
A. 2 (π – 2)
B. π – 2
C. 2π – 1
D. 2 (π + 2)
Area lying between the curve y2 = 4x and y = 2x is
A. 2/3
B. 1/3
C. 1/4
D. 3/4
Using the method of integration find the area bounded by the curve |x| + |y| = 1 .
[Hint: The required region is bounded by lines x + y = 1, x– y = 1, – x + y = 1 and
– x – y = 1].
Find the area bounded by curves {(x, y) : y ≥ x2 and y = |x|}.
Using integration, find the area of region bounded by the triangle whose vertices are (–2, 1), (0, 4) and (2, 3).
The area enclosed between the curves y = loge (x + e), x = loge \[\left( \frac{1}{y} \right)\] and the x-axis is _______ .
The area between x-axis and curve y = cos x when 0 ≤ x ≤ 2 π is ___________ .
Solve the following :
Find the area of the region lying between the parabolas :
y2 = 4x and x2 = 4y
The area of the region included between the parabolas y2 = 16x and x2 = 16y, is given by ______ sq.units
The area enclosed between the two parabolas y2 = 20x and y = 2x is ______ sq.units
The area of triangle ΔABC whose vertices are A(1, 1), B(2, 1) and C(3, 3) is ______ sq.units
Find the area enclosed between y = cos x and X-axis between the lines x = `pi/2` and x ≤ `(3pi)/2`
Find the area enclosed between the X-axis and the curve y = sin x for values of x between 0 to 2π
Find the area of the ellipse `x^2/36 + y^2/64` = 1, using integration
Find the area of the region lying between the parabolas 4y2 = 9x and 3x2 = 16y
Find the area of the region included between y = x2 + 5 and the line y = x + 7
Find the area of the region bounded by the curves x = at2 and y = 2at between the ordinate corresponding to t = 1 and t = 2.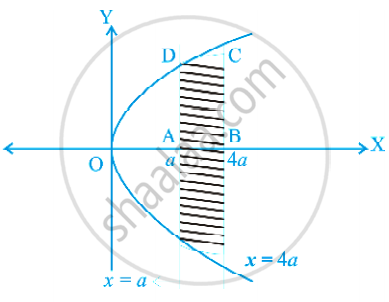
Find the area of a minor segment of the circle x2 + y2 = a2 cut off by the line x = `"a"/2`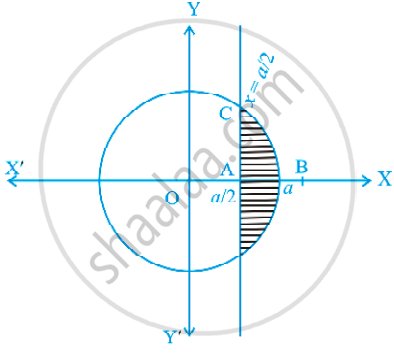
Calcualte the area under the curve y = `2sqrt(x)` included between the lines x = 0 and x = 1
Determine the area under the curve y = `sqrt("a"^2 - x^2)` included between the lines x = 0 and x = a.
The value of a for which the area between the curves y2 = 4ax and x2 = 4ay is 1 sq.unit, is ______.
Let the area enclosed by the x-axis, and the tangent and normal drawn to the curve 4x3 – 3xy2 + 6x2 – 5xy – 8y2 + 9x + 14 = 0 at the point (–2, 3) be A. Then 8A is equal to ______.
Using Integration, find the area of triangle whose vertices are (– 1, 1), (0, 5) and (3, 2).
Find the area enclosed between 3y = x2, X-axis and x = 2 to x = 3.
Find the area cut off from the parabola 4y = 3x2 by the line 2y = 3x + 12.
