Advertisements
Advertisements
प्रश्न
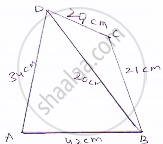
Find the area of a quadrilateral ABCD in which AB = 42 cm, BC = 21 cm, CD = 29 cm, DA =34 cm and diagonal BD =20 cm.
उत्तर
Given that
Sides of a quadrilateral are AB = 42 cm, BC = 21 cm, CD = 29 cm
DA = 34 cm and diagonal BD = 20 cm
Area of quadrilateral = area of ΔADB + area of ΔBCD.
Now, area of ΔABD
Perimeter of ΔABD
We know that
⇒ S = `1/2`(𝐴𝐵+𝐵𝐷+𝐷𝐴)
= `1/2`(34+42+20)=96
=`96/2`
= 48 𝑐𝑚
Area of ΔABD = `sqrt(S(S-AB)(S-BD)(S-DA))`
`=sqrt(48(48-42)(48-20)(48-34))`
`=sqrt(48(14)(6)(28)`
`=336cm^2`
Also for area of ΔBCD,
Perimeter of ΔBCD
`2s = BC + CD + BD`
`⇒S=1/2(29+21+20)+35cm`
By using heron’s formulae
Area of ΔBCD =`sqrt(s(s-bc)(s-cd)(s-db))`
`=sqrt(35(35-21)(53-29)(35-20))`
`=sqrt(210xx210)cm^2`
`= 210cm^2`
∴ Area of quadrilateral ABCD = 336 + 210 = 546 `cm^2`
APPEARS IN
संबंधित प्रश्न
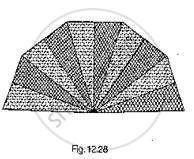
A hand fan is made by stitching lo equal size triangular strips of two different types of paper as shown in Fig. 12.28. The dimensions of equal strips are 25 cm, 25 cm and 14 cm. Find the area of each type of paper needed to make the hand fan.
Find the area of a triangle whose base and altitude are 5 cm and 4 cm respectively.
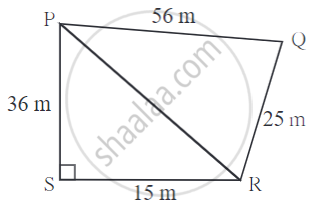
Look at the measures shown in the adjacent figure and find the area of ☐ PQRS.
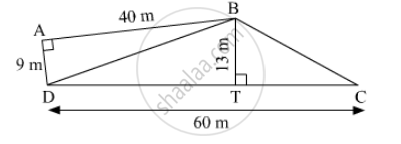
Some measures are given in the adjacent figure, find the area of ☐ABCD.
Determine whether the sets of points are collinear?
(a, b + c), (b, c + a) and (c, a + b)
If (5, 7), (3, p) and (6, 6) are collinear, then the value of p is
The sides of the triangular ground are 22 m, 120 m and 122 m. Find the area and cost of levelling the ground at the rate of ₹ 20 per m2
The area of the isosceles triangle is `5/4 sqrt(11)` cm2, if the perimeter is 11 cm and the base is 5 cm.
The sides of a quadrilateral ABCD are 6 cm, 8 cm, 12 cm and 14 cm (taken in order) respectively, and the angle between the first two sides is a right angle. Find its area.
The perimeter of a triangle is 50 cm. One side of a triangle is 4 cm longer than the smaller side and the third side is 6 cm less than twice the smaller side. Find the area of the triangle.
