Advertisements
Advertisements
प्रश्न
Find the ratio in which the line segment joining the points A(3, 8) and B(–9, 3) is divided by the Y– axis.
उत्तर
Let C be a point on Y-axis which divides seg AB in the ratio m: n.
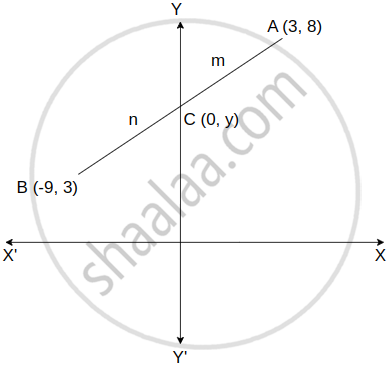
Point C lies on the Y-axis. Thus, its x coordinate is 0.
Let C = (0, y)
∴ x1 = −9, y1 = 3, x2 = 3, y2 = 8
∴ By section formula,
`"x" = ("mx"_2 + "nx"_1)/"m + n"`
∴ `0 = "m × 3 + n × −9"/"m + n"`
∴ `0 = "3m − 9n"/"m + n"`
∴ 0 = 3m − 9n
∴ 9n = 3m
∴ `"m"/"n" = 9/3`
∴ `"m"/"n" = 3/1`
∴ m : n = 3 : 1
Y-axis divides the seg AB in the ratio 3: 1.
APPEARS IN
संबंधित प्रश्न
If the points A(k + 1, 2k), B(3k, 2k + 3) and C(5k − 1, 5k) are collinear, then find the value of k
If (−2, 3), (4, −3) and (4, 5) are the mid-points of the sides of a triangle, find the coordinates of its centroid.
The area of the triangle formed by the points A(2,0) B(6,0) and C(4,6) is
Find the value of k if points A(k, 3), B(6, −2) and C(−3, 4) are collinear.
If R (x, y) is a point on the line segment joining the points P (a, b) and Q (b, a), then prove that x + y = a + b.
If the points A(−2, 1), B(a, b) and C(4, −1) ae collinear and a − b = 1, find the values of aand b.
If the distance between points (x, 0) and (0, 3) is 5, what are the values of x?
If the distance between the points (3, 0) and (0, y) is 5 units and y is positive. then what is the value of y?
Find the area of triangle with vertices ( a, b+c) , (b, c+a) and (c, a+b).
If x is a positive integer such that the distance between points P (x, 2) and Q (3, −6) is 10 units, then x =
The coordinates of a point on x-axis which lies on the perpendicular bisector of the line segment joining the points (7, 6) and (−3, 4) are
Which of the points P(-1, 1), Q(3, - 4), R(1, -1), S (-2, -3), T(-4, 4) lie in the fourth quadrant?
The point R divides the line segment AB, where A(−4, 0) and B(0, 6) such that AR=34AB.">AR = `3/4`AB. Find the coordinates of R.
The line segment joining the points (3, -1) and (-6, 5) is trisected. The coordinates of point of trisection are ______.
If the coordinates of the two points are P(–2, 3) and Q(–3, 5), then (abscissa of P) – (abscissa of Q) is ______.
The points whose abscissa and ordinate have different signs will lie in ______.
Points (1, –1) and (–1, 1) lie in the same quadrant.
Find the coordinates of the point which lies on x and y axes both.
The distance of the point (–6, 8) from x-axis is ______.
The distance of the point (–4, 3) from y-axis is ______.
