Advertisements
Advertisements
प्रश्न
Find the value of a so that the point (3, a) lies on the line represented by 2x − 3y + 5 = 0
उत्तर
It the point (3, a) lies on the line 2x - 3y = 5
then
2 (3) - 3 (a) = 5
⇒ 6 - 3a = 5
⇒ 3a = 6 - 5
⇒ 3a = 1
∴ a =`1/3`
APPEARS IN
संबंधित प्रश्न
Let ABCD be a square of side 2a. Find the coordinates of the vertices of this square when A coincides with the origin and AB and AD are along OX and OY respectively.
Find the point on x-axis which is equidistant from the points (−2, 5) and (2,−3).
Find the points of trisection of the line segment joining the points:
5, −6 and (−7, 5),
Find the coordinates of the point where the diagonals of the parallelogram formed by joining the points (-2, -1), (1, 0), (4, 3) and(1, 2) meet
Prove that the points A(-4,-1), B(-2, 4), C(4, 0) and D(2, 3) are the vertices of a rectangle.
Show that the following points are the vertices of a rectangle
A (0,-4), B(6,2), C(3,5) and D(-3,-1)
Find the area of quadrilateral ABCD whose vertices are A(-5, 7), B(-4, -5) C(-1,-6) and D(4,5)
If the point A(0,2) is equidistant from the points B(3,p) and C(p, 5), find p.
ABCD is a rectangle whose three vertices are A(4,0), C(4,3) and D(0,3). Find the length of one its diagonal.
Find the ratio in which the line segment joining the points A(3, 8) and B(–9, 3) is divided by the Y– axis.
Mark the correct alternative in each of the following:
The point of intersect of the coordinate axes is
If the point P(x, 3) is equidistant from the point A(7, −1) and B(6, 8), then find the value of x and find the distance AP.
Find the values of x for which the distance between the point P(2, −3), and Q (x, 5) is 10.
If the distance between the points (4, p) and (1, 0) is 5, then p =
If A (2, 2), B (−4, −4) and C (5, −8) are the vertices of a triangle, than the length of the median through vertex C is
In Fig. 14.46, the area of ΔABC (in square units) is

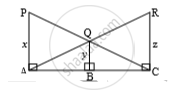
In the above figure, seg PA, seg QB and RC are perpendicular to seg AC. From the information given in the figure, prove that: `1/x + 1/y = 1/z`
Points (1, –1) and (–1, 1) lie in the same quadrant.
