Advertisements
Advertisements
प्रश्न
If a, b, c are all non-zero and a + b + c = 0, prove that `a^2/(bc) + b^2/(ca) + c^2/(ab) = 3`.
उत्तर
To prove, `a^2/(bc) + b^2/(ca) + c^2/(ab) = 3`
We know that, a3 + b3 + c3 – 3abc = (a + b + c)(a2 + b2 + c2 – ab – bc – ca)
= 0(a2 + b2 + c2 – ab – bc – ca) ...[∵ a + b + c = 0, given]
= 0
⇒ a3 + b3 + c3 = 3abc
On dividing both sides by abc, we get
`a^3/(abc) + b^3/(abc) + c^3/(abc) = 3`
⇒ `a^2/(bc) + b^2/(ac) + c^2/(ab) = 3`
Hence proved.
APPEARS IN
संबंधित प्रश्न
Find p(0), p(1) and p(2) for the following polynomial:-
p(y) = y2 – y + 1
Find p(0), p(1) and p(2) for the following polynomial:-
p(t) = 2 + t + 2t2 – t3
Find the zero of the polynomial in the following case:
p(x) = 3x
Find the zero of the polynomial in the following case:
p(x) = ax, a ≠ 0
Verify whether the following are zeros of the polynomial, indicated against them, or not
p(x) = (x + 3) (x – 4), x = −3, x = 4
Find the number of zeros of the following polynomial represented by their graph
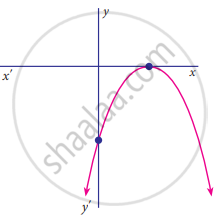
Find p(0), p(1), p(–2) for the following polynomial:
p(y) = (y + 2)(y – 2)
–3 is a zero of x – 3
–3 is a zero of y2 + y – 6
Find the zeroes of the polynomial in the following:
q(x) = 2x – 7
