Advertisements
Advertisements
प्रश्न
If perimeter of a rhombus is 100 cm and length of one diagonal is 48 cm, what is the area of the quadrilateral?
उत्तर

Perimeter of the rhombus = 100 cm
⇒ `4 xx` side = 100
⇒ side = `100/4` = 25 cm
Thus, each side of the rhombus = 25 cm.
Diagonals of a rhombus are perpandicular bisector of each other.
So, AO = OC = `48/2` = 24 cm
In Δ AOB,
We apply Pythagoras theorem,
AO² + OB² = AB²
⇒ 24² + OB² = 25²
⇒ OB² = 625 - 576 = 49
⇒ OB = 7 cm
So, DB = `2 xx "OB" = 2 xx 7` = 14 cm
Area of rhombus = `1/2 xx` (product of diagonal)
= `1/2 xx 14 xx 48`
= 336 cm²
संबंधित प्रश्न
The diagonals of a rhombus are 18 cm and 24 cm. Find:
(i) its area ;
(ii) length of its sides.
(iii) its perimeter
The length of the diagonals of a rhombus is in ratio 4 : 3. If its area is 384 cm2, find its side.
Find the area of a rhombus whose diagonals are of lengths 10 cm and 8.2 cm.
Find the area of a rhombus whose base is 14 cm and height is 9 cm.
Find the missing value.
| Diagonal (d1) | Diagonal (d2) | Area |
| 12 mm | 180 sq.mm |
If the diagonals of a rhombus get doubled, then the area of the rhombus becomes ______ its original area.
The area of a rectangular field is 48 m2 and one of its sides is 6 m. How long will a lady take to cross the field diagonally at the rate of 20 m/minute?
The walls and ceiling of a room are to be plastered. The length, breadth and height of the room are 4.5 m, 3 m, and 350 cm respectively. Find the cost of plastering at the rate of Rs 8 per m2.
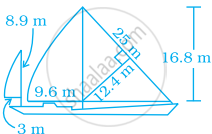
Most of the sailboats have two sails, the jib and the mainsail. Assume that the sails are triangles. Find the total area sail of the sailboats to the nearest tenth.
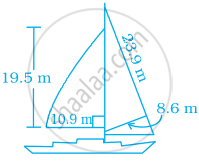
Most of the sailboats have two sails, the jib and the mainsail. Assume that the sails are triangles. Find the total area of sail of the sailboats to the nearest tenth.