Advertisements
Advertisements
प्रश्न
If the points A (6, 1), B (8, 2), C (9, 4) and D (k, p) are the vertices of a parallelogram taken in order, then find the values of k and p.
उत्तर
Let ABCD be a parallelogram in which the coordinates of the vertices are A (6, 1); B (8, 2); C (9, 4) and D (k, p).
Since ABCD is a parallelogram, the diagonals bisect each other. Therefore the mid-point of the diagonals of the parallelogram will coincide.
In general to find the mid-point P(x,y) of two points `A(x_1, y_1)` and `B(x_2,y_2)` we use section formula as,
`P(x,y) = ((x_1 + x_2)/2","(y_1 + y_2)/2)`
The mid-point of the diagonals of the parallelogram will coincide.
So,
Co-ordinate of mid-point o AC = Co-ordinate of mid-point of BD
Therefore,
`((6 + 9)/2, (4 + 1)/2) = ((k + 8)/2","(p + 2)/2))`
Now equate the individual terms to get the unknown value. So,
`(k + 8)/2 = 15/2`
k = 7
Similarly,
`(p + 2)/2 = 5/2`
p = 3
Therefore, k = 7 and p = 3
APPEARS IN
संबंधित प्रश्न
Determine the ratio in which the line 3x + y – 9 = 0 divides the segment joining the points (1, 3) and (2, 7)
The three vertices of a parallelogram taken in order are (–1, 0), (3, 1) and (2, 2) respectively. Find the coordinates of the fourth vertex.
If A (5, –1), B(–3, –2) and C(–1, 8) are the vertices of triangle ABC, find the length of median through A and the coordinates of the centroid.
Find the length of the medians of a ΔABC having vertices at A(0, -1), B(2, 1) and C(0, 3).
The line segment joining the points (3, -4) and (1, 2) is trisected at the points P and Q. If the coordinates of P and Q are (p, -2) and (5/3, q) respectively. Find the values of p and q.
The line joining P(–4, 5) and Q(3, 2) intersects the y-axis at point R. PM and QN are perpendicular from P and Q on the x-axis Find:
- the ratio PR : RQ
- the coordinates of R.
- the area of the quadrilateral PMNQ.
In the given figure, line APB meets the x-axis at point A and y-axis at point B. P is the point (−4, 2) and AP : PB = 1 : 2. Find the co-ordinates of A and B.
A(20, 0) and B(10, –20) are two fixed points. Find the co-ordinates of the point P in AB such that : 3PB = AB. Also, find the co-ordinates of some other point Q in AB such that : AB = 6 AQ.
Point P(– 4, 6) divides point A(– 6, 10) and B(m, n) in the ratio 2:1, then find the coordinates of point B
Complete the following activity to find the coordinates of point P which divides seg AB in the ratio 3:1 where A(4, – 3) and B(8, 5).
Activity: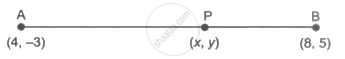
∴ By section formula,
∴ x = `("m"x_2 + "n"x_1)/square`,
∴ x = `(3 xx 8 + 1 xx 4)/(3 + 1)`,
= `(square + 4)/4`,
∴ x = `square`,
∴ y = `square/("m" + "n")`
∴ y = `(3 xx 5 + 1 xx (-3))/(3 + 1)`
= `(square - 3)/4`
∴ y = `square`
