Advertisements
Advertisements
प्रश्न
If the difference of Mode and Median of a data is 24, then the difference of median and mean is ______.
विकल्प
12
24
8
36
उत्तर
If the difference of Mode and Median of a data is 24, then the difference of median and mean is 12.
Explanation:
Given: Mode − Median = 24
We know that
Mode = 3Median − 2Mean
Now, Mode − Median = 2(Median − Mean)
⇒ 24 = 2(Median − Mean)
⇒ Median − Mean = 12
APPEARS IN
संबंधित प्रश्न
A life insurance agent found the following data for distribution of ages of 100 policy holders. Calculate the median age, if policies are given only to persons having age 18 years onwards but less than 60 years.
| Age (in years) | Number of policy holders |
| Below 20 | 2 |
| 20 - 25 | 4 |
| 25 - 30 | 18 |
| 30 - 35 | 21 |
| 35 - 40 | 33 |
| 40 - 45 | 11 |
| 45 - 50 | 3 |
| 50 - 55 | 6 |
| 55 - 60 | 2 |
A student got the following marks in 9 questions of a question paper.
3, 5, 7, 3, 8, 0, 1, 4 and 6.
Find the median of these marks.
The weights (in kg) of 10 students of a class are given below:
21, 28.5, 20.5, 24, 25.5, 22, 27.5, 28, 21 and 24.
Find the median of their weights.
Calculate the median from the following data:
| Height(in cm) | 135 - 140 | 140 - 145 | 145 - 150 | 150 - 155 | 155 - 160 | 160 - 165 | 165 - 170 | 170 - 175 |
| Frequency | 6 | 10 | 18 | 22 | 20 | 15 | 6 | 3 |
Find the correct answer from the alternatives given.
| Distance Covered per litre (km) | 12 - 14 | 14 - 16 | 16 - 18 | 18 - 20 |
| No. of cars | 11 | 12 | 20 | 7 |
The median of the distances covered per litre shown in the above data is in the group . . . . . .
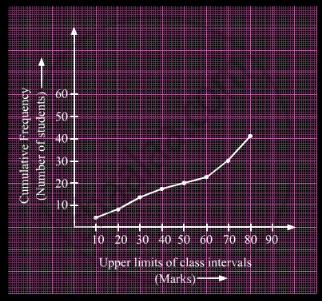
A student draws a cumulative frequency curve for the marks obtained by 40 students of a class as shown below. Find the median marks obtained by the students of the class.
In a hospital, weights of new born babies were recorded, for one month. Data is as shown:
| Weight of new born baby (in kg) | 1.4 - 1.8 | 1.8 - 2.2 | 2.2 - 2.6 | 2.6 - 3.0 |
| No of babies | 3 | 15 | 6 | 1 |
Then the median weight is?
If in a frequency distribution, the mean and median are 21 and 22 respectively, then its mode is approximately ______.
The empirical relation between the mode, median and mean of a distribution is ______.
The median of first 10 natural numbers is ______.
