Advertisements
Advertisements
प्रश्न
If the lines `(x - 1)/2 = (y + 1)/3 = (z - 1)/4 and (x - 3)/1 = (y - k)/2 = z/1` intersect each other, then find k.
उत्तर
The lines `(x - x_1)/l_1 = (y - y_1)/m_1 = (z - z_1)/n_1 and (x - x_2)/(l_2) = (y - y_2)/m_2 = (z - z_2)/n_2`
intersect, if `|(x_2 - x_1, y_2 - y_1,z_2 - z_1),(l_1, m_1, n_1),(l_2, m_2, n_2)|` = 0
The equations of the given lines are
`(x - 1)/(2) = (y +1)/3 = (z - 1)/4 and (x - 3)/1 = (y - k)/2 = z/1`
∴ x1 = 1, y1 = –1, z1 = 1, x2 = 3, y2 = k, z2 = 0,
l1 = 2, m1 = 3, n1 = 4, l2 = 1, m2 = 2, n2 = 1.
Since these lines intersect, we get
`|(2, k + 1, -1),(2, 3, 4),(1, 2, 1)|` = 0
∴ 2(3 – 8) – (k + 1)(2 – 4) – 1(4 – 3) = 0
∴ – 10 + 2(k + 1) – 1 = 0
∴ 2(k + 1) = 11
∴ k + 1 `11/2`
∴ k = `9/2`
APPEARS IN
संबंधित प्रश्न
Find the co-ordinates of the foot of the perpendicular drawn from the point `2hati - hatj + 5hatk` to the line `barr = (11hati - 2hatj - 8hatk) + λ(10hati - 4hatj - 11hatk).` Also find the length of the perpendicular.
Find the perpendicular distance of the origin from the plane 6x – 2y + 3z – 7 = 0.
Reduce the equation `bar"r".(3hat"i" + 4hat"j" + 12hat"k")` to normal form and hence find
(i) the length of the perpendicular from the origin to the plane
(ii) direction cosines of the normal.
Find the co-ordinates of the foot of the perpendicular drawn from the point (0, 2, 3) to the line `(x + 3)/(5) = (y - 1)/(2) = (z + 4)/(3)`.
Choose correct alternatives :
Equation of X-axis is ______.
Choose correct alternatives :
The direction cosines of the normal to the plane 2x – y + 2z = 3 are ______
Find the direction ratios of the normal to the plane 2x + 3y + z = 7
Find direction cosines of the normal to the plane `bar"r"*(3hat"i" + 4hat"k")` = 5
If z1 and z2 are z-coordinates of the points of trisection of the segment joining the points A (2, 1, 4), B (–1, 3, 6) then z1 + z2 = ______.
If the line `(x - 3)/2 = (y + 2)/-1 = (z + 4)/3` lies in the plane lx + my - z = 9, then l2 + m2 is equal to ______
The equation of the plane passing through the point (– 1, 2, 1) and perpendicular to the line joining the points (– 3, 1, 2) and (2, 3, 4) is ______.
The equation of a plane containing the line of intersection of the planes 2x - y - 4 = 0 and y + 2z - 4 = 0 and passing through the point (1, 1, 0) is ______
XY-plane divides the line joining the points A(2, 3, -5) and B(1, -2, -3) in the ratio ______
The equation of the plane through (1, 2, -3) and (2, -2, 1) and parallel to the X-axis is ______
Equation of the plane perpendicular to the line `x/1 = y/2 = z/3` and passing through the point (2, 3, 4) is ______
The equation of the plane through the point (2, -1, -3) and parallel to the lines `(x - 1)/3 = (y + 2)/2 = z/(-4)` and `x/2 = (y - 1)/(-3) = (z - 2)/2` is ______
The equation of the plane, which bisects the line joining the points (1, 2, 3) and (3, 4, 5) at right angles is ______
A plane which passes through the point (3, 2, 0) and the line `(x - 3)/1 = (y - 6)/5, (z - 4)/4` is ______
If the plane passing through the points (1, 2, 3), (2, 3, 1) and (3, 1, 2) is ax + by + cz = d then a + 2b + 3c = ______.
The equation of the plane passing through the intersection of the planes x + 2y + 3z + 4 = 0 and 4x + 3y + 2z + 1 = 0 and the origin is ______.
If the plane x - 3y + 5z = d passes through the point (1, 2, 4), then the lengths of intercepts cut by it on the axes of X, Y, Z are respectively ______
The d.r.s of normal to the plane through (1, 0, 0), (0, 1, 0) which makes an angle `pi/4` with plane x + y = 3, are ______.
The equation of the plane passing through the points (1, –2, 1), (2, –1, –3) and (0, 1, 5) is ______.
The equation of the plane passing through a point having position vector`-2hat"i" + 7hat"j" + 5hat"k"` and parallel to the vectors `4hat"i" - hat"j" + 3hat"k"` and `hat"i" + hat"j" + hat"k"` is ______.
If plane x + ay + z = 4 has equal intercepts on axes, then 'a' is equal to ______.
Let P be a plane Ix + my + nz = 0 containing the line, `(1 - x)/1 = ("y" + 4)/2 = ("z" + 2)/3`. If plane P divides the line segment AB joining points A(–3, –6, 1) and B(2, 4, –3) in ratio k:1 then the value of k is equal to ______.
The equation of the plane through the line x + y + z + 3 = 0 = 2x – y + 3z + 1 and parallel to the line `x/1 = y/2 = z/3`, is ______.
Find the equation of the plane containing the lines `(x - 1)/2 = (y + 1)/-1 = z/3` and `x/2 = (y - 2)/-1 = (z + 1)/3`.
Reduce the equation `barr*(3hati - 4hatj + 12hatk)` = 3 to the normal form and hence find the length of perpendicular from the origin to the plane.
Find the equation of plane which is at a distance of 4 units from the origin and which is normal to the vector `2hati - 2hatj + hatk`.
The coordinates of the foot of the perpendicular from the point P(1, 0, 0) in the line `(x - 1)/2 = (y + 1)/-3 = (z + 10)/8` are ______.
Find the vector equation of the line passing through the point (–2, 1, 4) and perpendicular to the plane `barr*(4hati - 5hatj + 7hatk)` = 15
Find the equation of the plane which contains the line of intersection of the planes x + 2y + 4z = 4 and 2x – 3y – z = 9 and which is perpendicular to the plane 4x – 3y + 5z = 10.
A mobile tower is situated at the top of a hill. Consider the surface on which the tower stands as a plane having points A(1, 0, 2), B(3, –1, 1) and C(1, 2, 1) on it. The mobile tower is tied with three cables from the points A, B and C such that it stands vertically on the ground. The top of the tower is at point P(2, 3, 1) as shown in the figure below. The foot of the perpendicular from the point P on the plane is at the point `Q(43/29, 77/29, 9/29)`.
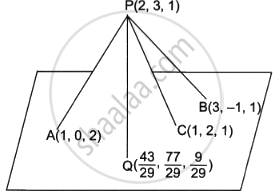
Answer the following questions.
- Find the equation of the plane containing the points A, B and C.
- Find the equation of the line PQ.
- Calculate the height of the tower.
Find the equation of the plane containing the line `x/(-2) = (y - 1)/3 = (1 - z)/1` and the point (–1, 0, 2).
