Advertisements
Advertisements
प्रश्न
If two sides of a triangle are 8 cm and 13 cm, then the length of the third side is between a cm and b cm. Find the values of a and b such that a is less than b.
उत्तर
The sum of any two sides of the triangle is always greater than third side of the triangle.
Third side < 13 + 8 = 21 cm.
The difference between any two sides of the triangle is always less than the third side of the triangle.
Third side > 13 - 8 = 5 cm.
Therefore, the length of the third side is between 5 cm and 21 cm, respectively.
The value of a = 5 cm and b = 21cm.
APPEARS IN
संबंधित प्रश्न
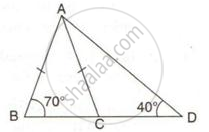
From the following figure, prove that: AB > CD.
In the following figure, write BC, AC, and CD in ascending order of their lengths.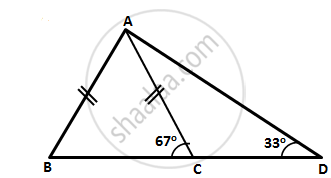
D is a point in side BC of triangle ABC. If AD > AC, show that AB > AC.
Arrange the sides of the following triangles in an ascending order:
ΔABC, ∠A = 45°, ∠B = 65°.
In ΔABC, the exterior ∠PBC > exterior ∠QCB. Prove that AB > AC.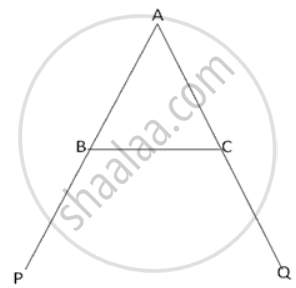
Prove that the perimeter of a triangle is greater than the sum of its three medians.
In ΔPQR, PR > PQ and T is a point on PR such that PT = PQ. Prove that QR > TR.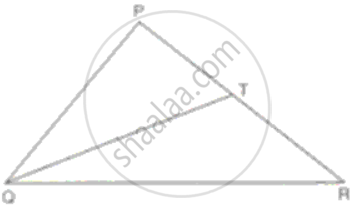
In the given figure, ∠QPR = 50° and ∠PQR = 60°. Show that : PN < RN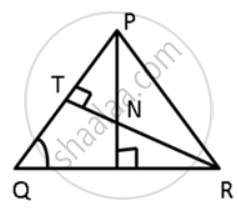
In ΔPQR, PS ⊥ QR ; prove that: PQ + PR > QR and PQ + QR >2PS.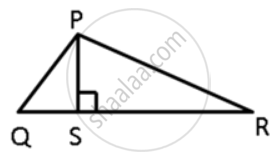
In ΔABC, D is a point in the interior of the triangle. Prove that DB + DC < AB + AC.
