Advertisements
Advertisements
प्रश्न
In ΔPQR, PS ⊥ QR ; prove that: PQ + PR > QR and PQ + QR >2PS.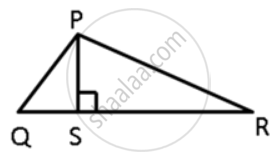
उत्तर
In ΔPQR,
PQ + PR > QR (∵ Sum of two sides of a triangle is always greater than third aside.)
In ΔPQS,
PQ + QS > PS (∵ Sum of two sides of a triangle is always greater than third aside.) .....(i)
In ΔPRS,
PR + SR > PS (∵ Sum of two sides of a triangle is always greater than third aside.) .....(ii)
Adding (i) and (ii),
PQ + QS + PR + SR > 2PS
PQ + (QS + SR) + PR > 2PS
PQ + QR + PR > 2PS
Since PQ + PR > QR
⇒ PQ + QR > 2PS.
APPEARS IN
संबंधित प्रश्न
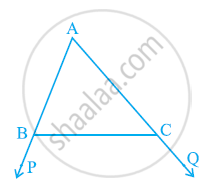
In the given figure sides AB and AC of ΔABC are extended to points P and Q respectively. Also, ∠PBC < ∠QCB. Show that AC > AB.
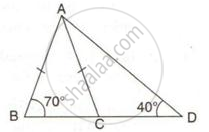
From the following figure, prove that: AB > CD.
Name the greatest and the smallest sides in the following triangles:
ΔXYZ, ∠X = 76°, ∠Y = 84°.
Arrange the sides of the following triangles in an ascending order:
ΔABC, ∠A = 45°, ∠B = 65°.
ABCD is a trapezium. Prove that:
CD + DA + AB + BC > 2AC.
ABCD is a trapezium. Prove that: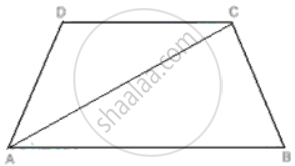
CD + DA + AB > BC.
In the given figure, T is a point on the side PR of an equilateral triangle PQR. Show that PT < QT
In ΔPQR is a triangle and S is any point in its interior. Prove that SQ + SR < PQ + PR.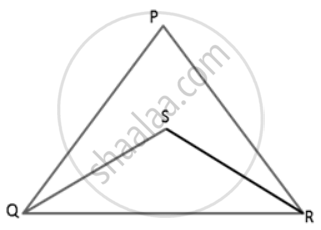
In ΔABC, AE is the bisector of ∠BAC. D is a point on AC such that AB = AD. Prove that BE = DE and ∠ABD > ∠C.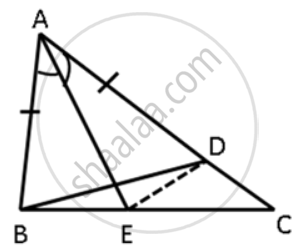
In ΔABC, D is a point in the interior of the triangle. Prove that DB + DC < AB + AC.
