Advertisements
Advertisements
प्रश्न
In ΔABC, AE is the bisector of ∠BAC. D is a point on AC such that AB = AD. Prove that BE = DE and ∠ABD > ∠C.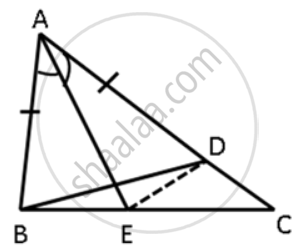
उत्तर
In the ΔABE and ΔADE,
AB = AD ....(Given)
∠BAE = ∠DAE ....(AE is the bisector of ∠BAC)
AE = AE ....(Common side)
∴ ΔABE ≅ ΔADE ....(SAS test)
⇒ BE = DE ....(c.p.c.t.c)
In ΔABD,
AB = AD
⇒ ∠ABD = ∠ADB
∠ADB > ∠C ...(Exterior angle property)
⇒ ∠ABD > ∠C.
APPEARS IN
संबंधित प्रश्न
In the following figure, ∠BAC = 60o and ∠ABC = 65o.

Prove that:
(i) CF > AF
(ii) DC > DF
In the following figure ; AC = CD; ∠BAD = 110o and ∠ACB = 74o.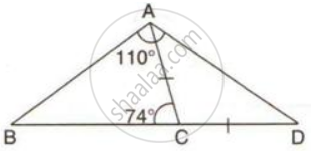
Prove that: BC > CD.
"Issues of caste discrimination began to be written about in many printed tracts and essays in India in the late nineteenth century." Support the statement with two suitable examples.
Name the greatest and the smallest sides in the following triangles:
ΔXYZ, ∠X = 76°, ∠Y = 84°.
Name the smallest angle in each of these triangles:
In ΔXYZ, XY = 6.2cm, XY = 6.8cm and YZ = 5cm
Prove that the hypotenuse is the longest side in a right-angled triangle.
For any quadrilateral, prove that its perimeter is greater than the sum of its diagonals.
ABCD is a trapezium. Prove that: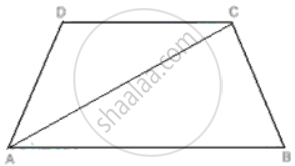
CD + DA + AB > BC.
In ΔPQR, PS ⊥ QR ; prove that: PQ + PR > QR and PQ + QR >2PS.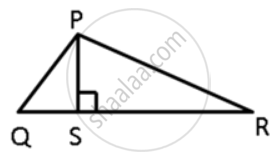
In the given figure, T is a point on the side PR of an equilateral triangle PQR. Show that RT < QT