Advertisements
Advertisements
प्रश्न
In the following figure, ∠BAC = 60o and ∠ABC = 65o.

Prove that:
(i) CF > AF
(ii) DC > DF
उत्तर
In ΔBEC,
∠B + ∠BEC + ∠BCE = 180°
∠B = 65° ...[Given]
∠BEC = 90° ...[CE is perpendicular to AB]
⇒ 65° + 90° + ∠BCE = 180°
⇒ ∠BCE = 180° - 155°
⇒ ∠BCE = 25°= ∠DCF …(i)
In ΔCDF,
∠DCF + ∠FDC + ∠CFD = 180°
∠DCF = 25° ....[From (i)]
∠FDC = 90° ...[ AD is perpendicular to BC]
⇒ 25°+ 90°+ ∠CFD = 180°
⇒ ∠CFD = 180° - 115°
⇒ ∠CFD = 65° …(ii)
Now, ∠AFC + ∠CFD = 180° ....[AFD is a straight line]
⇒ ∠AFC + 65° = 180°
⇒ ∠AFC = 115° …(iii)
In ΔACE,
∠ACE + ∠CEA + ∠BAC = 180°
∠BAC = 60° ....[Given]
⇒ ∠CEA = 90° ...[CE is perpendicular to AB]
⇒ ∠ACE + 90° + 60° = 180°
⇒ ∠ACE = 180° - 150°
∠ACE = 30° …(iv)
In ΔAFC,
∠AFC + ∠ACF + ∠FAC = 180°
∠AFC = 115° ....[From (iii)]
∠ACF = 30° ...[From (iv)]
⇒ 115° + 30° + ∠FAC = 180°
⇒ ∠FAC = 180° - 145°
⇒ ∠FAC = 35° …(v)
In ΔAFC,
⇒ ∠FAC = 35° ...[ From (v) ]
⇒ ∠ACF = 30° ...[ From (iv) ]
∴ ∠FAC > ∠ACF
⇒ CF > AF
In Δ CDF,
∠DCF = 25° ...[From (i)]
∠CFD = 65° ...[From (ii)]
∴ ∠CFD > ∠DCF
⇒ DC > DF
APPEARS IN
संबंधित प्रश्न
Show that in a right angled triangle, the hypotenuse is the longest side.
Show that of all line segments drawn from a given point not on it, the perpendicular line segment is the shortest.
In a huge park people are concentrated at three points (see the given figure):

A: where there are different slides and swings for children,
B: near which a man-made lake is situated,
C: which is near to a large parking and exit.
Where should an ice-cream parlour be set up so that maximum number of persons can approach it?
(Hint: The parlor should be equidistant from A, B and C)
From the following figure, prove that: AB > CD.
If two sides of a triangle are 8 cm and 13 cm, then the length of the third side is between a cm and b cm. Find the values of a and b such that a is less than b.
Arrange the sides of ∆BOC in descending order of their lengths. BO and CO are bisectors of angles ABC and ACB respectively.
In ΔABC, the exterior ∠PBC > exterior ∠QCB. Prove that AB > AC.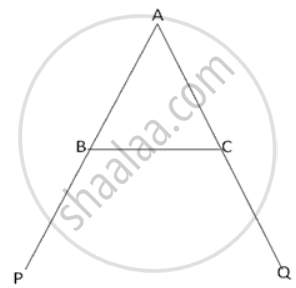
Prove that the hypotenuse is the longest side in a right-angled triangle.
In ΔPQR, PS ⊥ QR ; prove that: PQ + PR > QR and PQ + QR >2PS.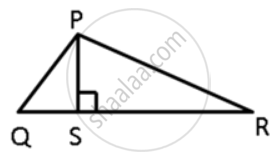
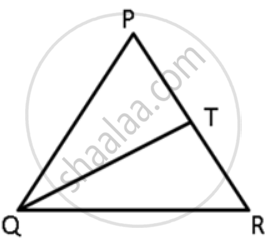
In the given figure, T is a point on the side PR of an equilateral triangle PQR. Show that RT < QT