Advertisements
Advertisements
प्रश्न
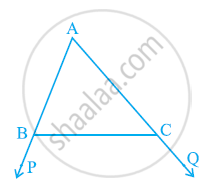
In the given figure sides AB and AC of ΔABC are extended to points P and Q respectively. Also, ∠PBC < ∠QCB. Show that AC > AB.
उत्तर
In the given figure,
∠ABC + ∠PBC = 180° (Linear pair)
⇒ ∠ABC = 180° − ∠PBC ... (1)
Also,
∠ACB + ∠QCB = 180°
∠ACB = 180° − ∠QCB … (2)
As ∠PBC < ∠QCB,
⇒ 180º − ∠PBC > 180º − ∠QCB
⇒ ∠ABC > ∠ACB [From equations (1) and (2)]
⇒ AC > AB (Side opposite to the larger angle is larger.)
APPEARS IN
संबंधित प्रश्न
In the given figure, ∠B < ∠A and ∠C < ∠D. Show that AD < BC.

How had the position of women improved in our country since independence ? Explain with examples.
From the following figure, prove that: AB > CD.
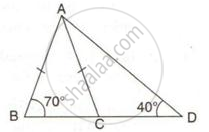
In the following figure, write BC, AC, and CD in ascending order of their lengths.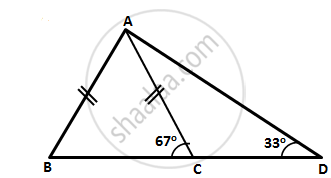
In the following figure, ∠BAC = 60o and ∠ABC = 65o.

Prove that:
(i) CF > AF
(ii) DC > DF
In the following figure, write BC, AC, and CD in ascending order of their lengths.
"Issues of caste discrimination began to be written about in many printed tracts and essays in India in the late nineteenth century." Support the statement with two suitable examples.
ΔABC is isosceles with AB = AC. If BC is extended to D, then prove that AD > AB.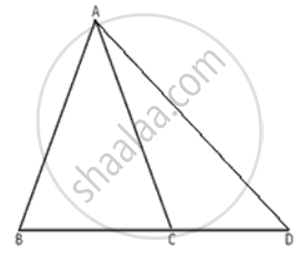
D is a point on the side of the BC of ΔABC. Prove that the perimeter of ΔABC is greater than twice of AD.
Prove that in an isosceles triangle any of its equal sides is greater than the straight line joining the vertex to any point on the base of the triangle.
