Advertisements
Advertisements
प्रश्न
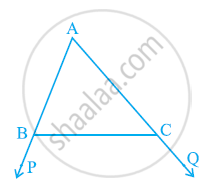
In the given figure sides AB and AC of ΔABC are extended to points P and Q respectively. Also, ∠PBC < ∠QCB. Show that AC > AB.
उत्तर
In the given figure,
∠ABC + ∠PBC = 180° (Linear pair)
⇒ ∠ABC = 180° − ∠PBC ... (1)
Also,
∠ACB + ∠QCB = 180°
∠ACB = 180° − ∠QCB … (2)
As ∠PBC < ∠QCB,
⇒ 180º − ∠PBC > 180º − ∠QCB
⇒ ∠ABC > ∠ACB [From equations (1) and (2)]
⇒ AC > AB (Side opposite to the larger angle is larger.)
APPEARS IN
संबंधित प्रश्न
In a triangle locate a point in its interior which is equidistant from all the sides of the triangle.
In the following figure, write BC, AC, and CD in ascending order of their lengths.
Name the greatest and the smallest sides in the following triangles:
ΔXYZ, ∠X = 76°, ∠Y = 84°.
Name the smallest angle in each of these triangles:
In ΔABC, AB = 6.2cm, BC = 5.6cm and AC = 4.2cm
In a triangle ABC, BC = AC and ∠ A = 35°. Which is the smallest side of the triangle?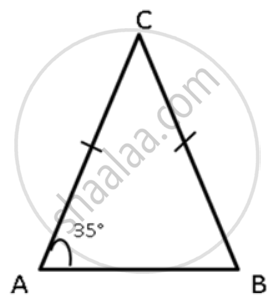
For any quadrilateral, prove that its perimeter is greater than the sum of its diagonals.
ABCD is a quadrilateral in which the diagonals AC and BD intersect at O. Prove that AB + BC + CD + AD < 2(AC + BC).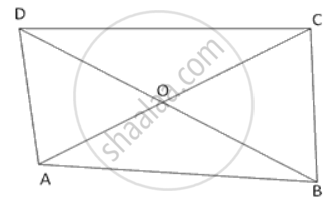
In ΔABC, BC produced to D, such that, AC = CD; ∠BAD = 125° and ∠ACD = 105°. Show that BC > CD.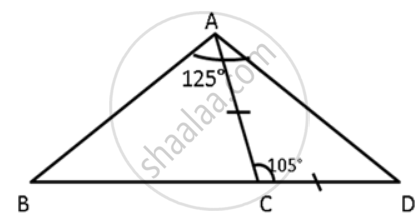
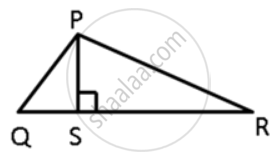
In ΔPQR, PS ⊥ QR ; prove that: PQ > QS and PQ > PS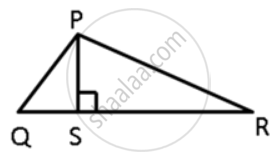
In ΔPQR, PS ⊥ QR ; prove that: PQ > QS and PR > PS