Advertisements
Advertisements
प्रश्न
Show that in a right angled triangle, the hypotenuse is the longest side.
उत्तर

Let us consider a right-angled triangle ABC, right-angled at B.
In ΔABC,
∠A + ∠B + ∠C = 180° (Angle sum property of a triangle)
∠A + 90º + ∠C = 180°
∠A + ∠C = 90°
Hence, the other two angles have to be acute (i.e., less than 90º).
∴ ∠B is the largest angle in ΔABC.
⇒ ∠B > ∠A and ∠B > ∠C
⇒ AC > BC and AC > AB
[In any triangle, the side opposite to the larger (greater) angle is longer.]
Therefore, AC is the largest side in ΔABC.
However, AC is the hypotenuse of ΔABC. Therefore, hypotenuse is the longest side in a right-angled triangle.
APPEARS IN
संबंधित प्रश्न
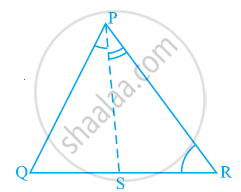
In the given figure, PR > PQ and PS bisects ∠QPR. Prove that ∠PSR >∠PSQ.
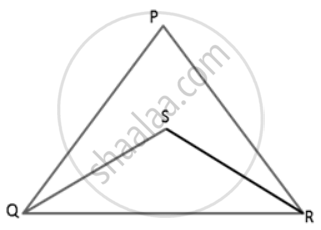
ABC is a triangle. Locate a point in the interior of ΔABC which is equidistant from all the vertices of ΔABC.
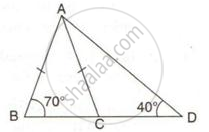
From the following figure, prove that: AB > CD.
In the following figure, ∠BAC = 60o and ∠ABC = 65o.

Prove that:
(i) CF > AF
(ii) DC > DF
For any quadrilateral, prove that its perimeter is greater than the sum of its diagonals.
In ABC, P, Q and R are points on AB, BC and AC respectively. Prove that AB + BC + AC > PQ + QR + PR.
In the given figure, ∠QPR = 50° and ∠PQR = 60°. Show that : PN < RN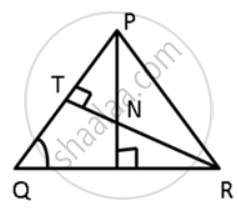
In ΔPQR, PS ⊥ QR ; prove that: PQ + PR > QR and PQ + QR >2PS.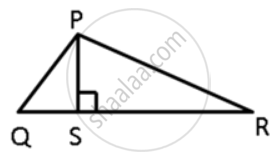
In the given figure, T is a point on the side PR of an equilateral triangle PQR. Show that PT < QT
In ΔPQR is a triangle and S is any point in its interior. Prove that SQ + SR < PQ + PR.