Advertisements
Advertisements
प्रश्न
In the given figure, ∠QPR = 50° and ∠PQR = 60°. Show that : PN < RN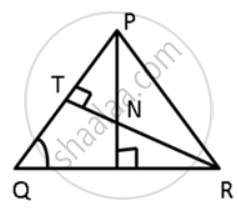
उत्तर
In the given ΔPQR,
PS < PR .....(Of all the straight lines that can be drawn to a given straight line from a point outside it, the perpendicular is the shortest)
PN < PR ....(i) (∵ PN < PS)
Also,
RT < PR .....(Of all the straight lines that can be drawn to a given straight line from a point outside it, the perpendicular is the shortest)
RN < PR .....(ii) (∵ RN < RT)
Dividing (i) bt (ii),
`"PN"/"RN" < "PR"/"PR"`
`"PN"/"RN" < 1`
PN < RN.
APPEARS IN
संबंधित प्रश्न
In the given figure, PR > PQ and PS bisects ∠QPR. Prove that ∠PSR >∠PSQ.
ABC is a triangle. Locate a point in the interior of ΔABC which is equidistant from all the vertices of ΔABC.
From the following figure, prove that: AB > CD.
Name the greatest and the smallest sides in the following triangles:
ΔDEF, ∠D = 32°, ∠E = 56° and ∠F = 92°.
Name the smallest angle in each of these triangles:
In ΔXYZ, XY = 6.2cm, XY = 6.8cm and YZ = 5cm
For any quadrilateral, prove that its perimeter is greater than the sum of its diagonals.
In ΔPQR, PR > PQ and T is a point on PR such that PT = PQ. Prove that QR > TR.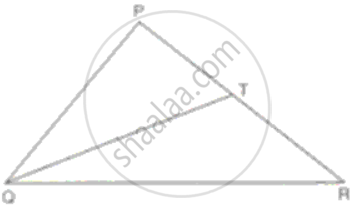
In the given figure, T is a point on the side PR of an equilateral triangle PQR. Show that PT < QT
Prove that in an isosceles triangle any of its equal sides is greater than the straight line joining the vertex to any point on the base of the triangle.
In ΔABC, D is a point in the interior of the triangle. Prove that DB + DC < AB + AC.
