Advertisements
Advertisements
प्रश्न
From the following figure, prove that: AB > CD.
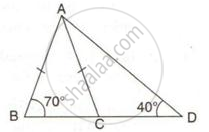
उत्तर
In ΔABC,
AB = AC ...[ Given ]
∴ ∠ACB = ∠B ...[ angles opposite to equal sides are equal ]
∠B = 70° ...[ Given ]
⇒ ∠ACB = 70° ...(i)
Now,
∠ACB +∠ACD = 180°...[ BCD is a straight line]
⇒ 70° + ∠ACD = 180°
⇒ ∠ACD = 110° ...(ii)
In ΔACD,
∠CAD + ∠ACD + ∠D = 180°
⇒ ∠CAD + 110° + ∠D = 180° ...[ From (ii) ]
⇒ ∠CAD + ∠D = 70°
But ∠D = 40° ...[ Given ]
⇒ ∠CAD + 40°= 70°
⇒ ∠CAD = 30° …(iii)
In ΔACD,
∠ACD = 110° ...[ From (ii) ]
∠CAD = 30° ...[ From (iii) ]
∠D = 40° ...[ Given ]
∴ D > ∠CAD
⇒ AC > CD ....[Greater angle has greater side opposite to it]
Also,
AB = AC ...[ Given ]
Therefore, AB > CD.
APPEARS IN
संबंधित प्रश्न
In the following figure, write BC, AC, and CD in ascending order of their lengths.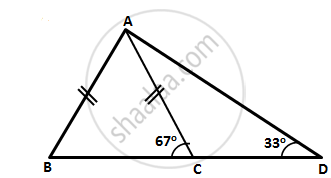
Name the greatest and the smallest sides in the following triangles:
ΔXYZ, ∠X = 76°, ∠Y = 84°.
Name the smallest angle in each of these triangles:
In ΔABC, AB = 6.2cm, BC = 5.6cm and AC = 4.2cm
Prove that the perimeter of a triangle is greater than the sum of its three medians.
D is a point on the side of the BC of ΔABC. Prove that the perimeter of ΔABC is greater than twice of AD.
ABCD is a trapezium. Prove that: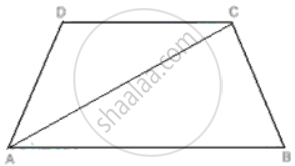
CD + DA + AB > BC.
In ΔPQR, PS ⊥ QR ; prove that: PQ + PR > QR and PQ + QR >2PS.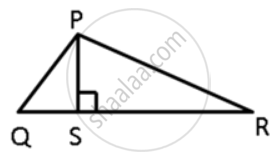
In ΔPQR is a triangle and S is any point in its interior. Prove that SQ + SR < PQ + PR.
Prove that in an isosceles triangle any of its equal sides is greater than the straight line joining the vertex to any point on the base of the triangle.
In ΔABC, D is a point in the interior of the triangle. Prove that DB + DC < AB + AC.
